The density of water at the surface of ocean is ρ . If the bulk modulus of water is B, then the density of ocean water at depth, when the pressure at a depth is αp0 and p0 is the atmospheric pressure is
ρKk−nP0
ρKK+nP0
ρKK−(n−1)P0
ρK(K+(n−1)P0)
The correct Answer is:C
To find the density of ocean water at a certain depth where the pressure is given, we can follow these steps:
1. Identify Given Values:
- Density of water at the surface: ρ
- Bulk modulus of water: B
- Pressure at depth: αP0 (where P0 is atmospheric pressure)
2. Understand the Relationship:
- The bulk modulus B is defined as:
B=−VdPdV
- This means that an increase in pressure causes a decrease in volume, which in turn increases density.
3. Relate Initial and Final States:
- Let V1 be the initial volume and V2 be the final volume at depth.
- The initial density ρ1 is ρ and the final density ρ2 can be expressed as:
ρ2=ρ1V1V2
4. Calculate Change in Pressure:
- The change in pressure ΔP when going from the surface to depth is:
ΔP=αP0−P0=(α−1)P0
5. Express Change in Volume:
- Using the definition of bulk modulus:
B=V1(α−1)P0ΔV
- Rearranging gives:
ΔV=V1(α−1)P0B
6. Relate Final Volume to Initial Volume:
- The final volume V2 can be expressed as:
V2=V1−ΔV=V1−V1(α−1)P0B
- Simplifying gives:
V2=V1(1−(α−1)P0B)
7. Substitute Back to Find Density:
- Now substituting V2 back into the density equation:
ρ2=ρV1V1(1−(α−1)P0B)=ρ1−(α−1)P0B
8. Final Expression:
- Therefore, the density of ocean water at depth is:
ρ2=ρBB−(α−1)P0
- Density of water at the surface: ρ
- Bulk modulus of water: B
- Pressure at depth: αP0 (where P0 is atmospheric pressure)
- The bulk modulus B is defined as:
B=−VdPdV
- This means that an increase in pressure causes a decrease in volume, which in turn increases density.
- Let V1 be the initial volume and V2 be the final volume at depth.
- The initial density ρ1 is ρ and the final density ρ2 can be expressed as:
ρ2=ρ1V1V2
- The change in pressure ΔP when going from the surface to depth is:
ΔP=αP0−P0=(α−1)P0
- Using the definition of bulk modulus:
B=V1(α−1)P0ΔV
- Rearranging gives:
ΔV=V1(α−1)P0B
- The final volume V2 can be expressed as:
V2=V1−ΔV=V1−V1(α−1)P0B
- Simplifying gives:
V2=V1(1−(α−1)P0B)
- Now substituting V2 back into the density equation:
ρ2=ρV1V1(1−(α−1)P0B)=ρ1−(α−1)P0B
- Therefore, the density of ocean water at depth is:
ρ2=ρBB−(α−1)P0
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBook:NCERT FINGERTPS ENGLISHChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:APPLICATIONS OF ELASTIC BEHAVIOUR OF MATERIALS
Explore 8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NCERT FINGERTPS ENGLISHChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:HOTS
Explore 8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBook:NCERT FINGERTPS ENGLISHChapter:MECHANICAL PROPERTIES OF SOLIDSExercise:STRESS-STRAIN CURVE
Explore 5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:NCERT FINGERTPS ENGLISHChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT Exemplar
Explore 5 Videos
- MOTION IN A PLANE Book:NCERT FINGERTPS ENGLISHChapter:MOTION IN A PLANE Exercise:Assertion And Reason
Explore 15 Videos
Similar Questions
The mean density of sea water is ρ, and bulk modulus is B. The change in density of sea water in going from the surface of water to a depth of h is
View SolutionA material has normal density ρ and bulk modulus K. The increase in the density of the material when it is subjected to an external pressure P from all sides is
View Solution
Knowledge Check
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depthThe normal density of gold is ρ and its bulk modulus is K. The increase is density of a lump of gold when a pressure P is applied uniformly on all sides is
Aρ P/KBρ K/PCP/ρ KDK/ρPThe pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρ
When unit mass of water boils to become steam at 100∘C, it absorbs Q amount of heat. The densities of water and steam at 100∘C are ρ1 and ρ2 respectively and the atmospheric pressure is P0. The increase in internal energy of the water is
View SolutionIf P is the pressure and ρ is the density of a gas, then P and ρ are related as :
View SolutionThe bulk modulus of water is 2.1×109Nm−2. The pressure required ot increase the density of water by 0.1% is
View Solution
Water is filled in a capillary tube of radius R. If the surface of water is hemispherical (θ=0), then find pressure at a point 'A' which is at h depth below the surface.
View SolutionA metal sphere connected by a string is dipped in a liquid of density ρ as shown in figure. The pressure at the bottom of the vessel will be, (p0=atmospheric pressure )
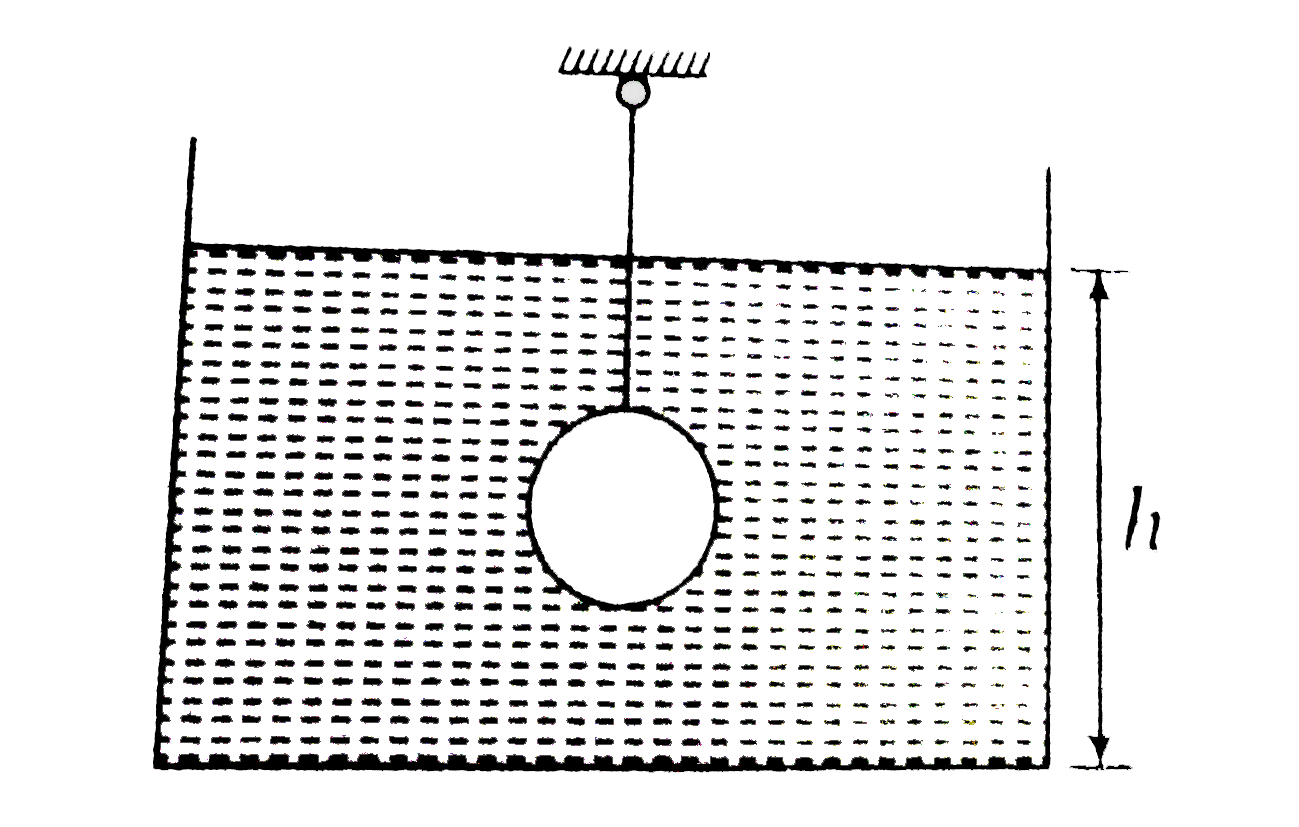
View Solution