At what angle of incidence will the light reflected from glass(μ=1.5) be completely polarised
72.8∘
51.6∘
40.3∘
56.3∘
The correct Answer is:D
To find the angle of incidence at which light reflected from glass (with a refractive index μ=1.5) is completely polarized, we can use Brewster's Law. Here’s the step-by-step solution:
Step 1: Understand Brewster's Law
Brewster's Law states that the angle of incidence at which light is completely polarized upon reflection is given by the formula:
tan(θP)=n2n1
where:
- θP is the polarizing angle,
- n2 is the refractive index of the medium into which the light is entering (in this case, glass),
- n1 is the refractive index of the medium from which the light is coming (in this case, air, which has an index of approximately 1).
Step 2: Identify the Refractive Indices
From the problem, we know:
- n2=1.5 (for glass),
- n1=1.0 (for air).
Step 3: Substitute the Values into Brewster's Law
Now, we substitute the values into the equation:
tan(θP)=1.51.0=1.5
Step 4: Calculate the Polarizing Angle
To find θP, we take the arctangent (inverse tangent) of 1.5:
θP=tan−1(1.5)
Step 5: Use a Calculator to Find the Angle
Using a calculator, we find:
θP≈56.31∘
Conclusion
The angle of incidence at which the light reflected from glass will be completely polarized is approximately:
θP≈56.3∘
Brewster's Law states that the angle of incidence at which light is completely polarized upon reflection is given by the formula:
tan(θP)=n2n1
where:
- θP is the polarizing angle,
- n2 is the refractive index of the medium into which the light is entering (in this case, glass),
- n1 is the refractive index of the medium from which the light is coming (in this case, air, which has an index of approximately 1).
From the problem, we know:
- n2=1.5 (for glass),
- n1=1.0 (for air).
Now, we substitute the values into the equation:
tan(θP)=1.51.0=1.5
To find θP, we take the arctangent (inverse tangent) of 1.5:
θP=tan−1(1.5)
Using a calculator, we find:
θP≈56.31∘
The angle of incidence at which the light reflected from glass will be completely polarized is approximately:
θP≈56.3∘
Topper's Solved these Questions
- WAVE OPTICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - HIGHER ORDER THINKING SKILLS
8 Videos
- WAVE OPTICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - NCERT EXEMPLAR PROBLEMS
5 Videos
- SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS EXERCISE - Assertion And Reason
15 Videos
Similar Questions
When light is incident from air to glass at an angle 57∘, the reflected beam is completely polarised. If the same beam is incident from water to glass, the angle of incidence at which reflected beam is completely polarised will be
View SolutionA beam of light strikes a surface at angle of incidence of 60∘ and reflected beam becomes completely polarised . The refractive index of glass surface is -
View SolutionWhen the angle of incidence on a material is 60∘, the reflected light is completely polarized. The velocity of the refracted ray inside the material is (in ms−1)
View SolutionWhen the angle of incidence on a material is 60∘, the reflected light is completely polarized. The velocity of the refracted ray inside the material is (in ms−1)
View SolutionWhen the angle of incidence on a material is 60∘, the reflected light is completely polarized. The velocity of the refracted ray inside the material is (in ms−1)
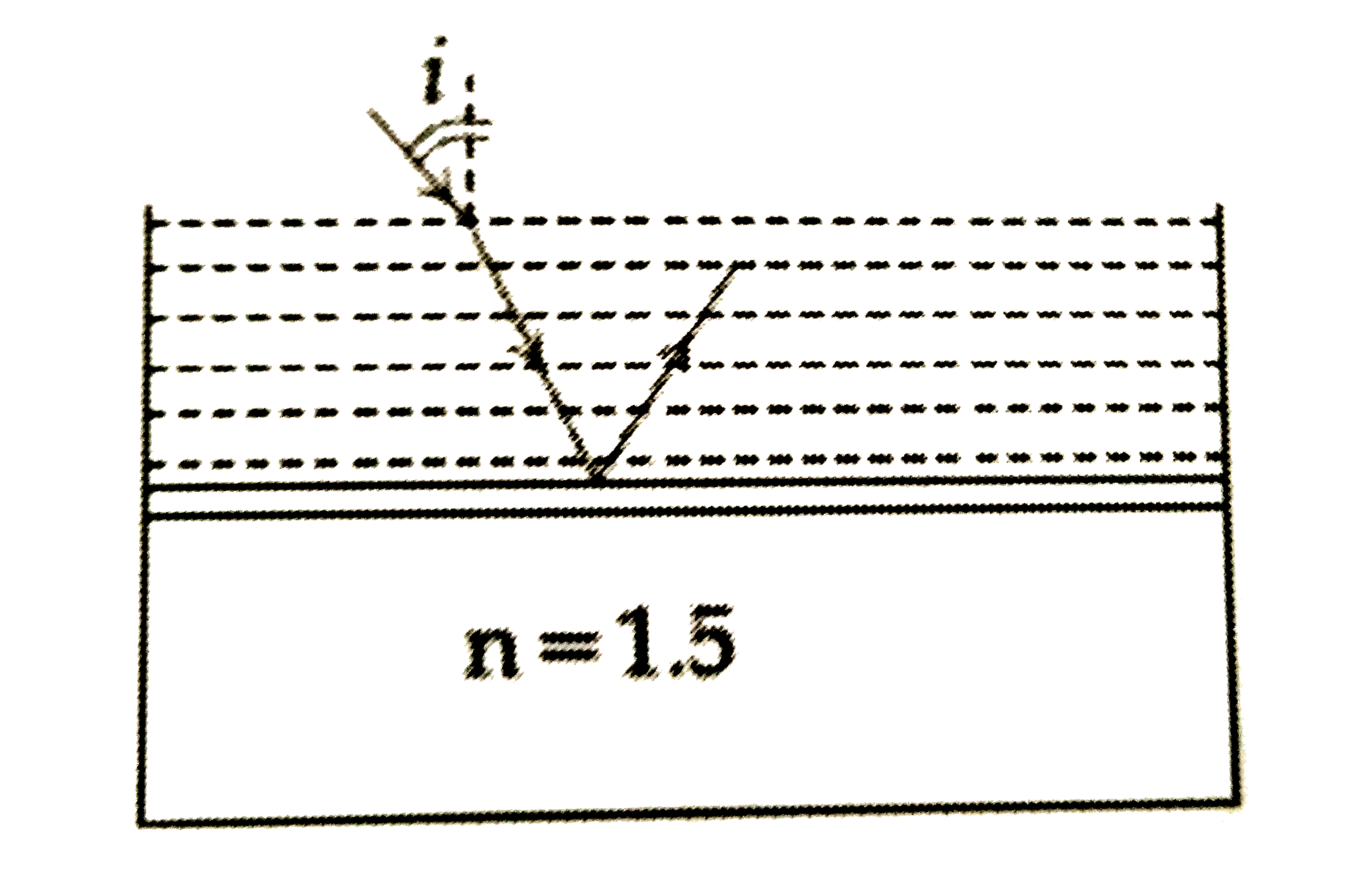
View SolutionConsider a tank made of glass (refractive index 1.5) with a thick bottom. It is filled with a liquid of refractive index μ. A student finds that, irrespective of what the incident angle I (see figure) is for a beam of light entering the liquid, the light reflected from the liquid glass interface is never completely polarized. For this to happen, the minimum value of μ is :
View SolutionA beam of light travelling in water strikes a glass plate, which is also immersed in water. When the angle of incidence is 51∘, the reflected beam is found to be plane polarised . What is the refractive index of glass if the refractive index of water is 43? [tan51∘=1.235]
View SolutionFind the maximum angle of refraction when a light ray is refracted from glass (μ=1.50) to air.
View SolutionA container made of glass (μ=1.5) contains a liquid. A ray of light passing through the liquid falls on the bottom of the container at an angle of incidence θ=tan−1(0.9) and completely polarized. The ray should strike the bottom of the container at an angle of incidences so that it undergoes total internal reflection
View SolutionUnpolarized light is incident on a plane glass surface having refractive index . The angle of incidence at which reflected and refracted rays would become perpendicular to each other is :
View Solution
