Two resistances are connected in the two gaps of a meter bridge. The balance point is from the zero end. When a resistance is connected in series with the smaller of two resistance, the null point+ shifts to . The smaller of the two resistance has the value.
3
6
9
12
The correct Answer is:C
To solve the problem step by step, we will use the principles of a meter bridge and the balance condition.
1. Understanding the Meter Bridge Principle:
In a meter bridge, when two resistances R and S are connected, the balance point L is given by the formula:
R=S⋅L100−L
where L is the distance from the zero end to the balance point.
2. First Condition:
Given that the balance point is at L=20cm:
R=S⋅20100−20=S⋅2080=S4
This gives us our first equation:
R=S4(Equation 1)
3. Second Condition:
When a resistance of 15Ω is connected in series with the smaller resistance R, the new balance point shifts to L′=40cm:
R′=R+15
The new balance condition can be expressed as:
R′=S⋅L′100−L′=S⋅40100−40=S⋅4060=2S3
This gives us our second equation:
R+15=2S3(Equation 2)
4. Substituting Equation 1 into Equation 2:
From Equation 1, we have S=4R. Substituting this into Equation 2:
R+15=2(4R)3
Simplifying this:
R+15=8R3
5. Clearing the Fraction:
Multiply through by 3 to eliminate the fraction:
3R+45=8R
6. Rearranging the Equation:
Rearranging gives:
45=8R−3R
45=5R
7. Solving for R:
Dividing both sides by 5:
R=9Ω
8. Conclusion:
The smaller of the two resistances R has a value of 9Ω.
Final Answer:
The smaller of the two resistances has the value 9Ω.
In a meter bridge, when two resistances R and S are connected, the balance point L is given by the formula:
R=S⋅L100−L
where L is the distance from the zero end to the balance point.
Given that the balance point is at L=20cm:
R=S⋅20100−20=S⋅2080=S4
This gives us our first equation:
R=S4(Equation 1)
When a resistance of 15Ω is connected in series with the smaller resistance R, the new balance point shifts to L′=40cm:
R′=R+15
The new balance condition can be expressed as:
R′=S⋅L′100−L′=S⋅40100−40=S⋅4060=2S3
This gives us our second equation:
R+15=2S3(Equation 2)
From Equation 1, we have S=4R. Substituting this into Equation 2:
R+15=2(4R)3
Simplifying this:
R+15=8R3
Multiply through by 3 to eliminate the fraction:
3R+45=8R
Rearranging gives:
45=8R−3R
45=5R
Dividing both sides by 5:
R=9Ω
The smaller of the two resistances R has a value of 9Ω.
The smaller of the two resistances has the value 9Ω.
Topper's Solved these Questions
- PRACTICE PAPPERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Papper 3
50 Videos
- PRACTICE PAPPERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 1
50 Videos
- PRACTICE PAPPERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Paper 3
50 Videos
- NUCLEIBOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - Assertion And Reason
15 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT Exemplar
11 Videos
Similar Questions
Two resistances are connected in two gaps of a meter bridge. The balance point is 20cm from the zero end. A resistance of 15Ω is connected in series with the smaller of the two. The null point shifts to 40cm. The value of the smaller resistance in Ω is
View SolutionTwo unknown resistances are connected in two gaps of a meter-bridge. The null point is obtained at 40 cm from left end. A 30Ω resistance is connected in series with the smaller of the two resistances, the null point shifts by 20 cm to the right end. The value of smaller resistance in Ω is
View SolutionWith two resistance R1andR2(>R1) in the two gaps of a metre bridge the balance was found to be 1/3 m from the zero end. When a 6Ωresistance is connected in series with the smaller of the two resistance, the point is shifted to 2/3 m from the same end, then R1andR2 are
View SolutionThe figure shows experimental set up of a meter bridge. When the two unknown resistances X and Y are inserted, the null point D is obtained 40 cm from the end A. When a resistance of 10Ω is connected in series with X, the null point shifts by 10 cm. Find the position of the null point when the 10Ω resistance is instead connected in series with resistance 'Y'. Determine the value of the resistance X and Y
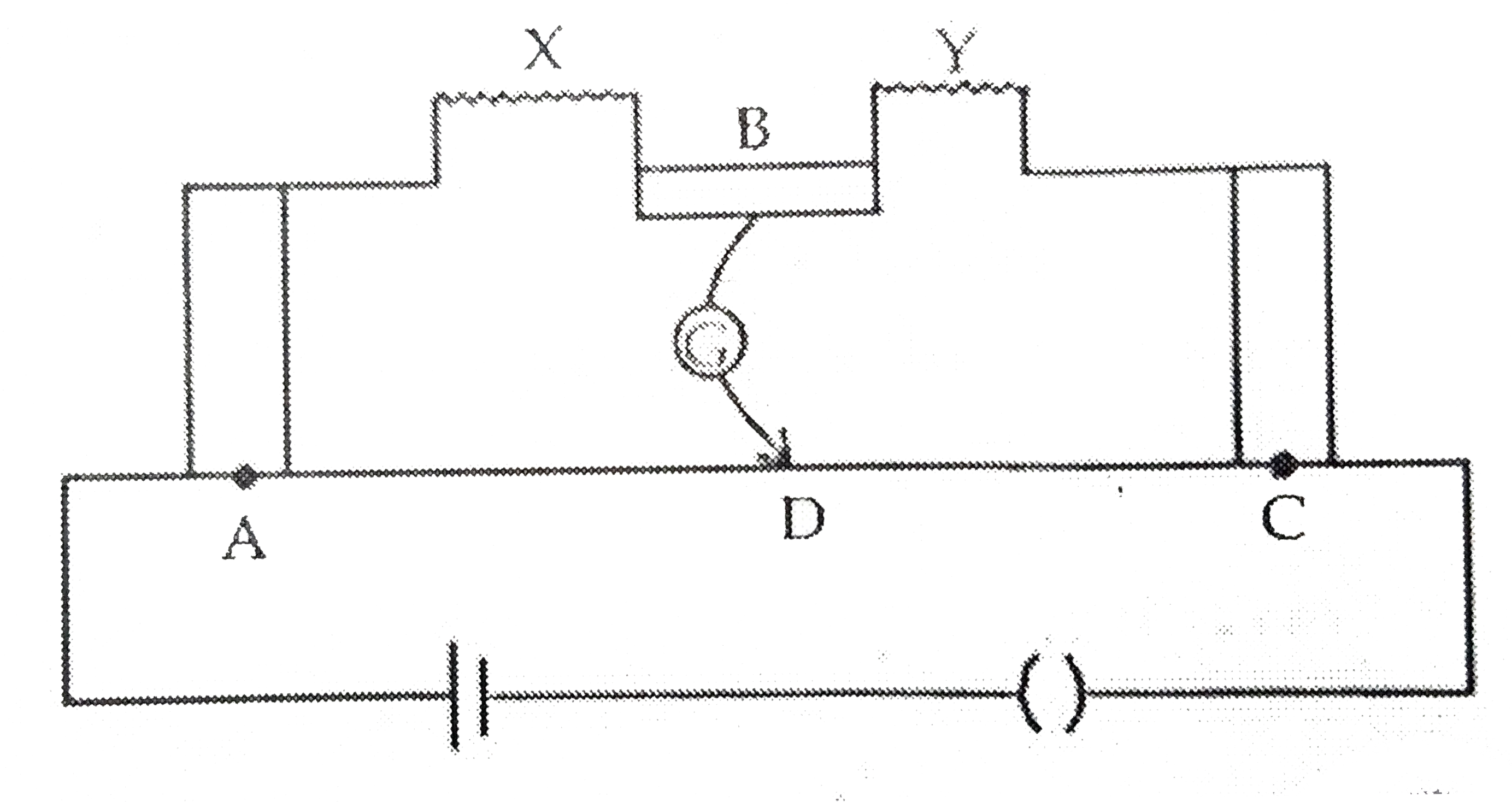
View SolutionTwo unkonwn resistances X and Y are connected to left and right gaps of a meter bridge and the balancing point is obtained at 80cm from left. When a 10Ω resistance is connected parallel to X, the balancing point is 50cm from left. The values of X and Y respectively are
View SolutionThe figure
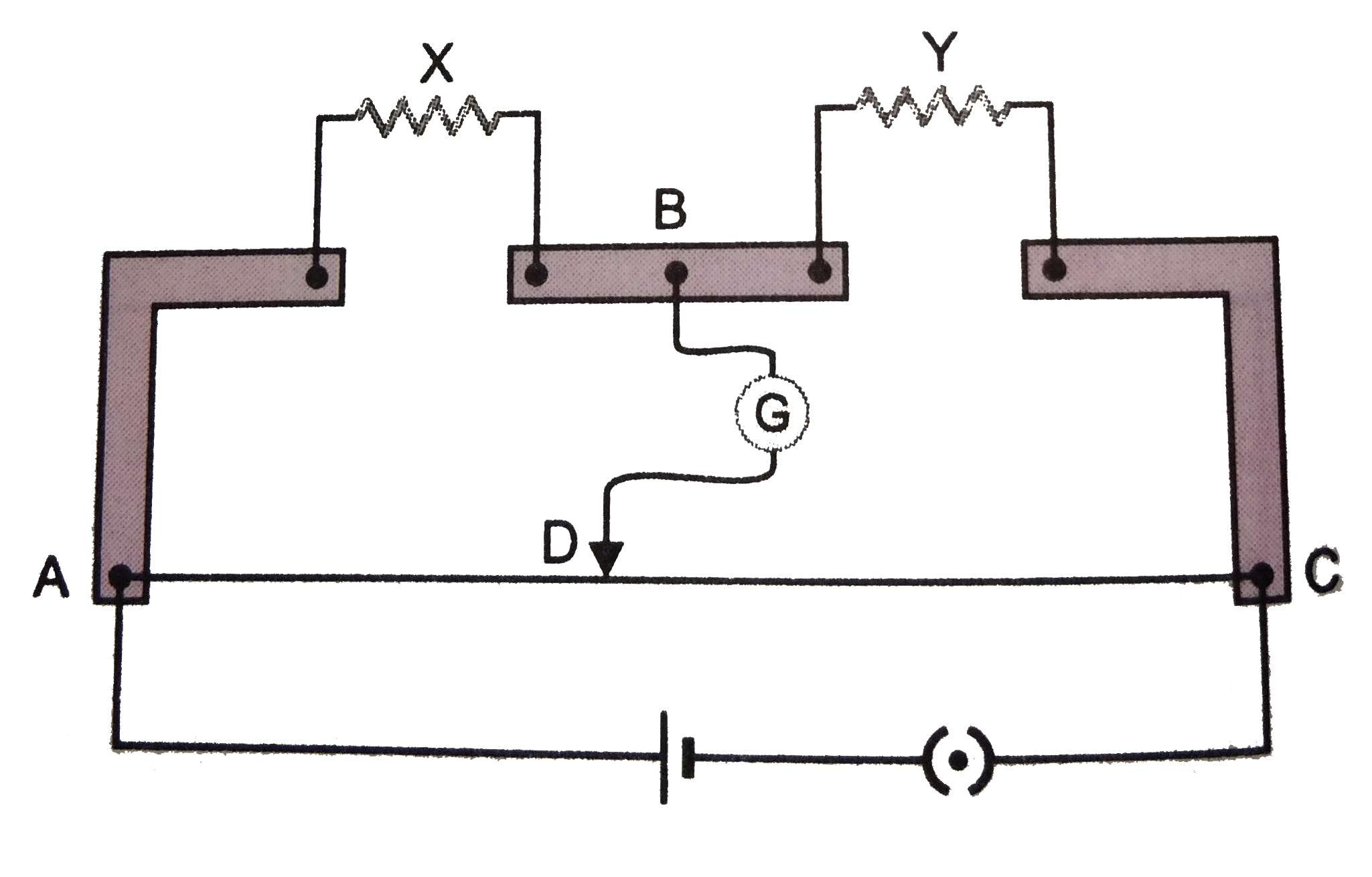
Shows the experimental set up of a meter bridge. The null point is found to be 60 cm away from the end A with X and Y in positions as shown. When a resistance of 15Ω is connected in series with Y, the null points is found to shift by 10 cm towards the end A of the wire. Find the position of the null point if resistance of 30Ω were connected in parallel with Y.
View SolutionThe resistance in the left and right gaps of a balanced meter bridge are R1 and R1. The balanced point is 50cm. If a resistance of 24Ω is connected in parallel to R2, the balance point is 70cm. The value of R1 or R2 is.
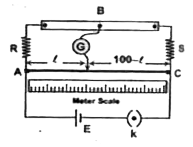
View SolutionIn a meter bridge, the null point is found at a distance of 40 cm from A. If now a resistance of 12 Ω is connected in parallel with S, the null point occurs at 60 cm from A. Determine the value of R.
View SolutionIn a meter bridge,
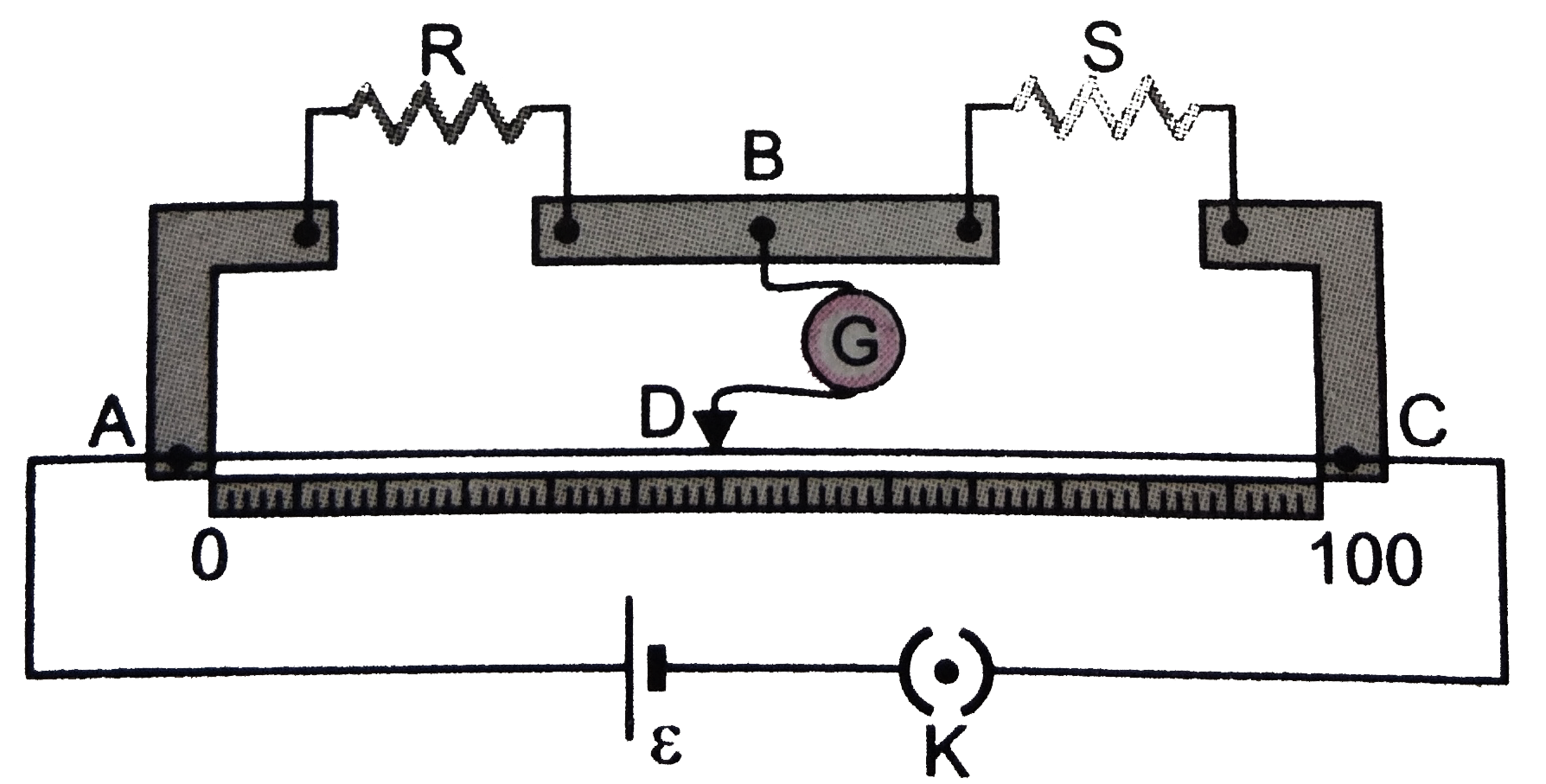
the null points is found at a distance of 33.7 cm from A. If now a resistance of 12Ω is connected in parallel with S, the null point occurs at 51.9 cm. Determine the values of R and S.
View SolutionWhen a resistance of 100Ω is connected in series with a galvanometer of resistance R, then its range is V. To double its range, a resistance of 1000Ω is connected in series. Find the value of R.
View Solution
