The circular plates A and B of a parallel plate air capacitor have a diameter of 0.1 m and are 2×10−3m apart. The plates C and D of a similar capacitor have a diameter of 0.12m and are 3×10−3m apart. Plate A is earthed. Plates B and D are connected together. Plate C is connected to the positive pole of a 120V battery whose negative is earthed. The energy stored in the system is
0.1224μJ
0.2224μJ
0.3224μJ
0.4224μJ
The correct Answer is:A
To solve the problem, we need to find the energy stored in the system of capacitors. We have two parallel plate capacitors, and we will calculate the capacitance for each and then find the total energy stored.
Step 1: Calculate the Capacitance of Capacitor A-B (C1)
The formula for the capacitance C of a parallel plate capacitor is given by:
C=ϵ0Ad
Where:
- ϵ0 is the permittivity of free space, approximately 8.85×10−12F/m
- A is the area of one of the plates
- d is the separation between the plates
The diameter of plates A and B is 0.1m, so the radius r1 is:
r1=0.12=0.05m
The area A1 is:
A1=πr21=π(0.05)2=π(0.0025)≈0.00785m2
The separation d1 is 2×10−3m.
Now substituting the values into the capacitance formula:
C1=ϵ0A1d1=8.85×10−12×0.007852×10−3≈3.47×10−10F
Step 2: Calculate the Capacitance of Capacitor C-D (C2)
For plates C and D, the diameter is 0.12m, so the radius r2 is:
r2=0.122=0.06m
The area A2 is:
A2=πr22=π(0.06)2=π(0.0036)≈0.0113m2
The separation d2 is 3×10−3m.
Now substituting the values into the capacitance formula:
C2=ϵ0A2d2=8.85×10−12×0.01133×10−3≈3.34×10−10F
Step 3: Calculate the Effective Capacitance (C)
Since capacitors C1 and C2 are connected in series, the effective capacitance C is given by:
1C=1C1+1C2
Substituting the values we calculated:
1C=13.47×10−10+13.34×10−10
Calculating this gives:
1C≈2.88×109F−1
Thus,
C≈12.88×109≈3.47×10−10F
Step 4: Calculate the Energy Stored in the Capacitors
The energy E stored in a capacitor is given by:
E=12CV2
Where V is the voltage across the capacitor. Here, V=120V.
Substituting the values:
E=12×3.47×10−10×(120)2
Calculating this gives:
E≈12×3.47×10−10×14400≈2.5×10−6J=0.25microjoules
Final Answer
The energy stored in the system is approximately 0.25microjoules.
---
- ϵ0 is the permittivity of free space, approximately 8.85×10−12F/m
- A is the area of one of the plates
- d is the separation between the plates
Topper's Solved these Questions
- PRACTICE PAPPERBook:NCERT FINGERTPS ENGLISHChapter:PRACTICE PAPPERExercise:Practice Paper 1
Explore 50 Videos
- PRACTICE PAPPERBook:NCERT FINGERTPS ENGLISHChapter:PRACTICE PAPPERExercise:Practice Paper 2
Explore 50 Videos
- PRACTICE PAPPERBook:NCERT FINGERTPS ENGLISHChapter:PRACTICE PAPPERExercise:Practice Papper 2
Explore 50 Videos
- NUCLEIBook:NCERT FINGERTPS ENGLISHChapter:NUCLEIExercise:Assertion And Reason
Explore 15 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:NCERT FINGERTPS ENGLISHChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:NCERT Exemplar
Explore 11 Videos
Similar Questions
Knowledge Check
A copper plate of thickness b is placed inside a parallel plate capacitor of plate distance d and area A as shown in figure. The capacitance of capacitor is
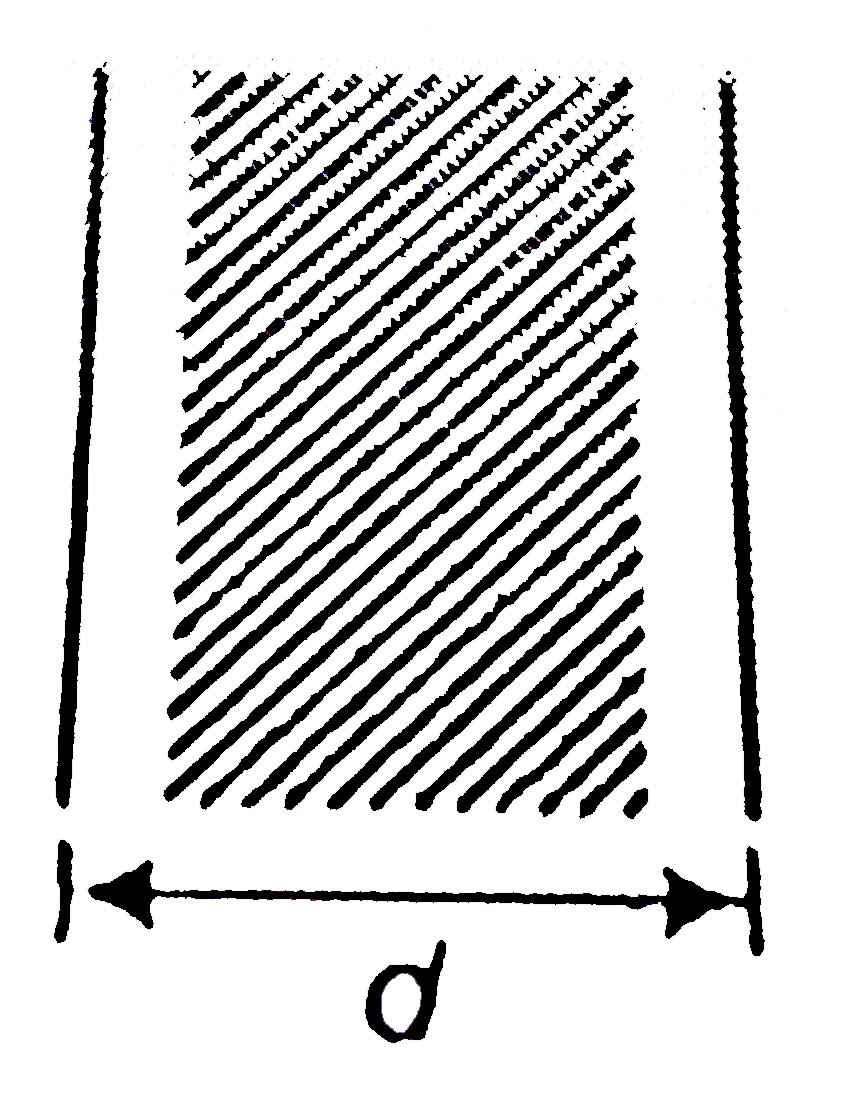 Aε0Ad+b2Bε0A2dCε0Ad−bD2ε0Ad+b2
Aε0Ad+b2Bε0A2dCε0Ad−bD2ε0Ad+b2
Each plate of a parallel plate capacitor has a charge q on it. The capacitor is now connected to a battery. Now,
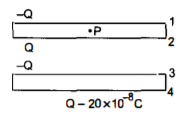
View SolutionThe nagative plate of a parallel plate capacitor is given a charge of −20X10−8C. Find the charges appearing on the four surface of the capacitor plates.
View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionThe distance between the circular plates of a parallel plate condenser 40 mm in diameter, in order to have same capacity as a sphere of radius 1 m is
View SolutionA parallel plate capacitor has an electric field of 105V/m between the plates. If the charge on the capacitor plate is 1μC, then force on each capacitor plate is-
View SolutionThe plates of a paraller-plate capacitor are made of circular discs of radii5.0cm each .If the separation between the plates is 1.0mm,What is the capacitance?
View Solution