A cube of side x has a charge q at each of its vertices. Determine the potential due to this charge array at the center of the cube.
4q3πε0x
4q√3πε0x
3q4πε0x
2q√3πε0x
The correct Answer is:B
To determine the electric potential at the center of a cube with a charge q at each of its vertices, we can follow these steps:
Step 1: Understand the Geometry of the Cube
A cube has 8 vertices, and we need to find the potential at the center of the cube due to the charges at these vertices.
Step 2: Calculate the Distance from the Center to a Vertex
The distance from the center of the cube to any vertex can be calculated using the formula for the diagonal of the cube. The length of the diagonal d of a cube with side length x is given by:
d=√x2+x2+x2=√3x2=x√3
Since we want the distance from the center to a vertex, we take half of this diagonal:
r=d2=x√32
Step 3: Formula for Electric Potential
The electric potential V due to a point charge q at a distance r is given by:
V=kqr
where k=14πϵ0.
Step 4: Calculate the Total Potential at the Center
Since there are 8 charges at the vertices, the total potential Vtotal at the center of the cube is the sum of the potentials due to each charge:
Vtotal=8×V=8×kqr
Substituting for r:
Vtotal=8×kqx√32=8×k2qx√3=16kqx√3
Step 5: Substitute the Value of k
Now substituting k=14πϵ0:
Vtotal=16⋅14πϵ0⋅qx√3=4qπϵ0x√3
Final Answer
Thus, the potential at the center of the cube due to the charges at its vertices is:
V=4qπϵ0x√3
A cube has 8 vertices, and we need to find the potential at the center of the cube due to the charges at these vertices.
The distance from the center of the cube to any vertex can be calculated using the formula for the diagonal of the cube. The length of the diagonal d of a cube with side length x is given by:
d=√x2+x2+x2=√3x2=x√3
Since we want the distance from the center to a vertex, we take half of this diagonal:
r=d2=x√32
The electric potential V due to a point charge q at a distance r is given by:
V=kqr
where k=14πϵ0.
Since there are 8 charges at the vertices, the total potential Vtotal at the center of the cube is the sum of the potentials due to each charge:
Vtotal=8×V=8×kqr
Substituting for r:
Vtotal=8×kqx√32=8×k2qx√3=16kqx√3
Now substituting k=14πϵ0:
Vtotal=16⋅14πϵ0⋅qx√3=4qπϵ0x√3
Thus, the potential at the center of the cube due to the charges at its vertices is:
V=4qπϵ0x√3
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
Similar Questions
A cube of side b has a charge q at each of its vertices. Determine the electric field due to this charge array at the center of the cube.
View SolutionA cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the center of the cube.
View Solution
Knowledge Check
A hexagon of side 8 cm has a charge 4μC at each of its vertices. The potential at the centre of the hexagon is
A2.7×106VB7.2×1011VC2.5×1012VD3.4×104V
A regular hexagon of side 10 cm has a charge 5μC at each of its vertices. Calculate the potential at the center of the hexagon.
View SolutionGiven a cube with point charges q on each of its vertices. Calculate the force exerted on any of the charges due to rest of the 7 charges.
View SolutionA cube of side 10 cm encloses a charge of 0.1μC at its center. Calculate the number of lines of force through each face of the cube.
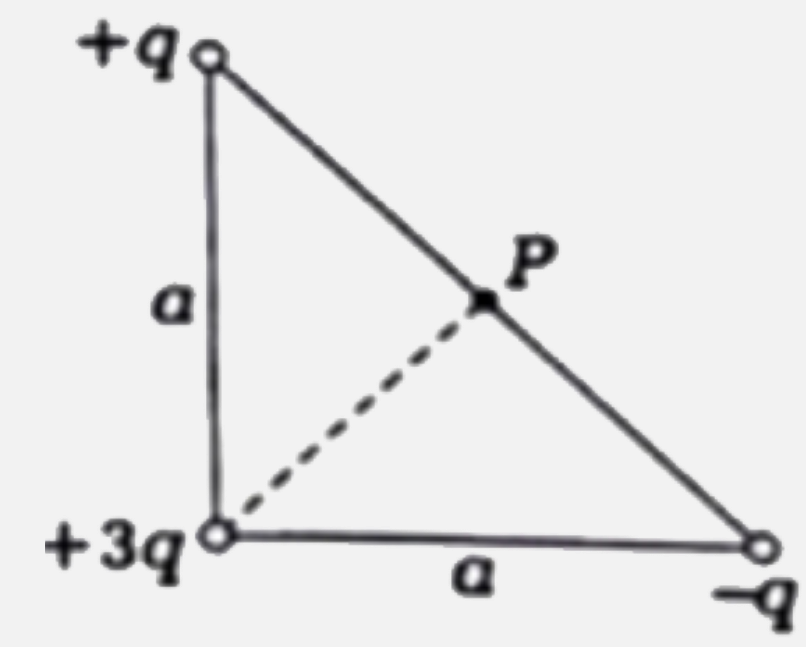
View SolutionA right isosceles triangle of side a has charges q, 3q and - q arranged on its vertices. What is the electric potential at P midway between the line connecting the + q and – q charges ?
View SolutionA charge Q is placed at each corner of a cube of side a. The potential at the centre of the cube is
View SolutionWhen identical point charges are placed at the vertices of a cube of edge length 'a' each of them experiences a net force of magnitude F.
Now these charges are placed on the vertices of another cube of edge length 'b'. What will be magnitude of the net force on any of the charges? These cubes are simply geometrical constructs and not made by any matter.
View SolutionIf identical charges (−q) are placed at each corner of a cube of side b, then electric potential energy of charge (+q) which is placed at centre of the cube will be
View Solution
