Minimum number of capacitors each of 8μF and 250 V used to make a composite capacitor of 16μF and 1000 V are
8
32
16
24
The correct Answer is:B
To solve the problem of finding the minimum number of capacitors required to create a composite capacitor of 16 µF and 1000 V using capacitors of 8 µF and 250 V, we can follow these steps:
Step 1: Determine the Voltage Requirement
We need to create a composite capacitor that can handle 1000 V. Each capacitor can handle a maximum of 250 V. To find out how many capacitors we need in series to achieve the required voltage, we can use the formula:
Number of capacitors in series=Total VoltageVoltage rating of one capacitor=1000 V250 V=4
Hint: To increase the voltage rating of capacitors, connect them in series. The total voltage rating is the sum of the individual voltage ratings.
Step 2: Determine the Capacitance Requirement
Next, we need to achieve a total capacitance of 16 µF. When capacitors are connected in series, the equivalent capacitance (C_eq) can be calculated using the formula:
1Ceq=1C1+1C2+…+1Cn
For 'n' identical capacitors of capacitance C, this simplifies to:
Ceq=Cn
In our case, we have 4 capacitors of 8 µF in series:
Ceq=8 µF4=2 µF
Hint: When capacitors are connected in series, the equivalent capacitance is less than the smallest capacitance in the series.
Step 3: Calculate the Number of Rows Needed
Now, we need to find out how many such series combinations (rows) are required to achieve a total capacitance of 16 µF. We can use the formula for total capacitance in parallel:
Ctotal=Ceq×Number of Rows
Let 'm' be the number of rows. We want:
16 µF=2 µF×m
Solving for m gives:
m=16 µF2 µF=8
Hint: To increase the total capacitance, connect the equivalent capacitors in parallel.
Step 4: Calculate the Total Number of Capacitors
Now, we know that we need 4 capacitors in each row and we have 8 rows. Therefore, the total number of capacitors required is:
Total Capacitors=Number of capacitors per row×Number of rows=4×8=32
Final Answer
The minimum number of capacitors required is 32 capacitors.
---
We need to create a composite capacitor that can handle 1000 V. Each capacitor can handle a maximum of 250 V. To find out how many capacitors we need in series to achieve the required voltage, we can use the formula:
Next, we need to achieve a total capacitance of 16 µF. When capacitors are connected in series, the equivalent capacitance (C_eq) can be calculated using the formula:
Now, we need to find out how many such series combinations (rows) are required to achieve a total capacitance of 16 µF. We can use the formula for total capacitance in parallel:
Now, we know that we need 4 capacitors in each row and we have 8 rows. Therefore, the total number of capacitors required is:
The minimum number of capacitors required is 32 capacitors.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
Similar Questions
A capacitorn of 2μF charged to 50 V is connected in parallel with another capacitor of 1μF charged to 20 V. The common potential and loss of energy will be
View SolutionA capacitor of 20μF charged upto 500 V is connected in parallel with another capacitor of 10μF, which is charged upto 200 V. the common potential is
View SolutionMinimum number of capacitors each of 8μF and 250 V used to make a composite capacitor of 16μF and 1000 V are
View SolutionA capacitor of capacitor 5.00μF is charged to24.0V and another capacitor of capacitance 6.0μF is charged to 12.0V (a) Find the energy stored in each capacitor .(b)The positive plates of the first capacitor is now connected to the negative plate of the second and vice versa . Find the new charges on the capacitors .(c)Find the loss of electrostatic energy during the process .(d)Where does this energy go?
View SolutionA capacitor 4μF charged to 50V is connected to another capacitor of 2μF charged to 100V with plates of like charges connected together. The total energy before and after connection in multiples of (10−2J) is
View SolutionA capacitor of capacitance 4μF is charged to 80V and another capacitor of capacitance 6μF is charged to 30V are connected to each other using zero resistance wires such that the positive plate of one capacitor is connected to the positive plate of the other. The energy lost by the 4μF capacitor in the process in X∗10−4J. Find the value of X.
View SolutionA capacitor of capacitance 4μF is charged to 80V and another capacitor of capacitance 6μF is charged to 30V are connected to each other using zero resistance wires such that the positive plate of one capacitor is connected to the positive plate of the other. The energy lost by the 4μF capacitor in the process in X∗10−4J. Find the value of X.
View SolutionFive identical capacitor plates are arranged such that they make four capacitors each of 2μF. The plates are connected to a source of emf 10V. The charge on plate C is
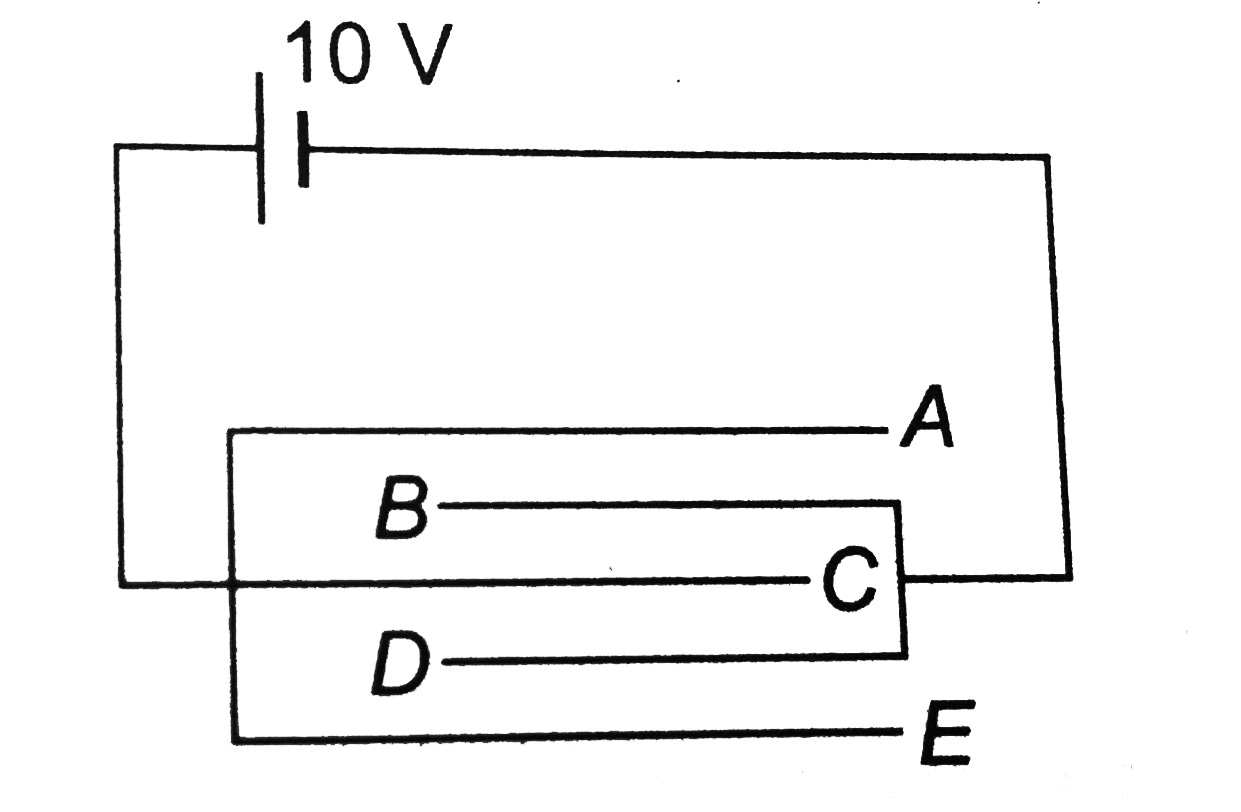
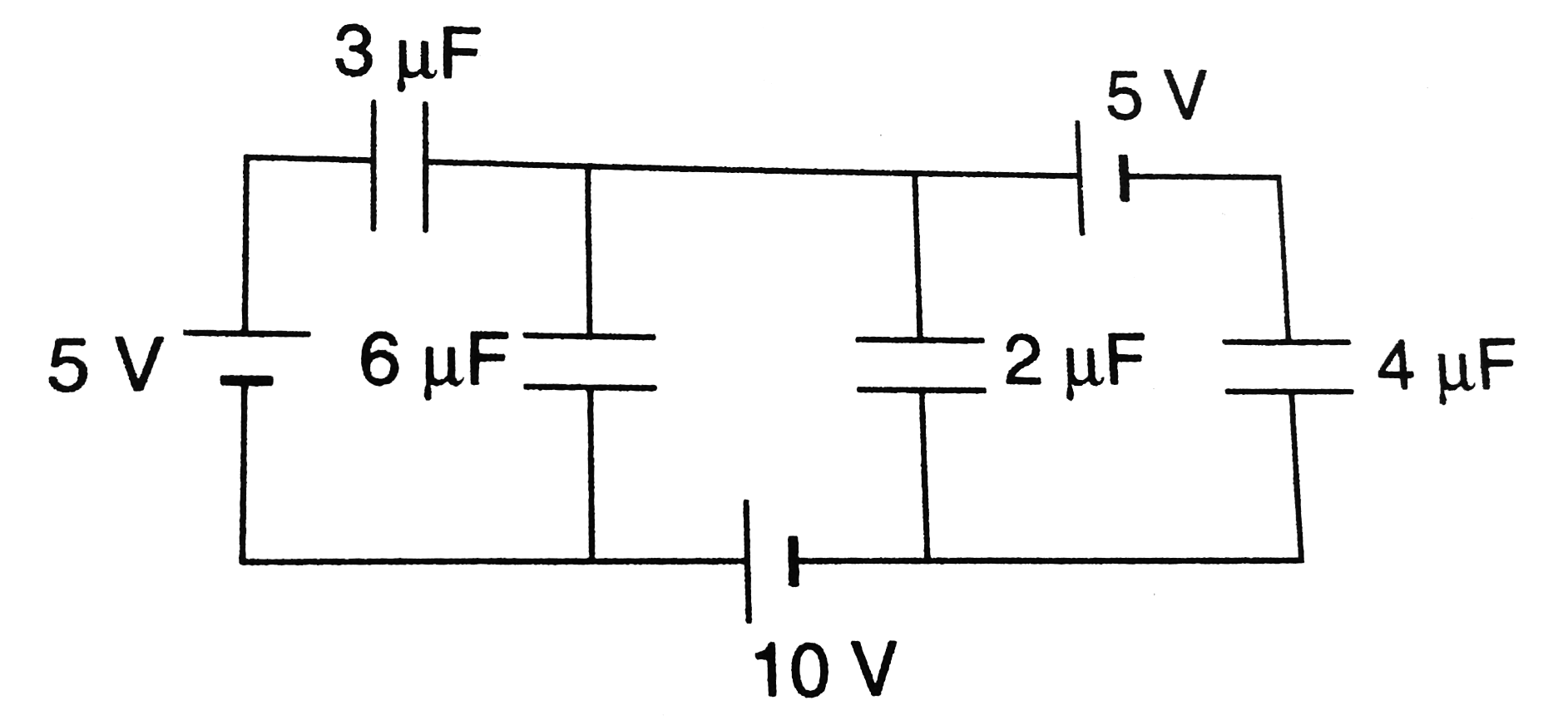
View SolutionFind the charges on 6μF and 4μF capacitors
View SolutionTwo capacitors of capacitance 2μF and 3μF are joined in series. Outer plate first capacitor is at 1000 volt and outer plate of second capacitor is earthed (grounded). Now the potential on inner plate of each capacitor will be
View Solution
