Consider a parallel plate capcaitor with plates 20 cm by 20 cm and separated by 2 mm. The dielectric constant of the material between the plates is 5. The plates are connected to a voltage source of 500 V. The energy density of the field between the plates will be close to
2.65J/m3
1.95J/m3
1.38J/m3
0.69J/m3
The correct Answer is:C
To find the energy density of the electric field between the plates of a parallel plate capacitor, we can use the formula for energy density U:
U=12ϵE2
Where:
- U is the energy density,
- ϵ is the permittivity of the dielectric material,
- E is the electric field between the plates.
Step 1: Calculate the Electric Field E
The electric field E between the plates of a capacitor is given by the formula:
E=Vd
Where:
- V is the voltage across the plates (500 V),
- d is the separation between the plates (2 mm = 2×10−3 m).
Substituting the values:
E=500V2×10−3m=5000.002=250000V/m
Step 2: Calculate the Permittivity ϵ
The permittivity ϵ of the dielectric material is given by:
ϵ=Kϵ0
Where:
- K is the dielectric constant (5),
- ϵ0 is the permittivity of free space, approximately 8.854×10−12F/m.
Substituting the values:
ϵ=5×8.854×10−12F/m=4.427×10−11F/m
Step 3: Calculate the Energy Density U
Now, substitute E and ϵ into the energy density formula:
U=12ϵE2
Substituting the values:
U=12×4.427×10−11F/m×(250000V/m)2
Calculating (250000)2:
(250000)2=62500000000(V/m)2
Now substituting this value into the equation for U:
U=12×4.427×10−11×62500000000
Calculating:
U=12×4.427×10−11×6.25×1010
U=12×2.7671875≈1.38359375J/m3
Rounding off, we get:
U≈1.38J/m3
Final Answer
The energy density of the field between the plates will be close to 1.38 J/m³.
---
- U is the energy density,
- ϵ is the permittivity of the dielectric material,
- E is the electric field between the plates.
- V is the voltage across the plates (500 V),
- d is the separation between the plates (2 mm = 2×10−3 m).
- K is the dielectric constant (5),
- ϵ0 is the permittivity of free space, approximately 8.854×10−12F/m.
U=12×2.7671875≈1.38359375J/m3
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS5videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS3videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason15videos
ELECTROMAGNETIC WAVES
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar5videos
MAGNETISM AND MATTER
Similar Questions
Consider the diagram,

A parallel plate capacitor has the plate width t and length L while the separation between the plates is d. The capacitor is connected to a battery of voltage rating V. A dielectric which carefully occupy, the space between the plates of the capacitor is slowly inserted between the plates. when length x of the dielectric slab is introduced into the capacitor, then energy stored in the system isView SolutionA parallel plate capacitor is made of two circular plates separated by a distance 5mm and with a dielectric of dielectric constant 2.2 between them. When the electric field in the dielectric is 3×104V/m the charge density of the positive plate will be close to:
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
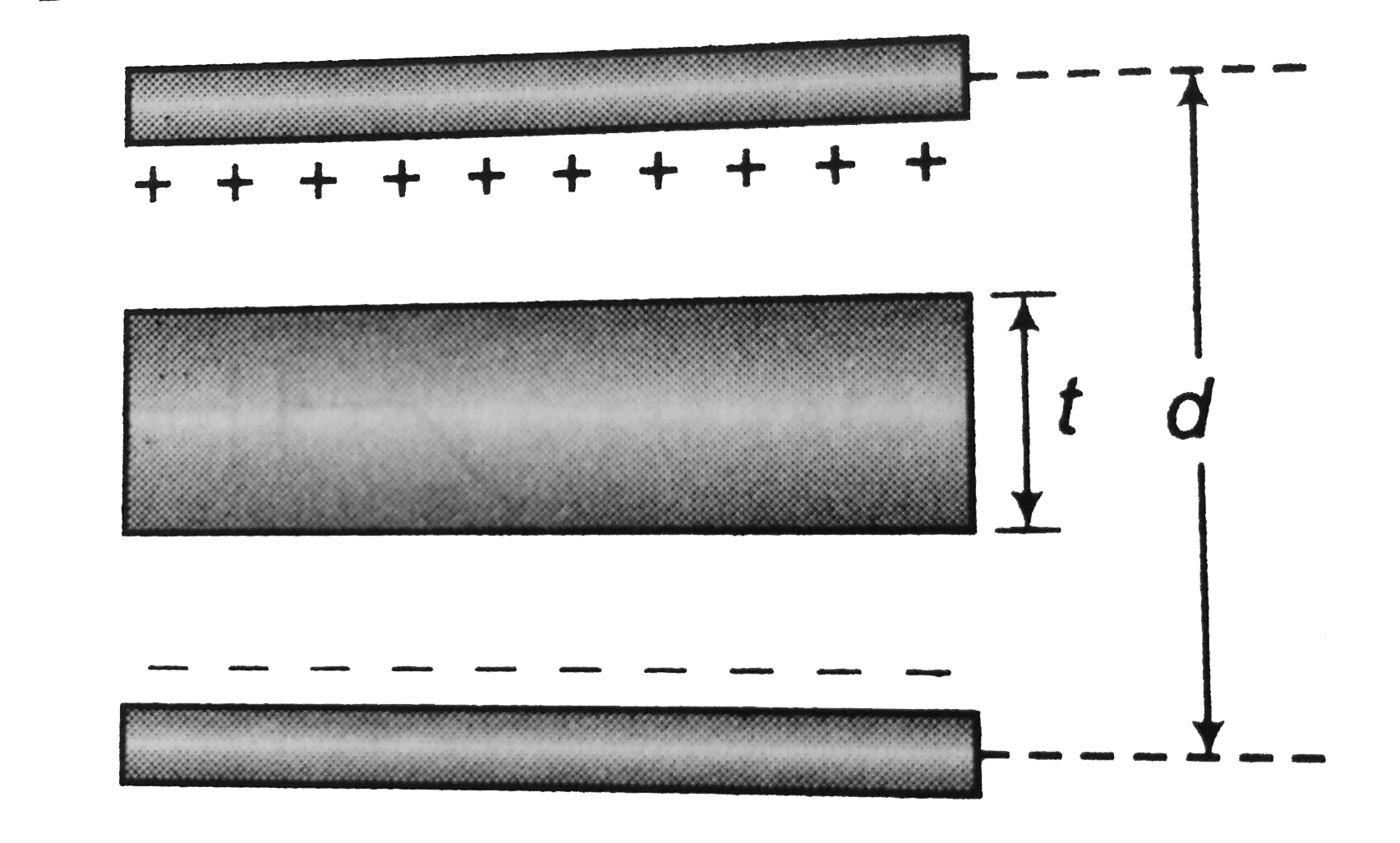
The electric field in the gaps between the plates and the electric slab will beView SolutionFigue shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
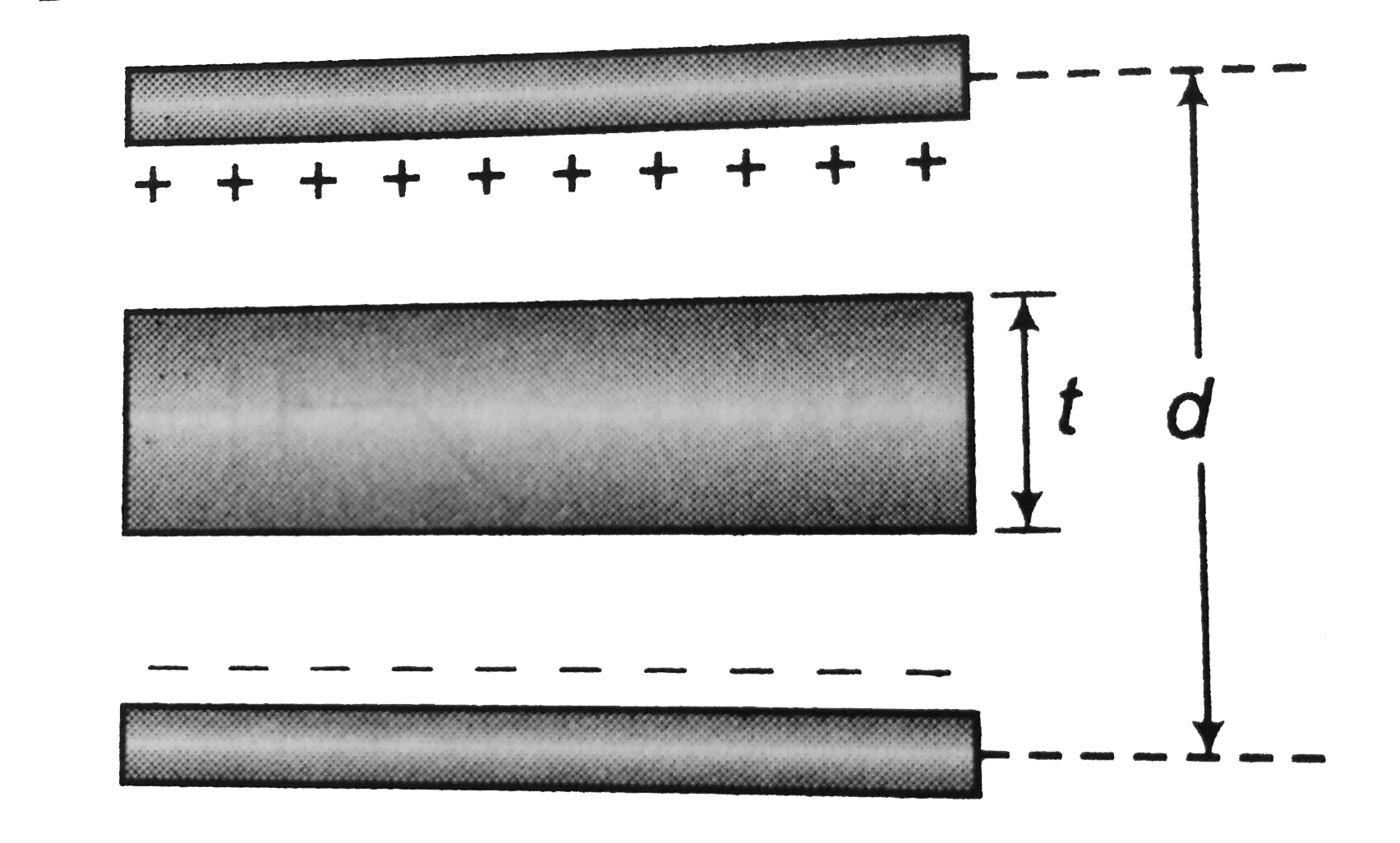
The electric field in the dielectric slab isView SolutionA parallel plate capacitor (without dielectric) is charged by a battery and kept connected to the battery. A dielectric salb of dielectric constant 'k' is inserted between the plates fully occupying the space between the plates. The energy density of electric field between the plates will:
View SolutionA parallel plate capacitor of plate area A and plates separation distance d is charged by applying a potential V0 between the plates. The dielectric constant of the medium between the plates is K. What is the uniform electric field E between the plates of the capacitor ?
View SolutionA parallel plate capacitor has a dielectric (of dielectric constant k) between the plates. The plates are charged to a surface charge density σ0. What is the density of the polarized charge that appear on the surface of the dielectric.
View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionAn uncharged parallel plate capacitor is connected to a battery. The electric field between the plates is 10V/m. Now a dielectric of dielectric constant 2 is inserted between the plates filling the entries space. The electric field between the plates now is
View Solution

