A square loop of side 12 cm and resistance 0.6Ω is placed vertically in the east-west plane. A uniform magnetic field of 0.10 T is set up across the plane in north-east direction. The magnetic field is decreased to zeroin 0.6 s at steady rate. The magnitude of currnet during this time interval is
1.42×10−3A
2.67×10−3A
3.41×10−3A
4.21×10−3A
The correct Answer is:B
To solve the problem step by step, we will follow these steps:
Step 1: Calculate the Area of the Square Loop
The area A of a square loop is given by the formula:
A=side2
Given the side of the square loop is 12 cm, we first convert it to meters:
side=12 cm=0.12 m
Now, calculate the area:
A=(0.12m)2=0.0144m2
Hint: Remember to convert centimeters to meters when calculating area in SI units.
Step 2: Determine the Initial Magnetic Flux
The initial magnetic flux Φ1 can be calculated using the formula:
Φ1=B1⋅A⋅cos(θ)
Where:
- B1=0.10T (initial magnetic field)
- A=0.0144m2 (area calculated in Step 1)
- θ=45∘ (angle between the magnetic field and the normal to the loop)
Now, calculate cos(45∘)=1√2≈0.707:
Φ1=0.10⋅0.0144⋅0.707≈0.00102Wb
Hint: Use the cosine of the angle to find the component of the magnetic field that is perpendicular to the area.
Step 3: Determine the Final Magnetic Flux
The final magnetic flux Φ2 when the magnetic field is zero is:
Φ2=0Wb
Hint: When the magnetic field is zero, the flux through the loop is also zero.
Step 4: Calculate the Change in Magnetic Flux
The change in magnetic flux ΔΦ is given by:
ΔΦ=Φ2−Φ1=0−0.00102=−0.00102Wb
Hint: The change in flux is simply the final flux minus the initial flux.
Step 5: Calculate the Induced EMF
The induced electromotive force (EMF) E can be calculated using Faraday's law:
E=−ΔΦΔt
Where Δt=0.6s:
E=−−0.001020.6≈0.0017V
Hint: The negative sign indicates the direction of the induced EMF, but we are interested in the magnitude.
Step 6: Calculate the Current
Using Ohm's Law, the current I can be calculated as:
I=ER
Where R=0.6Ω:
I=0.00170.6≈0.00283A or 2.83mA
Hint: Make sure to use the resistance in ohms to find the current in amperes.
Final Answer
The magnitude of the current during this time interval is approximately 2.83mA.
The area A of a square loop is given by the formula:
A=side2
Given the side of the square loop is 12 cm, we first convert it to meters:
side=12 cm=0.12 m
Now, calculate the area:
A=(0.12m)2=0.0144m2
The initial magnetic flux Φ1 can be calculated using the formula:
Φ1=B1⋅A⋅cos(θ)
Where:
- B1=0.10T (initial magnetic field)
- A=0.0144m2 (area calculated in Step 1)
- θ=45∘ (angle between the magnetic field and the normal to the loop)
Φ1=0.10⋅0.0144⋅0.707≈0.00102Wb
The final magnetic flux Φ2 when the magnetic field is zero is:
Φ2=0Wb
The change in magnetic flux ΔΦ is given by:
ΔΦ=Φ2−Φ1=0−0.00102=−0.00102Wb
The induced electromotive force (EMF) E can be calculated using Faraday's law:
E=−ΔΦΔt
Where Δt=0.6s:
E=−−0.001020.6≈0.0017V
Using Ohm's Law, the current I can be calculated as:
I=ER
Where R=0.6Ω:
I=0.00170.6≈0.00283A or 2.83mA
The magnitude of the current during this time interval is approximately 2.83mA.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSERTION AND REASON
15 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
Similar Questions
A square loop of side 10cm and resistance 0.5Ω is placed vertically in the east-west plane. A uniform magnetic field of 0.10T is set up across the plane in the north-east direction. The magnetic field is decreased to zero in 0.70s at a steady rate. The magnitude of current in this time-interval is.
View SolutionA square loop of side 10 cm and resistance 0.5 Ω is placed vertically in the east-west plane. A uniform magnetic field of 0.10 T is set up across the plane in the north-east direction. The magnetic field is decreased to zero in 0.70 s at a steady rate. Determine the magnitudes of induced emf and current during this time-interval.
View SolutionA square metal wire loop of side 20 cm and resistance 2Ω is moved with a constant velcoity v0 in a uniform magnetic field of induction B = 1 Wb/m2 as shown in the figure. The magnetic field lines are perpendicular to the plane of the loop. The loop is connected to a network of resistance each of value 5Ω. The resistances of the lead wires BF and AE are negligible. What should be the speed of the loop, so as to have a steady current of 2 mA in the loop? Give the direction of current in the loop.

View SolutionShows a coil placed in a decreasing magnetic field applied perpendicular to the plane of the coil. The magnetic field is decreasing at a rate of 10Ts−1. Find out current in magnitude and direction of current.

View SolutionShows a coil placed in a decreasing magnetic field applied perpendicular to the plane of the coil. The magnetic field is decreasing at a rate of 10Ts−1. Find out current in magnitude and direction of current.

View SolutionA closed loop with the geometry shown in Fig. 3.9 is placed in a uniform magnetic field direction into the plane of the paper. If the magnetic field decreases with time, determine the direction of the induced emf in this loop.
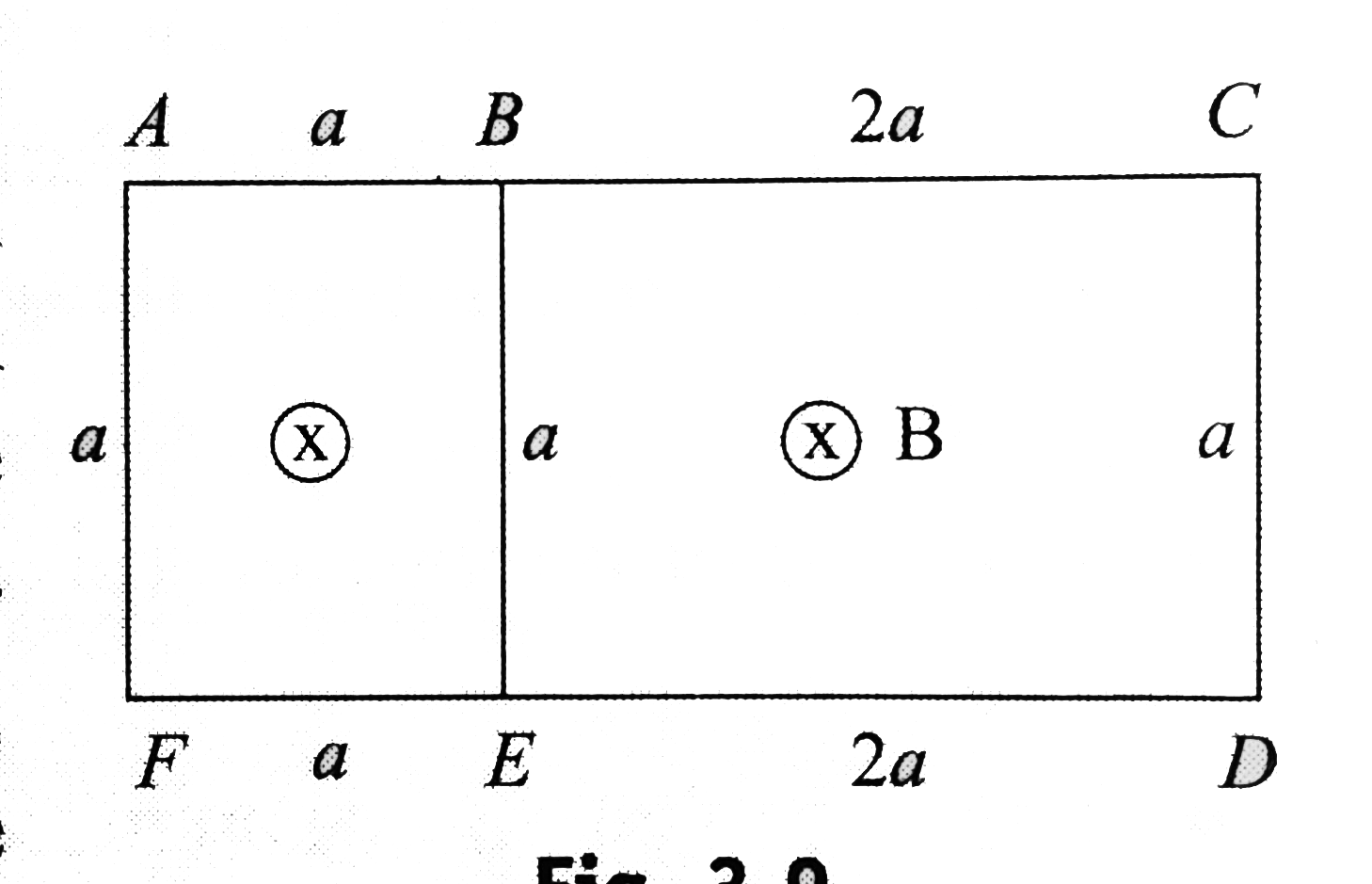
View SolutionA square loop of side 10 cm with its side parallel to X, and y axis is kept in a uniform magnetic field pointing towards positive z-direction. If magnetic field changes at the rate of 0.1 T/s, then induced emf is
View SolutionA square loop of side 10 cm and resistance 1 Omega is moved towards right with a constant velocity V_0 as shown in . The left arm of the loop is in a uniform magnetic field of 2 T. the field is perpendicular ot the plane of the drawing and is going into it. the loop is connected to a network of resistors each of value 3 Omega with what speed should the loop be moved so that a steady curfrent of 1 mA flows in the loop.

View SolutionA circular loop of area 1cm2 , carrying a current of 10 A , is placed in a magnetic field of 0.1 T perpendicular to the plane of the loop. The torque on the loop due to the magnetic field is
View SolutionA square loop of side L, resistance R placed in a uniform magnetic field B acting normally to the plane of the loop. If we attempt to pull it out of the field with a constant velocity v, then the power needed is
View Solution