A particle projected with velocity $v_{0}$ , strikes at right angles $α$ plane through the point of projection and of inclination $β$ with the horizontal. Find the height of the point struck, from horizontal plane through the point of projection.

Text Solution

Verified by Experts

Let $α$ be the angle between the velocity of projection and the inclined plane.
$v_{\otimes .} = v_{0} \cos α, v_{o y .} = v_{0} \sin α$
$a_{x .} = - g β a_{y .} = - g \cos β$
$\Rightarrow v_{x .} (t) = v_{0} \cos α - g \sin β t$
At the point of impact $v_{x .} = 0 \Rightarrow t = \frac{v_{0} \cos α}{g \sin β}$ ........i

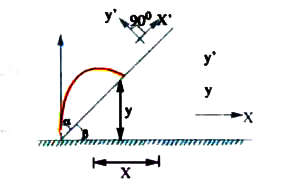
At y. at the point is zero
$\Rightarrow v_{0} \sin α t - \frac{1}{2} g \cos β t^{2} = 0 \Rightarrow t = \frac{2 v_{0} \sin α}{g \cos β}$ ......ii
From ia nd ii $\frac{v_{0} \cos α}{g \sin β} = \frac{2 v_{0} \sin α}{g \cos β}$
$\tan α = \frac{1}{2} \cot β$ .................iii
$x = v_{0} \cos (α + β) t$
$= V_{0} [\cos α \cos β - \sin α β] \frac{V_{0} \cos α}{g \sin β} = \frac{V_{0}^{2}}{g} [\cos^{2} α \cot β - \sin α \cos α]$
$= \frac{v_{0}^{2}}{g} [{(\frac{2}{\sqrt{4 + \cot^{2} β}})}^{2} \cot β - \frac{\cot β}{\sqrt{4 + \cot^{2} β}} \frac{2}{\sqrt{4 + \cot^{2} β}}]$
(using $\tan α = \frac{1}{2} \cot β) = \frac{v_{0}^{2}}{g} . \frac{2 \cot β}{4 + \cot^{2} β}$
From figure
$∴ y = x \tan β = \frac{V_{0}^{2}}{g} . \frac{2 \cot β}{4 + \cot^{2} β} \tan β \Rightarrow y = \frac{2 V_{0}^{2}}{g (4 + \cot^{2} β)}$

Updated on:21/07/2023

A projectile is projected and it takes $9 s$ to reach in the horizontal plane through the point of projection. In its path it passes a point $P$ after $4 s$ . The height of $P$ above the horizontal plane is
A $10 m$
B $50 m$
C $100 m$
D $200 m$
A particle is projected with velocity v at an angle $θ$ aith horizontal. The average angle velocity of the particle from the point of projection to impact equals
A $\frac{g cos θ}{θ v}$
B $\frac{g}{v sin θ}$
C $\frac{g}{v θ}$
D $\frac{g θ}{v sin θ}$
A particle is projected with a certain velocity at an angle $α$ above the horizontal from the foot of an inclined plane of inclination $30^{\circ}$ . If the particle strikes the plane normally then $α$ is equal to
A $30^{\circ} + {tan}^{- 1} (\frac{\sqrt{3}}{2})$
B $45^{\circ}$
C $60^{\circ}$
D $30^{\circ} + {tan}^{- 1} (2 \sqrt{3})$