A rectangular tank having base 15 cm × 20 cm is filled with water (density ρ=1000kgm−3 ) up to 20 cm height. One end of an ideal spring of natural length 20 cm and force constant k 280 N/m is fixed to the bottom of a tank so that spring, remains vertical. This system is in an elevator moving downwards with acceleration a=2ms−2 Cubical block of side l=10 cm and mass m= 2 kg is gently placed over in figure.
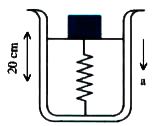
If block is attached to spring and slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations (g = 10 m/s2)
5√2πHz
Topper's Solved these Questions
- OSCILLATIONBOOK - AAKASH SERIESCHAPTER - OSCILLATIONEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
12 Videos
- OSCILLATIONBOOK - AAKASH SERIESCHAPTER - OSCILLATIONEXERCISE - NUMERICAL EXERCISE (LEVEL-1)
33 Videos
- NEWTONS LAWS OF MOTION BOOK - AAKASH SERIESCHAPTER - NEWTONS LAWS OF MOTION EXERCISE - ADDITIONAL PRACTICE EXERCISE PRACTICE SHEET (ADVANECED) (Matrix Matching Type Questions)
1 Videos
- OSCILLATIONSBOOK - AAKASH SERIESCHAPTER - OSCILLATIONSEXERCISE - PRACTICE EXERCISE - SPRINGS
16 Videos
Similar Questions
A rectangular tank having base 15cm×20cm is filled with water (density ρ=1000kg/m3) up to 20cm and force constant K=280N/m is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration a=2m/s2. A cubical block of side l=10cm and mass m=2kg is gently placed over the spring and released gradually, as shown in the figure.
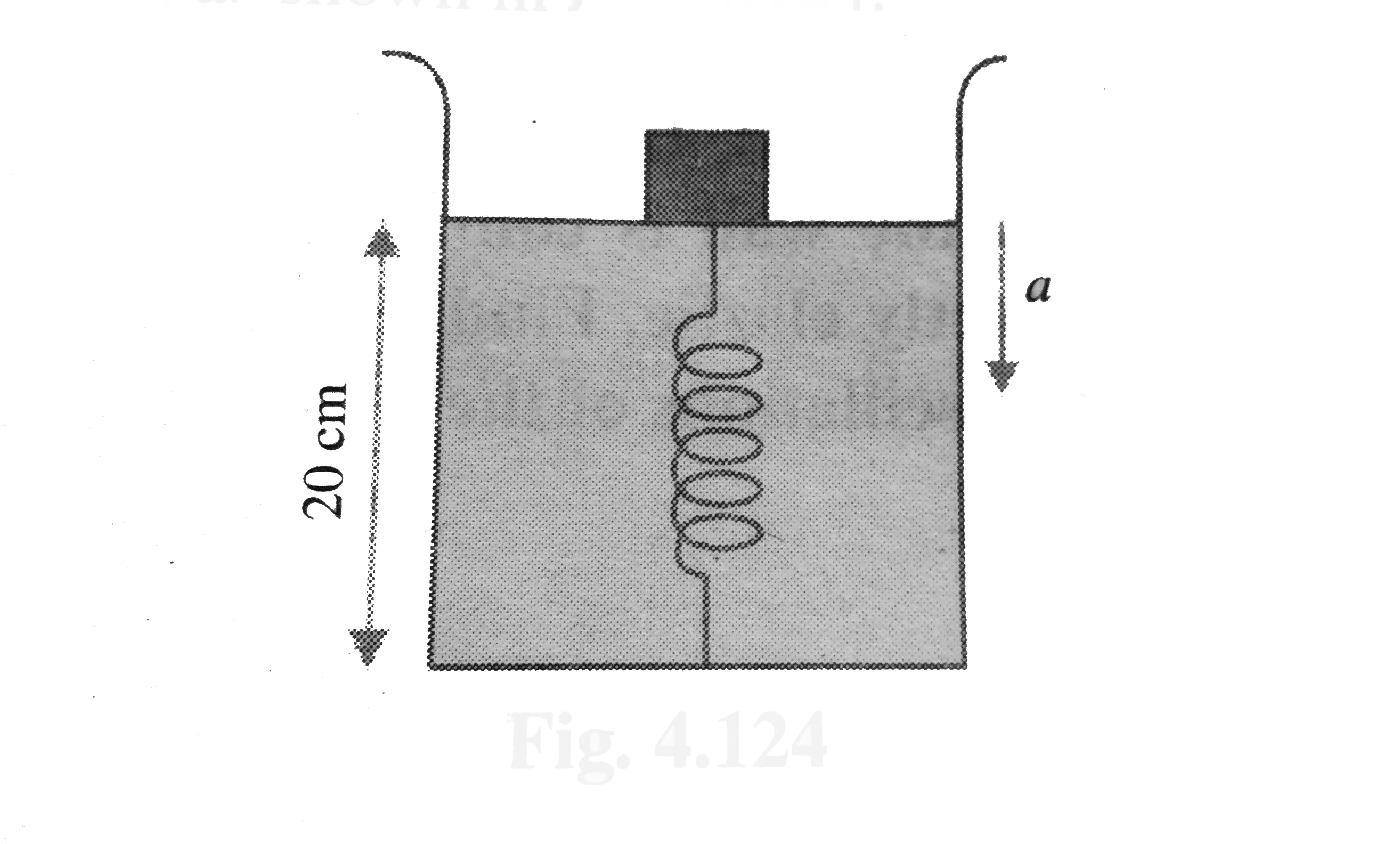
a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.
View SolutionA rectangular tank having base 15cm×20cm is filled with water (density ρ=1000kg/m2) up to 20cm and force constant K=280N/m is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration a=2m/s2. A cubical block of side l=10cm and mass m=2kg is gently placed over the spring and released gradually, as shown in the figure.

a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.
View Solution
Knowledge Check
A block of mass 2kg is connected with a spring of natural length 40cm of force constant K=200N/m. The cofficient of friction is μ=0.5. When released from the given position, acceleration of block will be
 AzeroB2m/s2C6m/s2D8m/s2
AzeroB2m/s2C6m/s2D8m/s2A tank is filled with water of density 1 g per cm3 and oil of density 0.9gpercm3 The height of water layer is 100 cm and of the oil layer is 400 cm If g=980cm/s2, then the velocity of efflux from an opening in the bottom of the tank is :
A√900×980cm/sB√1000×980cm/sC√920×980cm/sD√950×980cm/sA tank is filled with water of density 1 g per cm3 and oil of density 0.9gpercm3 The height of water layer is 100 cm and of the oil layer is 400 cm If g=980cm/s2, then the velocity of efflux from an opening in the bottom of the tank is :
A√900×980cm/sB√1000×980cm/sC√920×980cm/sD√950×980cm/s
A block of mass 2kg is connected with a spring of natural length 40cm of force constant K=200N/m. The cofficient of friction is μ=0.5. When released from the given position, acceleration of block will be

View SolutionA spring has natural length 40cm and spring constant 500N/m. A block of mass 1kg is attached at one end of the spring and other end of the spring is attached to a ceiling. The block is relesed from the position, where the spring has length 45cm.
View SolutionA tank is filled with water of density 1gcm−3 and oil of density 0.9gcm−3 . The height of the water layer is 100 cm and of the oil layer is 400 cm. If g = 980 cms−2 , then the velocity of efflux from an opening in the bottom of the tank is
View SolutionA spring has natural length 40cm and spring constant 500N/m. A block of mass 1kg is attached at one end of the spring and other end of the spring is attached to a ceiling. The block is relesed from the position, where the spring has length 45cm.
View SolutionA rectangular tank 25 cm long and 20 cm wide contains water to a depth of 5 cm . A metal cube of side 10 cm is placed in the tank so that one face of the cube rests on the bottom of the tank . Find how many litres of water must be poured into the tank so as to just cover the cube ?
View Solution
