Show that are rate of flow of heat through a spherical shell , the inner and outer walls of which have radii r1 and r2 and are maintained at differnet unifrom temperature θ1 and θ2 respectievly , is given by H=4Kr1r2θ1−θ2r2−r1 where K is thermal conductivity of material of shell . He obtain the expression for temperature distributions.

Let O be the centre of spherical shell , having inner and outer radii r1 and r2 respectively , i.e., OA=r1,OB=r2 . Let θ1 and θ2 be the steady temperature of inner and outerall (θ1>θ2) respectively . The shell may be supposed to be formed of large number of thin concentric spherical shells . Consider one such shell of radius r and thickness dr .
Let θ and θ+dθ the respectively temperature at distance r and r+dr from centre O.
The temperature gradient =dθdr∴ Rate of flow of heat across the surface of the surface of the shell .
H=−KAδθdr(or)H=−K(4πr2.dθdr)..............(1)
K being thermal conductivity of the material of shelll.
Due to symmetic nature of shell H is same in all directions is steady state.
Therefore from (1)
dθ=−H4πKdrr2...........(2)∫θ2θ1dθ=H4πKr2∫ridrr2or[θ]θ2θ1=−H4πK[−1r]r2r1
(or) θ2−θ1=H4πK[1r2−1r1](or)θ1−θ2=H4πK[1r1−1r2]
The gives rate of flow of heat H=4πK(θ1−θ2)(1r1−1r2)...............(3)
Temperature distribution :
Integrating equaiton (2) betwee limits θ1 and θ we get θ2∫θ1dθ=−H4πkr∫ridrr2
(or) θ−θ1=−H4πK[−1r]rri............(4) substituting value of H from (3) in (4) .
we get θ=θ1+(θ1−θ2)(1r−1r1)(1r1−1r2)..............(5)
This is the required expression .
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - TRANSMISSION OF HEAT EXERCISE - TEST YOUR SELF6videos
TRANSMISSION OF HEAT
- BOOK - AAKASH SERIESCHAPTER - TRANSMISSION OF HEAT EXERCISE - VERY SHORT ANSWER TYPE QUESTIONS28videos
TRANSMISSION OF HEAT
- BOOK - AAKASH SERIESCHAPTER - THERMOMETRY EXERCISE - Numerical Exercise (LEVEL- 1)11videos
THERMOMETRY
- BOOK - AAKASH SERIESCHAPTER - TRANSMISSION OF HEATEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED)) (Integer/Subjective Type Questions)3videos
TRANSMISSION OF HEAT
Similar Questions
A hole of radius r1 is made centrally in a uniform circular disc of thickness d and radius r2 . The inner surface (a cylinder of length d and radius r1) is maintained at a temperature θ1 and the outer surface (a cylinder of length d and radius r2) is maintained at a temperature θ2(θ1>θ2) .The thermal conductivity of the material of the disc is K. Calculate the heat flowing per unit time through the disc.
View SolutionCalculated thermal conductance for radial flow of a spherical sheel of inner and outer radius r1and r2. Assume that thermal conductivity of the material is K
View Solution
Knowledge Check
- Question 1 - Select One
Two identical rods are made of different materials whose thermal conductivities are K1andK2. They are placed end to end between two heat reservoirs at temperatures θ1andθ2. The temperature of the junction of the rod is
 Aθ1+θ22BK1θ1+K2θ2K1+K2CK1θ2+K2θ1K1+K2DK1θ1+K2θ2[K1−K2]
Aθ1+θ22BK1θ1+K2θ2K1+K2CK1θ2+K2θ1K1+K2DK1θ1+K2θ2[K1−K2] - Question 2 - Select One
Two different metal rods of the same length have their ends kept at the same temperature θ1, and θ2with θ2>θ1. If A1 and A2 are their cross-sectional areas and K1 and K2 their thermal conductivities, the rate of flow of heat in the two rods will be the same it:
AA1A2=k1k2BA1A2=k2k1CA1A2=k1θ1k2θ2DA1A2=k2θ2k1θ1
Calculated thermal conductance for radial flow of a spherical sheel of inner and outer radius r1and r2. Assume that thermal conductivity of the material is K
View SolutionFind the rate of heat flow through a cross section of the rod shown in figure (θ2>θ1) . Thermal conductivity of the material of the rod is K.
 View Solution
View SolutionTwo identical rods are made of different materials whose thermal conductivities are K1andK2. They are placed end to end between two heat reservoirs at temperatures θ1andθ2. The temperature of the junction of the rod is
 View Solution
View SolutionA hole of radius r1 is made centrally in a uniform circular disc of thickness d and radius r2 . The inner surface (a cylinder of length d and radius r1 is maintained at a temperature θ1 and the oouter surface (a cylinder of length d and radius r2 . The is maintianed at a temperature θ2(θ1>θ2) .The thermal conductivity of the material of the dics is K. Calculate the heat flowing per unit time through the disc.
View SolutionTwo slabs are placed adjacently having thermal resistance R1&R2, their free ends maintained at temperature of θ1, & and θ2, respectively. Find the temperature of junction.
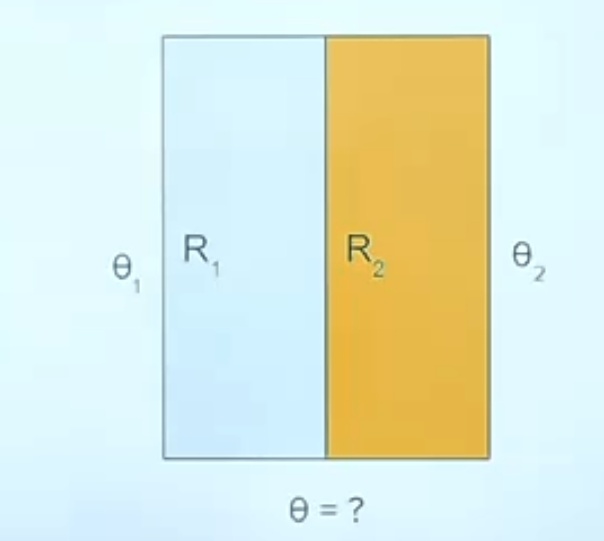 View Solution
View SolutionFind the rate of heat flow through a cross section of the rod shown in figure (θ2>θ1 . Thermal conductivity of the material of the rod is K.
 View Solution
View Solution

