A massless spool of inner radius r, outer radius R is placed against vertical wall and tilted split floor as shown. A light inextensible thread is tightly wound around the spool through which a mass m is hanging. There exists no friction at point A, while the coefficient of friction between spool and point B is μ . The angle between two surface is .
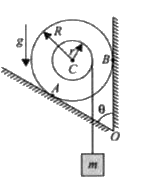
The magnitude of force on the spool at B in order to maintain equilibrium is
The magnitude of force on the spool at B in order to maintain equilibrium is mg
The minimum value of μ for the system to remain in equilibrium is
The minimum value of μ for the system to remain in equilibrium is .
The correct Answer is:A, D
From the free body diagram we can write that,
mgr= jR ... (i)
….(ii)
…(iii)
From (i),
Net force at B:
For minimum value of
Topper's Solved these Questions
- MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONBOOK - MTG-WBJEECHAPTER - MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONEXERCISE - WB-JEE Previous years questions
17 Videos
- MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONBOOK - MTG-WBJEECHAPTER - MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTIONEXERCISE - WB-JEE Previous years questions
17 Videos
- MODEL TEST PAPTER BOOK - MTG-WBJEECHAPTER - MODEL TEST PAPTER EXERCISE - MCQs
80 Videos
- NUCLEAR PHYSICBOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS
5 Videos
Similar Questions
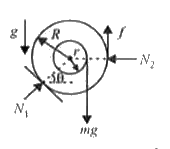
A massless spool of inner radius r outer radius R is placed against a vertical wall and a titled split floor as shown. A light inextensible thread is tightly wound around the spool through which a mass m is hainging. There exists no friction at point A, while the coefficient of friction between the spool and point B is μ. The angle between the two surface is θ

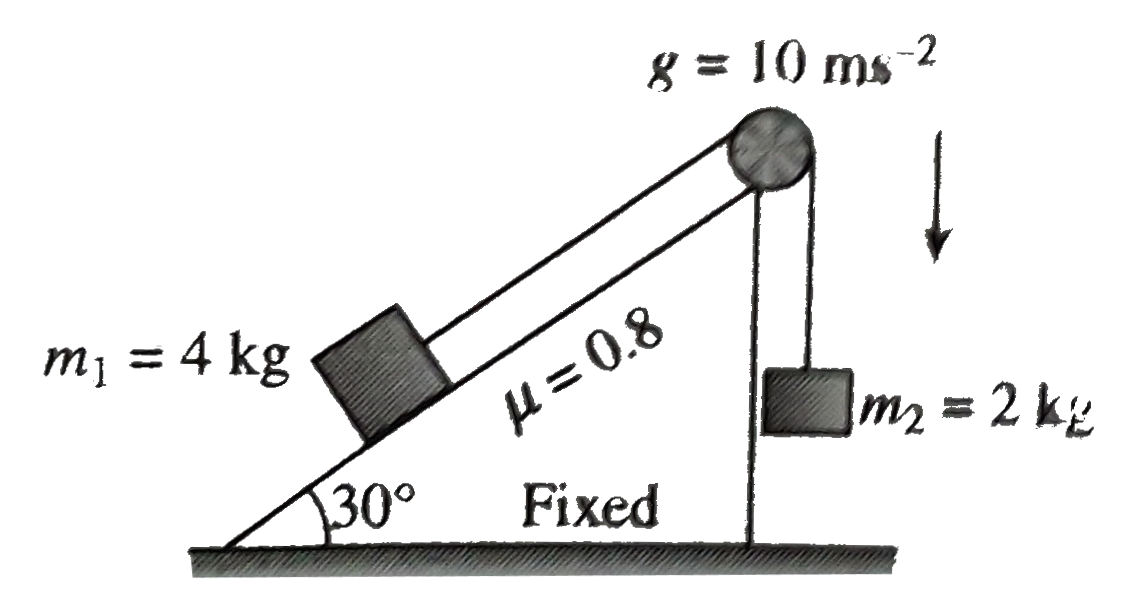
View SolutionTwo blocks of masses m1 and m2 are connected through a massless inextensible string .A block of mass m1 is placed at the fixed rigid inclined surface while the block of mass m2 hanging at the other end of the string which is passing through a fixed massless frictionless pulley shown in figure The coefficient of static friction between the block and the inclined plane is 0.8 The system of masses m1 and m2 released from rest
View Solution
Knowledge Check
A massless spool of inner radius r outer radius R is placed against a vertical wall and a titled split floor as shown. A light inextensible thread is tightly wound around the spool through which a mass m is hainging. There exists no friction at point A, while the coefficient of friction between the spool and point B is μ. The angle between the two surface is ρ
 AThe magnitude of the force on the spool at B in order to maintain equilibrium is mg√(rR)2+(1−rR)21tan2θBThe magnitude of the force on the spool at B in order to maintain equilibrum is mg(1−rR)1tanθCThe minimum value of μ for the system to remain in equilibrium is cotθ(R/r)−1DThe minimum values of μ for the system to remain in equilibrium is tanθ(R/r)−1
AThe magnitude of the force on the spool at B in order to maintain equilibrium is mg√(rR)2+(1−rR)21tan2θBThe magnitude of the force on the spool at B in order to maintain equilibrum is mg(1−rR)1tanθCThe minimum value of μ for the system to remain in equilibrium is cotθ(R/r)−1DThe minimum values of μ for the system to remain in equilibrium is tanθ(R/r)−1Two blocks of masses m1 and m2 are connected through a massless inextensible string .A block of mass m1 is placed at the fixed rigid inclined surface while the block of mass m2 hanging at the other end of the string which is passing through a fixed massless frictionless pulley shown in figure The coefficient of static friction between the block and the inclined plane is 0.8 The system of masses m1 and m2 released from rest
 AThe tension in the string is 20N after releasing the systemBThe contact force by the inclined surface on the block in along normal to the inclined surfaceCThe magnitude of contact force by the inclined surface on the m1 is 20√3NDNone of these
AThe tension in the string is 20N after releasing the systemBThe contact force by the inclined surface on the block in along normal to the inclined surfaceCThe magnitude of contact force by the inclined surface on the m1 is 20√3NDNone of theseA spool of mass M and radius 2R lies on an inclined plane as shown in the figure. A light thread is wound around the connecting tube of the spool and its free end carries a weight of mass m. The value of m so that system is in equilibrium is
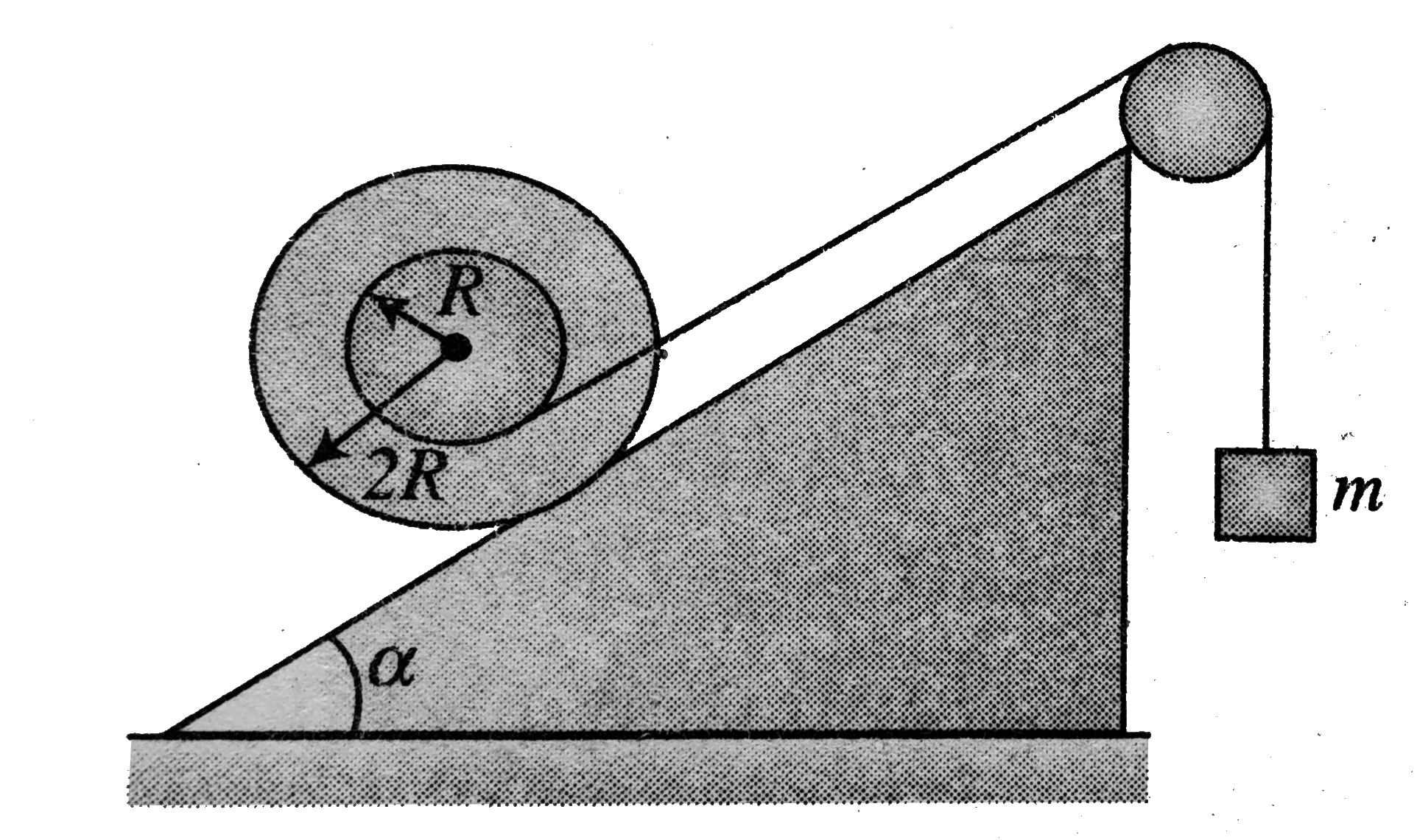 A2MsinαBMsinαC2MtanαDMtanα
A2MsinαBMsinαC2MtanαDMtanα
A spool of mass M and radius 2R lies on an inclined plane as shown in the figure. A light thread is wound around the connecting tube of the spool and its free end carries a weight of mass m. The value of m so that system is in equilibrium is

View SolutionA ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
View SolutionA ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
View SolutionA solid cylinder of radius r and mass m is placed with no initial velocity on a moving belt 'B'. It is placed against a rough wall ’A', which restricts the sliding of the cylinder on the belt as shown in the figure. Assuming that the
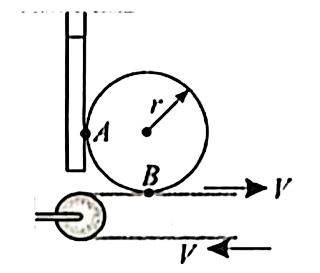
coefficient of friction at A and B is μ(<1), the angular acceleration of the cylinder will be
View SolutionA uniform cylinder of mass m lies on a fixed plane inclined at a angle θ with the horizontal. A light string is tied to the cylinder at the rightmost point, and a mass m hangs from the string as shown. Assume that the coefficient of friction between the cylinder and the incline plane is sufficiently large to prevent slipping. for the cylinder to remain static the value of m is

View Solution
