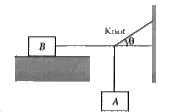
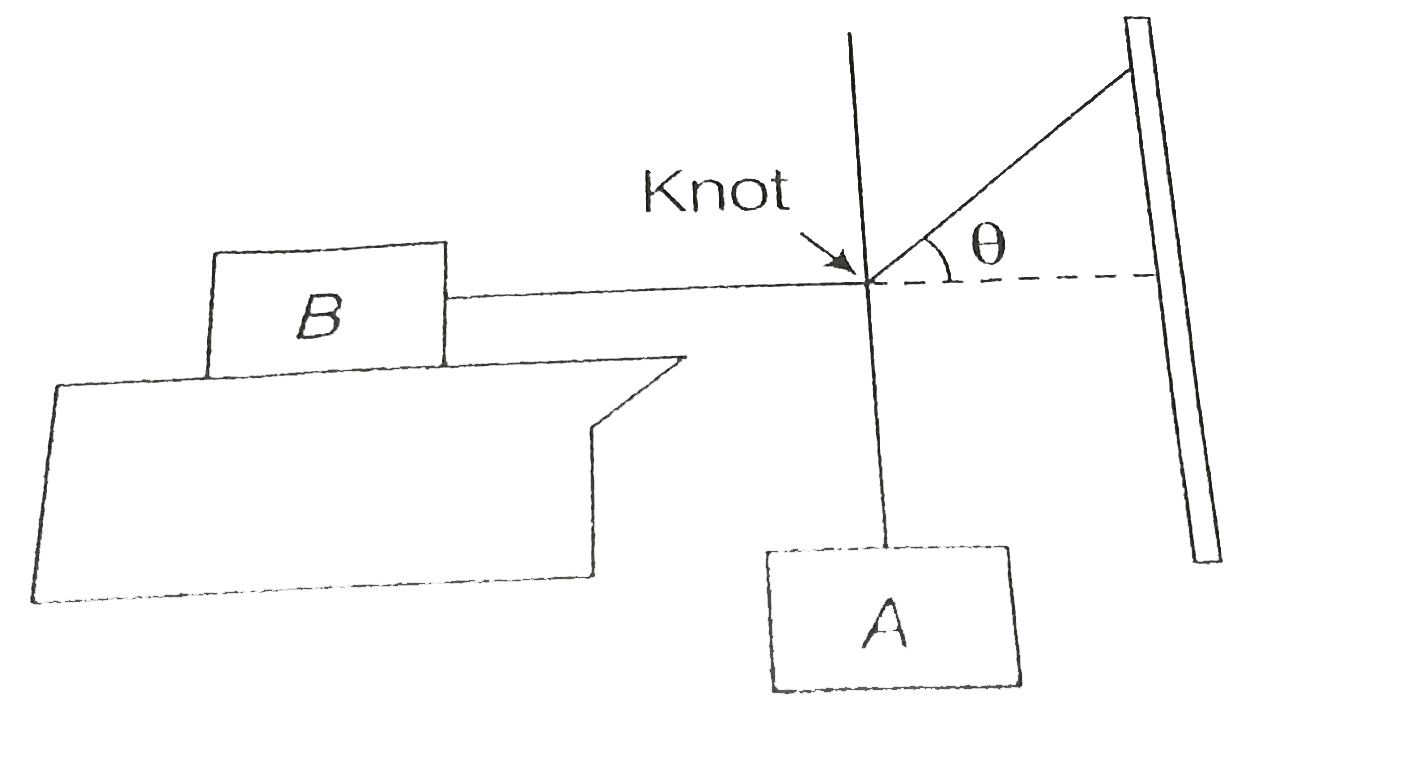
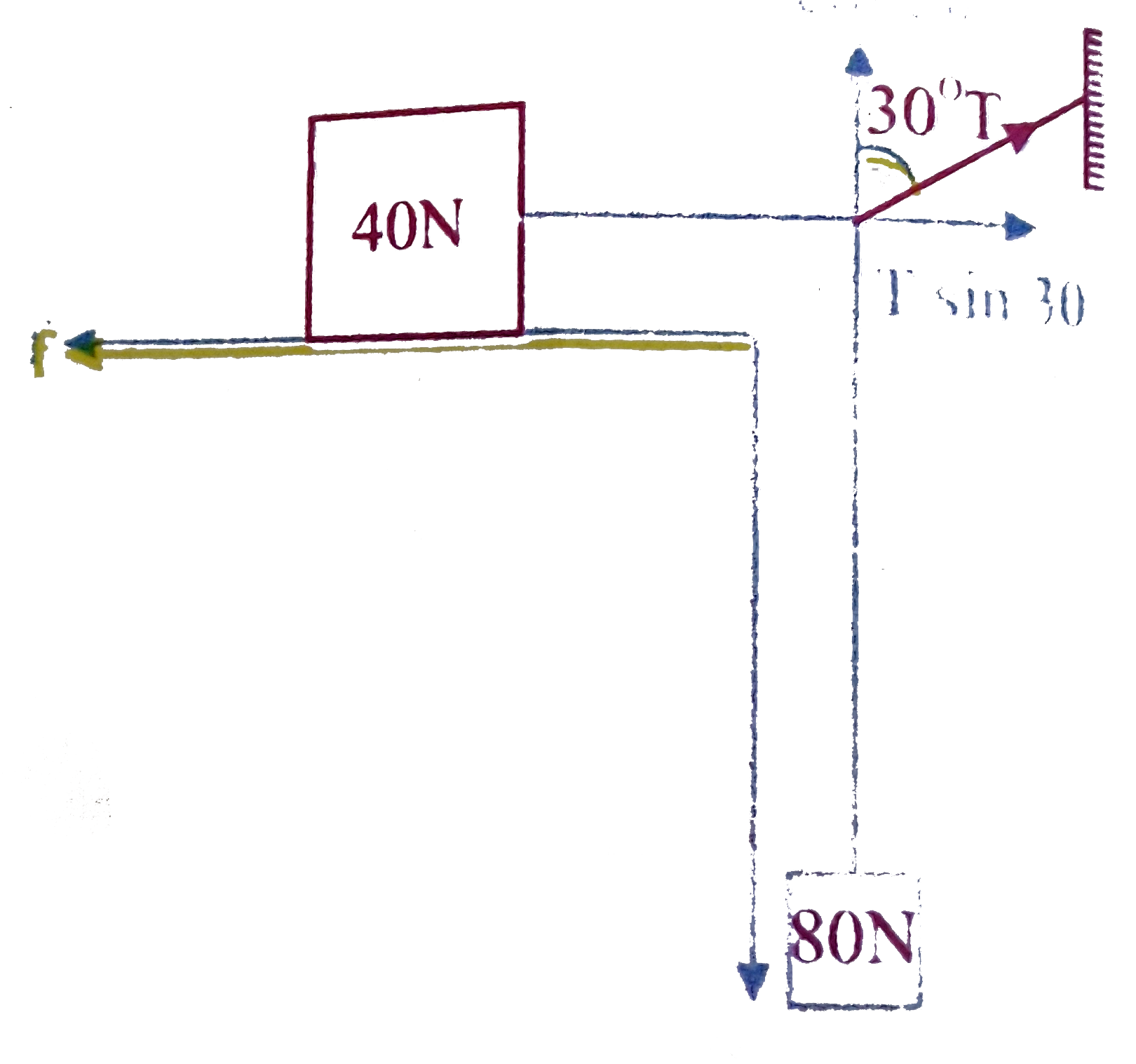
Block B lying on a table weighs W. The coefficient of static friction between the block and the table is μ. Assume that the cord between B and the knot is horizontal. The maximum weight of the block A for which the system will be stationary is

A

$\frac{W tan θ}{μ}$

B

$μ W tan θ$

C

$μ W \sqrt{1 + {tan}^{2} θ}$

D

$μ W sin θ$

Text Solution

Verified by Experts

The correct Answer is:B

Weight of block B = W
Weight of block A = W.
T = tension in the string
Normal on block B, N= W
Friction force on block B,f= μN= μW

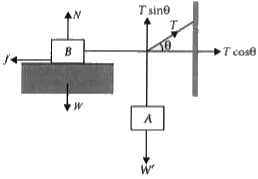
System will be in equilibrium, if
$T cos θ = f = μ W$ ...(i)
$T sin θ = W .$ ...(ii)
Divide eqn (ii) by eqn (i), we get
$\frac{sin θ}{cos θ} = \frac{W .}{μ W}, ∴ W . = μ W tan θ$

Updated on:21/07/2023

|

Class 12 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Block B lying on a table weighs w. The coefficient of static friction between the block and the table is mu. Assume that the cord between B and the knot is horizontal. The maximum weight of the block A for which the system will be stationary is

A $\frac{w tan θ}{μ}$
B $μ w tan θ$
C $μ w \sqrt{1 + {tan}^{2} θ}$
D $μ w sin θ$
A block is stationary on an inclined plane If the coefficient of friction between the block and the plane is $μ$ then
.
A $μ > tan θ$
B $f = m g sin θ$
C $f = μ m g cos θ$
Dthe reaction of the ground on the block is mg cos $θ$
The system shown is just on the verge of slipping. The coefficient of static friction between the block and the table top is :

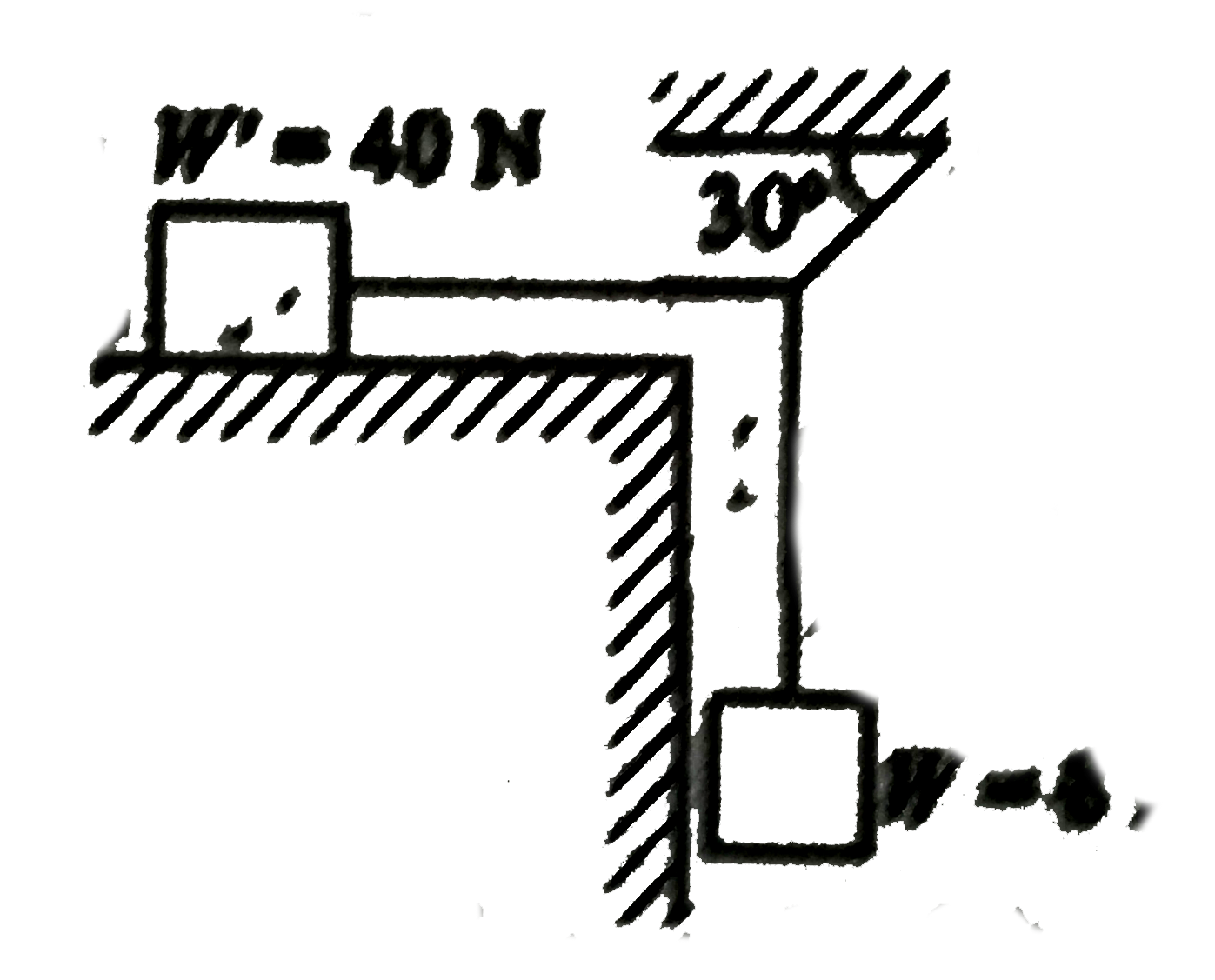A0.5
B0.95
C0.15
D0.35

MTG-WBJEE-MOTION OF CENTRE OF MASS, CONNECTED SYSTEMS, FRICTION-WB-JEE Previous years questions

Home
Ask Doubt
Profile