A heating element of piston resistance r is fitted inside an adiabatic cylinder which carries a frictionless piston of mass m and crosssection A as shown in diagram. The cylinder contains one mole of an ideal diatomic gas. The current flows through the element such that the temperature rises with time t as ΔT=alpaht+12βt2? (α and β are constants), while pressure remains constant. The atmospheric pressure above the piston is P0. Then
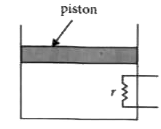
the rate of increase in internal energy is 52R(α+βt)
the current flowing in the element is √52rR(α+βt)
the piston moves upwards with constant acceleration,
the piston moves upwards with constant speed.
The correct Answer is:A, C
increase in internal energy dU=nCVdT
∴ Rate of increase in internal energy
dUdt=nCVdTdt
here n=1,dT=αt+12βt2∴dTdt=α+βt
for diatomic gas CV=52R,CP=72R
∴dUdt=1×52R×(α+βt)=52R(α+βt)
heat supplied by the heating element to the gas at constant pressure
dQ=nCPdT
Rate of heta supplied
dQdt=nCPdtdt=1×72R×(α+βt) ( using (i ))
=72R(α+βt)
Let I be current flowing in the element
∴I2r=72R(α+βt)
I2=72rR(α+βy)⇒I=√72rR(α+βt)
according to ideal gas equation PV=nRT
At contain pressure PdVnRT
PdV=nR(αt+12βt2)
work done w=pdV
=nR(αt+12βt2)
Let F be force exerted by the gas on the piston of area A
∴P=FAorF=pA
let the piston moved upwards by a distance x .
∴W=Fxorx=WF=nRpA(αt+12βt2)( using (ii ) )
veloity v=dxdt=nRPA(α+βt)
Acceleration a=dvdt=nRPAβ
Topper's Solved these Questions
- BULK PROPERTIES OF MATTERBOOK - MTG-WBJEECHAPTER - BULK PROPERTIES OF MATTEREXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (ONE OR MORE THAN ONE OPTION CORRECT TYPE)
1 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENT BOOK - MTG-WBJEECHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENT EXERCISE - WB JEE Previous Years Questions
14 Videos
Similar Questions
A resistance coil of resistance r connected to an external battery, is placed inside an adiabatic cylinder fitted with a frictionless platon of mass m and same area A. Initially cylinder contains one mole of ideal gas he. A current through the coil such that temperature of gas varies as T=T0+at+bt2, keeping pressure constant with time t. Atosphere pressure above piston is P0. Find
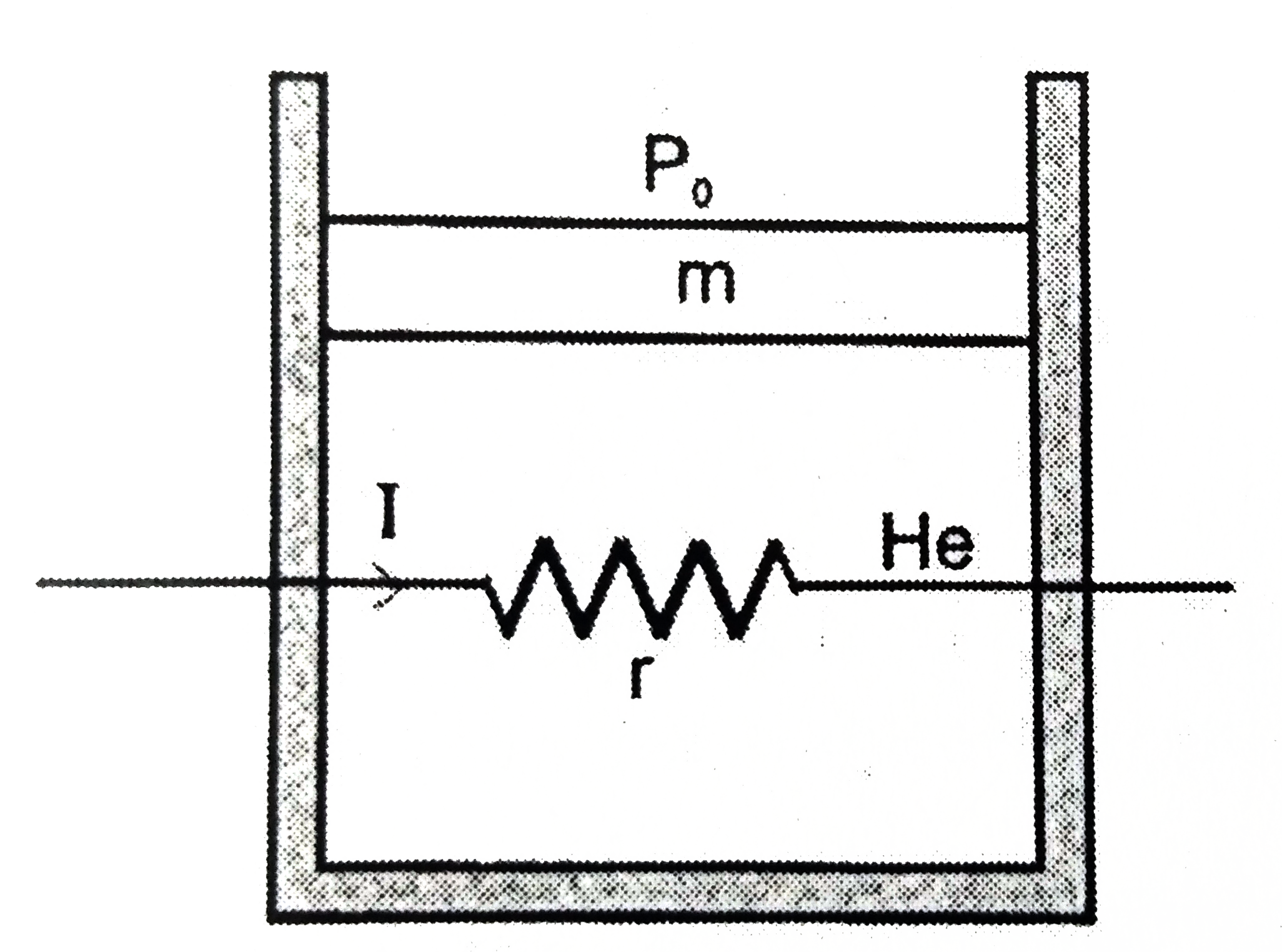
(a) Current l flowing through the coil as function of time and
(b) Speed of piston as function of time.
View SolutionA resistance coil, connected to an external battery is placed inside an adiabatic cylinder filled with frictionless piston of mass m and containing an ideal gas. A current i flows through the coil, which has resistance R. The speed with which the piston must move in order to keep the temperature of the gas constant will be
View Solution
Knowledge Check
A resistance coil, connected to an external battery is placed inside an adiabatic cylinder filled with frictionless piston of mass m and containing an ideal gas. A current i flows through the coil, which has resistance R. The speed with which the piston must move in order to keep the temperature of the gas constant will be
 Ai2R/mgBiR/mgC0DData is insufficient.
Ai2R/mgBiR/mgC0DData is insufficient.The temperature (T) of one mole of an ideal gas varies with its volume (V) as T=−αV3+βV2, where αandβ are positive constants. The maximum pressure of gas during this process is
Aαβ2RBβ2R4αC(α+β)R2β2Dα2R2βThe charge flowing through a resistance R varies with time tasQ=at−bt2. The total heat produced in R is
Aα2R2βBα3R6βCα3RβDNone
The temperature (T) of one mole of an ideal gas varies with its volume (V) as T=−αV3+βV2, where αandβ are positive constants. The maximum pressure of gas during this process is
View SolutionIf →a and →b are two unit vectors and θ is the angle between them, then the unit vector along the angular bisector of →a and →b will be given by
View SolutionAn ideal gas enclosed in a cylinder fitted with a frictionless and weightless piston, pushes it outside. What would be the sign convention for the work ?
View SolutionAn ideal gas enclosed in a cylinder fitted with a frictionless and weightless piston, pushes it outside. What would be the sign convention for the work ?
View SolutionA vertical cylinder with heat - conducting walls is closed at the bottom and is fitted with a smooth light piston. It contains one mole of an ideal gas. The temperature of the gas is always equal to the surrounding 's temperature, T0 The piston is moved up slowly to increase the volume of the gas to η times. Which of the following is incorrect?
View Solution
