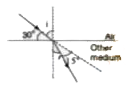
Light of wavelength in air in incident on a plane boundary between air and another medium at an angle with the plane boundary. As if enters from air into the other medium, it deviates by 15 towards the normal. Find refractive index of the medium, and also the wavelength of given light in the medium?
Angle of incidence
As the ra bends towards the normal, it deviates by an angle (given)
Applying snell.s law
In terms of wavelength
Topper's Solved these Questions
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE I
95 Videos
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE 1B
45 Videos
- PHYSICAL WORLDBOOK - AAKASH SERIESCHAPTER - PHYSICAL WORLDEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)
6 Videos
Similar Questions
Light of wavelength 4500∘ in air is incident on a plane boundary between air and another medium at an angle 30∘ with the plane boundary. As it enters from air into the other medium, it deviates by 15∘ s towards the normal Find refractive index of the medium and also the wavelength of given light in the medium.
View SolutionFind the speed of light of wavelength λ=600nm (in air) in a medium of refractive index 5/3 and also wavelength of this light in medium.
View Solution
Knowledge Check
A light wave enters from air into a medium of refractive index 1.5 . The speed of light in the medium will be
A2×108m/sB4.5×108m/sC3×108m/sDnone of theseA light wave enters from air into a medium of refractive index 1.5. The speed of light in the medium will be
A2×108m/sB4.5×108m/sC9×108m/sD(330/1.5)×108m/s
On entering in a medium from air, the speed of light becomes half of its value in air. Find the refractive index of that medium with respect to air.
View SolutionFind the speed of light of wavelength λ=780nm (in air) in a medium of refractive index μ=1.55.
(b) What is the wavelength of this light in the given medium ?
View SolutionFind the speed of light of wavelength λ=780nm (in air) in a medium of refractive index μ=1.55.
(b) What is the wavelength of this light in the given medium ?
View SolutionA light way deviates by 30∘ (which is one third of the angle of incidence) when it gets refracted from vacumm to a medium. Find the refractive index of the medium.
View SolutionLight of wavelength 6000Å in air enters a medium of refractive index 1.5. What will be its wavelength in the medium ?
View SolutionA ray of light travelling in air is incident on the plane surface of a transparent medium. The angle of incidence is 45∘ and the angle of refraction is 30∘ . Find the refractive index of the medium .
View Solution
