A light way deviates by 30∘ (which is one third of the angle of incidence) when it gets refracted from vacumm to a medium. Find the refractive index of the medium.
To solve the problem of finding the refractive index of a medium when a light ray deviates by 30 degrees (which is one third of the angle of incidence) while transitioning from a vacuum to that medium, we can follow these steps:
1. Identify the Given Information:
- Angle of deviation (D) = 30 degrees
- Angle of incidence (I) = 3 * D = 3 * 30 degrees = 90 degrees
2. Draw a Diagram:
- Draw a diagram showing the incident ray coming from a vacuum (air) striking the boundary of the medium. Mark the normal line at the point of incidence. The incident ray makes an angle of 90 degrees with the normal.
3. Determine the Angle of Refraction (R):
- According to the geometry of the situation, the angle of deviation (D) is the angle between the incident ray (if it had continued straight) and the refracted ray.
- Since the angle of incidence (I) is 90 degrees, we can find the angle of refraction (R) using the relationship:
I−R=D
- Substituting the known values:
90∘−R=30∘
- Solving for R:
R=90∘−30∘=60∘
4. Apply Snell's Law:
- Snell's Law states:
n1sinI=n2sinR
- Here, n1 is the refractive index of the first medium (vacuum), which is 1, I is 90 degrees, n2 is the refractive index of the medium we want to find, and R is 60 degrees.
- Substituting the known values into Snell's Law:
1⋅sin(90∘)=n2⋅sin(60∘)
- Since sin(90∘)=1 and sin(60∘)=√32:
1=n2⋅√32
5. Solve for the Refractive Index (n2):
- Rearranging the equation gives:
n2=1sin(60∘)=1√32=2√3
Final Answer:
The refractive index of the medium is:
n2=2√3
- Angle of deviation (D) = 30 degrees
- Angle of incidence (I) = 3 * D = 3 * 30 degrees = 90 degrees
- Draw a diagram showing the incident ray coming from a vacuum (air) striking the boundary of the medium. Mark the normal line at the point of incidence. The incident ray makes an angle of 90 degrees with the normal.
- According to the geometry of the situation, the angle of deviation (D) is the angle between the incident ray (if it had continued straight) and the refracted ray.
- Since the angle of incidence (I) is 90 degrees, we can find the angle of refraction (R) using the relationship:
I−R=D
- Substituting the known values:
90∘−R=30∘
- Solving for R:
R=90∘−30∘=60∘
- Snell's Law states:
n1sinI=n2sinR
- Here, n1 is the refractive index of the first medium (vacuum), which is 1, I is 90 degrees, n2 is the refractive index of the medium we want to find, and R is 60 degrees.
- Substituting the known values into Snell's Law:
1⋅sin(90∘)=n2⋅sin(60∘)
- Since sin(90∘)=1 and sin(60∘)=√32:
1=n2⋅√32
- Rearranging the equation gives:
n2=1sin(60∘)=1√32=2√3
The refractive index of the medium is:
n2=2√3
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-1141videos
GEOMATRICAL OPTICS
- BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-262videos
GEOMATRICAL OPTICS
- BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Advance level Problems35videos
GEOMATRICAL OPTICS
- BOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II10videos
EXPERIMENTAL PHYSICS
- BOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS16videos
GRAVITATION
Similar Questions
Define refractive index of a medium.
View SolutionHow is it related to the refractive index of the medium ?
View SolutionRefractive index of a medium depends on
View SolutionWhen light is refracted into a medium
View SolutionWhen light is refracted into a medium
View SolutionHow is the critical angle related to the refractive index of a medium ?
View SolutionThe wavelength of light in vacuum is 6000A∘ and in a medium it is 4000A∘ The refractive index of the medium is:
View SolutionThe speed of light slows down in a medium whose refractive index is ……….
View SolutionFigure shows a ray of light as it travels from medium A to medium B. Refractive index of the medium B relative to medium A is
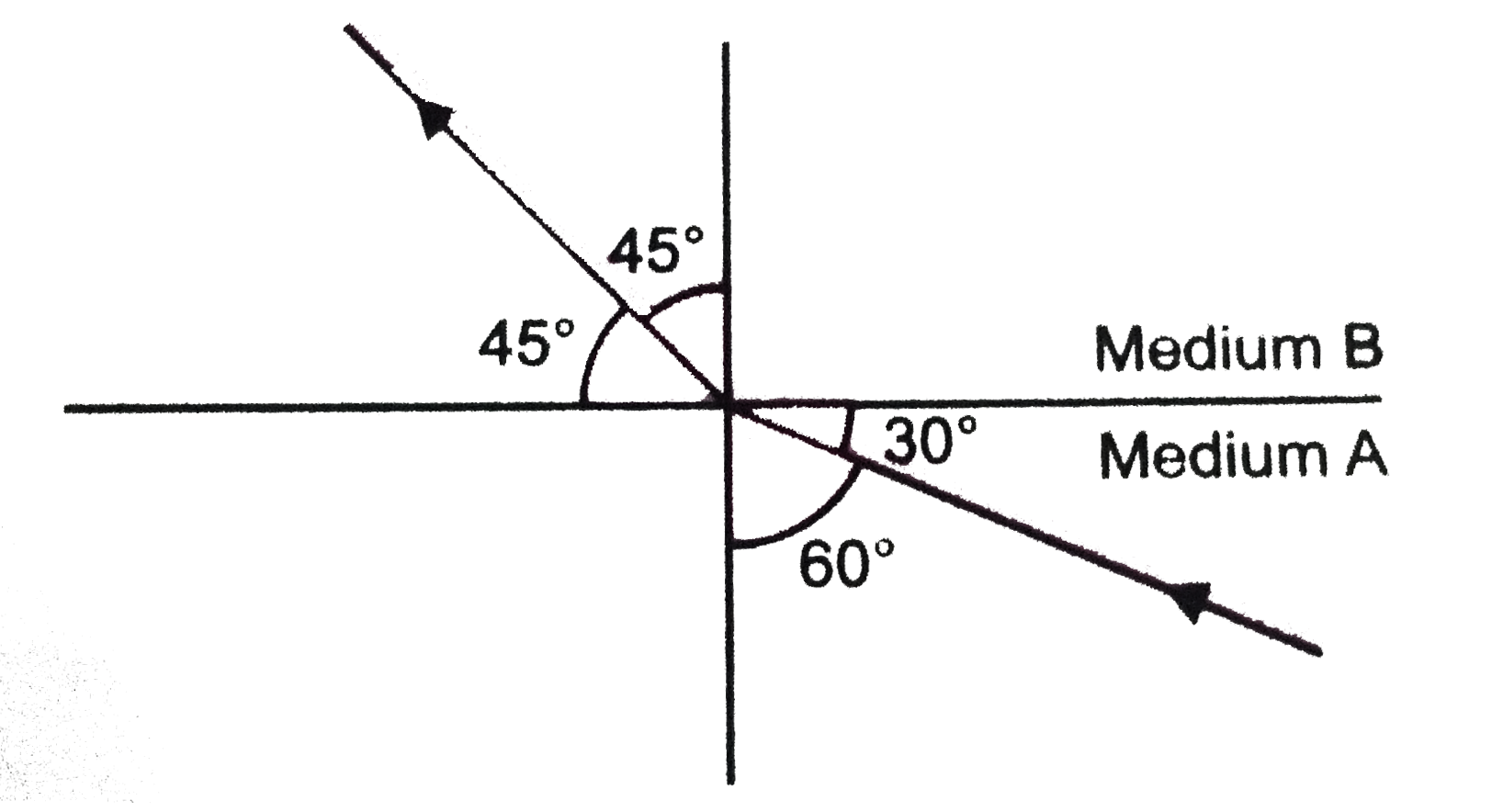 View Solution
View SolutionWhen a light ray from a rarer medium is refracted into a denser medium, its
View Solution

