A uniform horizontal beam of light is incident upon a quarter cyclinder of radius R=5cm, and has a refractive index 2/√3, A patch on the table at a distance x from the cylinder is unilluminated. Find the value of x?
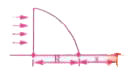
When the angle of incidence is θc x region will remain unilluminated. This is the limiting case.

When the angle of incidence is greater than critical agle TIR will place In this case, also x regio is unilluminated.
When angle of incidence θ<θc then the incident ray will refract and hence x region will be illuminated. So in limiting case:
In ΔPQR,cosθc=RR+xsinθc=μrμd=12/√3=√32
∴θc=60∘,R=5cm (given)
⇒cos60=55+x⇒5+x=10⇒x=5cm
Topper's Solved these Questions
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE I
95 Videos
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE 1B
45 Videos
- PHYSICAL WORLDBOOK - AAKASH SERIESCHAPTER - PHYSICAL WORLDEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)
6 Videos
Similar Questions
A uniform,horizontal beam of light is incident upon a quarter cylinder of radiusR=5cm and has a refractive index 2/√3.A patch on the table for a distance 'x'from the cylinder is unilluminated ,find the value of 'x'?
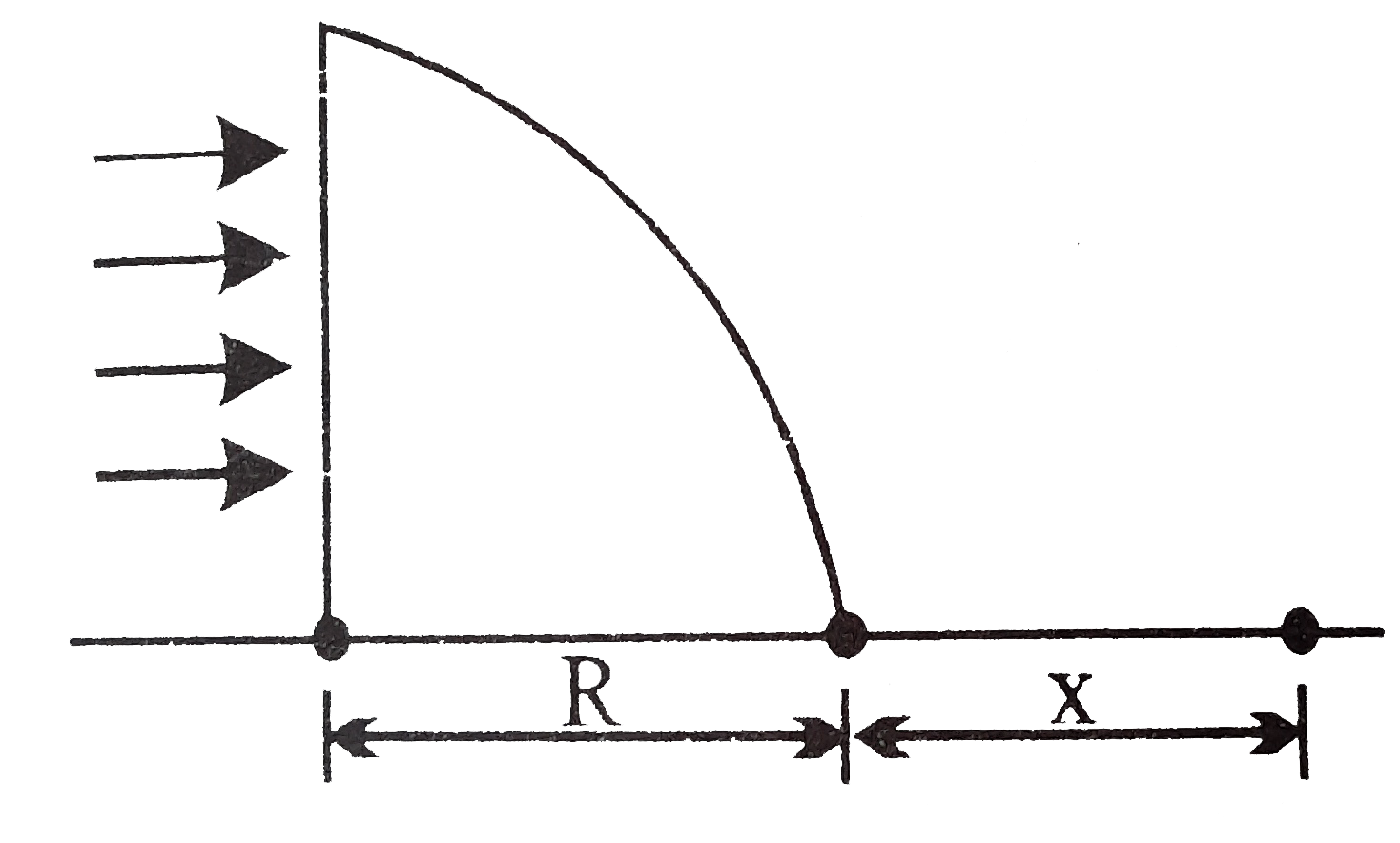
View SolutionA uniform,horizontal beam of light is incident upon a quarter cylinder of radiusR=5cm and has a refractive index 2/√3.A patch on the table for a distance 'x'from the cylinder is unilluminated ,find the value of 'x'?

View Solution
Knowledge Check
As shown, a narrow beam of light is incident onto a semi-circular glass cylinder of radius R. Light can exit the cylinder when the beam is at the centre. When the beam is moved parallel to a maximum distance d from the central line, no light can exit the cylinder from its lower surface. Find the refractive index of the glass.
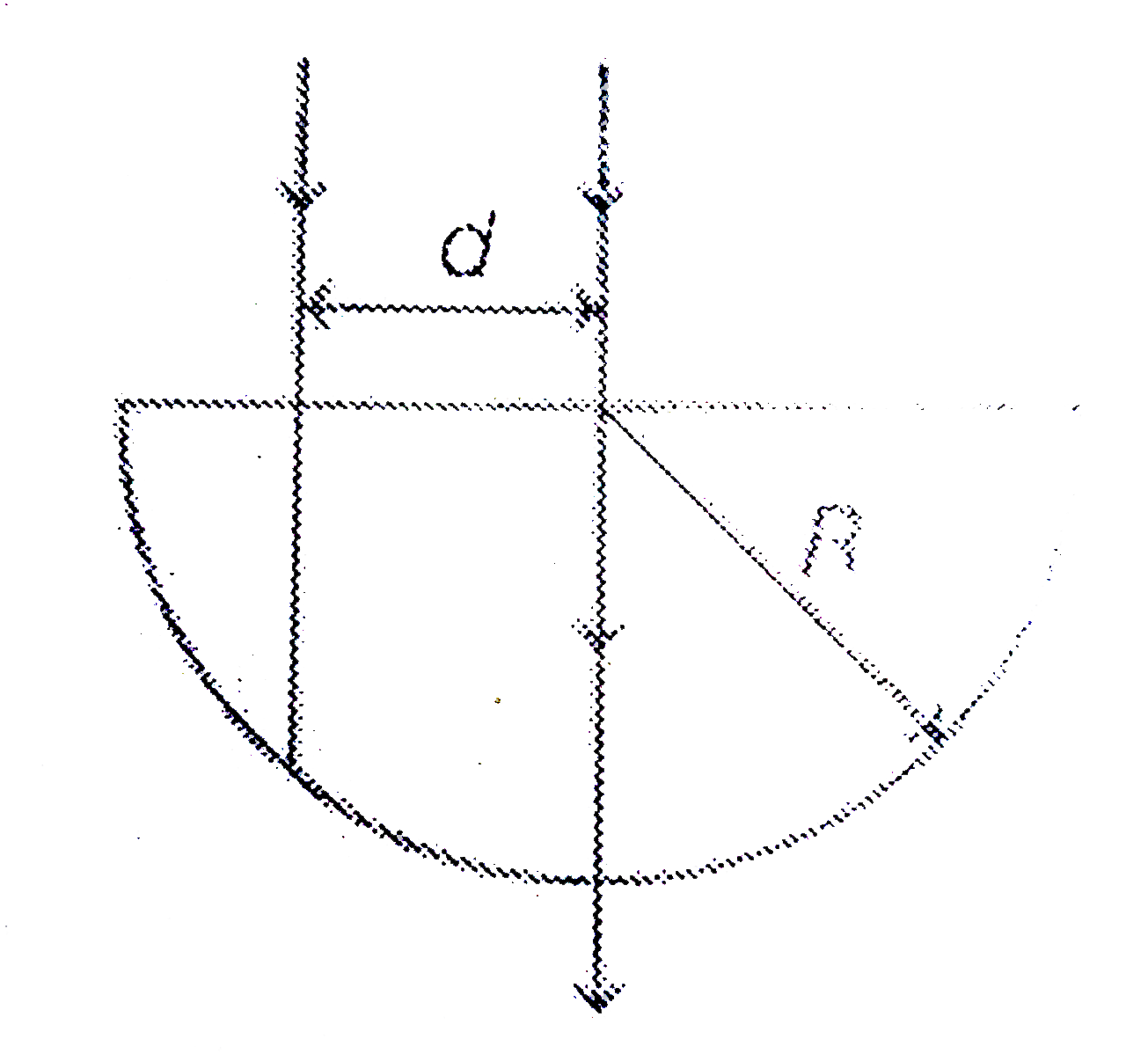 ARdBdRCR√R2−d2D√R2−d2R
ARdBdRCR√R2−d2D√R2−d2R
A uniform, horizontal parallel beam of light is incident upon a prism as shown. The prism is in the shape of a quarter cylinder, of radius 5cm, and has refractive index 5/3. The width of the regionat which the incident rays after normal incidence on plane surface and subsequent refraction at curved surface intersect on x axis is (Neglect the ray which travels along x-axis)
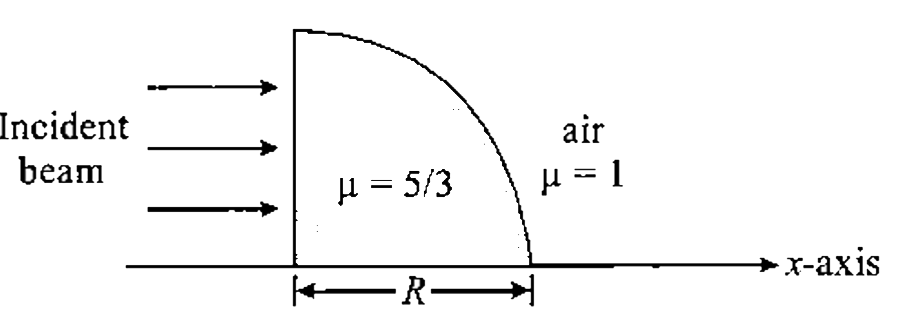
View SolutionA horizontal ray of light is incident on a solid glass sphere of radius R and refractive index μ . What is net deviation of the beam when it emerged from the other side of the sphere?
View SolutionA horizontal ray of light is incident on a solid glass sphere of radius R and refractive index μ . What is net deviation of the beam when it emerged from the other side of the sphere?
View SolutionA light beam of diameter √3R is incident symmetrically on a glass hemispher of radius R and of refractive index n=√3 . Find radius of the beam at the base of hemispher.
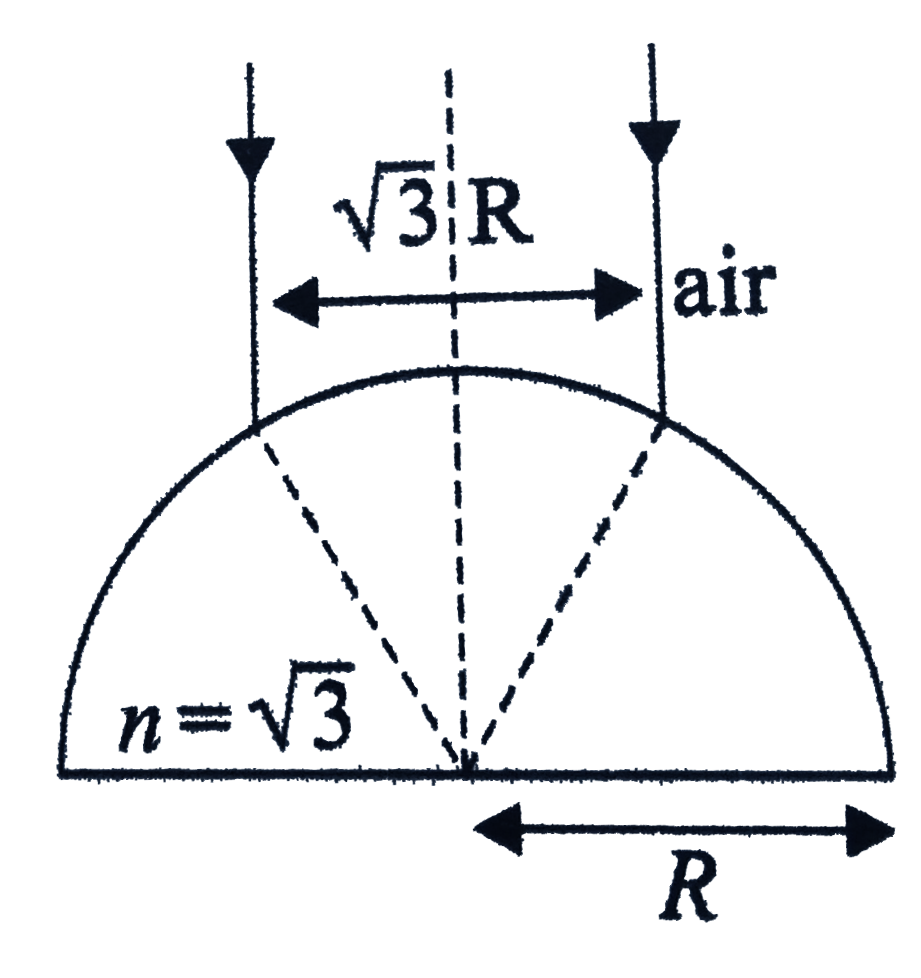
View SolutionA light beam of diameter √3R is incident symmetrically on a glass hemispher of radius R and of refractive index n=√3 . Find radius of the beam at the base of hemispher.

View SolutionA light beam of diameter √3R is incident symmetrically on a glass hemisphere of radius R and of refractive index n=√3 . Find radius of the beam at the base of hemisphere.

View SolutionA narrow parallel beam of light falls on a glass sphere of radius R and refractive index μ at normal incidence. The distance of the image from the outer edge is given by-
View Solution
