a block of mass 0.1 kg attached to a spring of spring constant400Nm pulled horizontally from x=0 tox1=10mm. Find the work done by the spring force
2⋅10−2J
2⋅10−6J
3⋅10−6J
4⋅10−2J
The correct Answer is:A
To find the work done by the spring force when a block is pulled from x=0 to x1=10 mm, we can use the formula for the work done by a spring:
1. Identify the Given Values:
- Mass of the block, m=0.1kg
- Spring constant, k=400N/m
- Displacement, x1=10mm=10×10−3m=0.01m
2. Understand the Work Done by the Spring:
The work done by the spring force when it is stretched or compressed is given by the formula:
W=−12kx2
Here, the negative sign indicates that the work done by the spring force is in the opposite direction of the displacement.
3. Calculate the Work Done:
Substitute the values into the formula:
W=−12×400N/m×(0.01m)2
W=−12×400×0.0001
W=−12×0.04
W=−0.02J
4. Final Result:
The work done by the spring force when the block is pulled from x=0 to x=10 mm is:
W=−0.02JorW=−2×10−2J
- Mass of the block, m=0.1kg
- Spring constant, k=400N/m
- Displacement, x1=10mm=10×10−3m=0.01m
The work done by the spring force when it is stretched or compressed is given by the formula:
W=−12kx2
Here, the negative sign indicates that the work done by the spring force is in the opposite direction of the displacement.
Substitute the values into the formula:
W=−12×400N/m×(0.01m)2
W=−12×400×0.0001
W=−12×0.04
W=−0.02J
The work done by the spring force when the block is pulled from x=0 to x=10 mm is:
W=−0.02JorW=−2×10−2J
Topper's Solved these Questions
Similar Questions
A block of mass 0.1 kg attached to a spring of spring constant 400 N/m is pulled rightward from x0=0 to x1=15 mm. Find the work done by spring force.
View SolutionAn external unknown horizontal force F pulls a smooth block of mass m attached with an ideal spring of stiffness k. Block is initially at rest and spring is relaxed. Find the work done (in J) by the force F, when the block moves with a velocity v and the deformation of the spring is x (k = 25 N/m, v = 1 m/s, m = 1 kg, x =20 cm)
View Solution
Knowledge Check
A block of mass 1kg is connected with a massless spring of force constant 100N/m. At t=0, a constant force F=10N is applied on the block. The spring is in its netural length at t=0. The speed of particel at x=6cm form mean position is
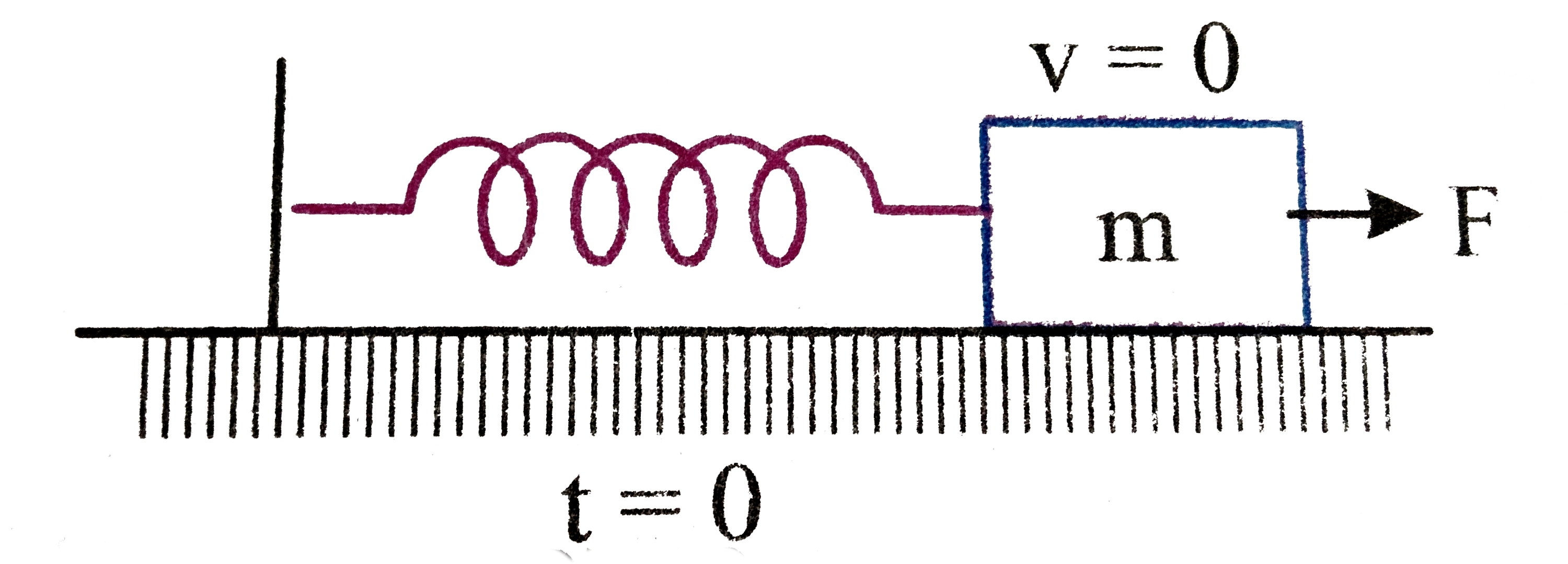 A4cm/sB10cm/sC80cm/sD50cm/s
A4cm/sB10cm/sC80cm/sD50cm/sA spring with spring constant k is extended from x=0 to x=x1. The work done will be
Akx21B12kx21C2kx21D2kx1A block of mass 1 kg is attached to one end of a spring of force constant k = 20 N/m. The other end of the spring is attached to a fixed rigid support. This spring block system is made to oscillate on a rough horizontal surface (μ=0.04). The initial displacement of the block from the equilibrium position is a = 30 cm. How many times the block passes from the mean position before coming to rest ? (g=10m/s2)
A11B7C6D15
A spring of spring constasnt 50 N/m is compressed from its natural position through 1 cm. Find the work done by the work done by the spring force on the agency compressing the spring.
View SolutionA small block of mass 2 kg is placed on a bigger block of mass 4 kg which is attached to horizontal spring of spring constant K=500N/m as shown in figure, coefficient of friction between block is 0.2 .Maximum amplitude of system so that there is no relative slipping between blocks.
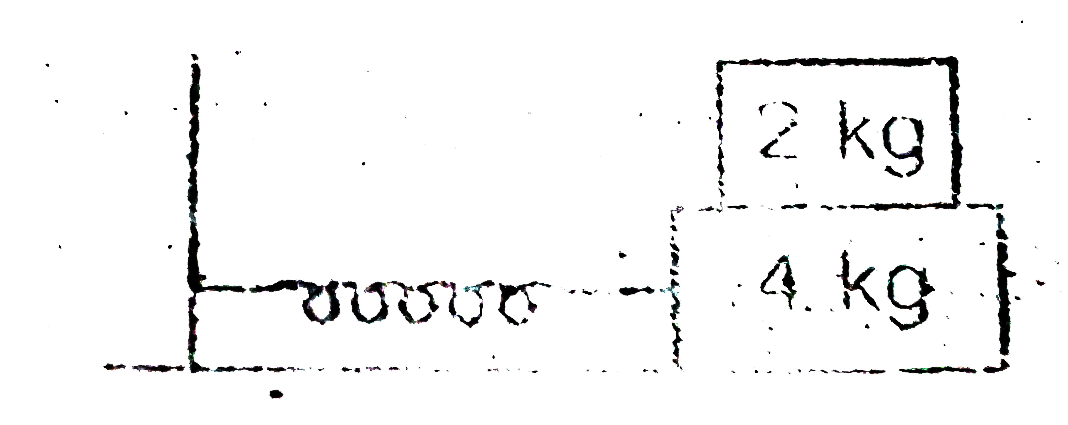
View SolutionA block of mass 0.2 kg is attached to a mass less spring of force constant 80 N/m as shown in figure. Find the period of oscillation. Take g=10m/s2. Neglect friction

View SolutionOne end of a fixed spring is pulled by an average force of 10N through a distance of 5cm, find the work done by the spring.
View SolutionA block whose mass is 2 kg is fastened on a spring whose spring constant is 100 Nm−1 . It is pulled to a distance of 0.1 m from over a frictionless surface and is released at t=0. Calculate the kinetic eneryg of the block when it is 0.05 m away from its mean position.
View Solution
