The path length difference between two waves coming from coherent sources for destructive interference should be
Zero
(2n)λ
(2n−1)λ3
(2n+1)λ2
The correct Answer is:D
To determine the path length difference between two waves coming from coherent sources for destructive interference, we can follow these steps:
1. Understanding Interference:
- Interference occurs when two coherent waves meet. Coherent waves are waves that have a constant phase difference and the same frequency.
2. Types of Interference:
- There are two types of interference: constructive and destructive.
- Constructive Interference occurs when the path length difference between the two waves is an integer multiple of the wavelength (nλ, where n is an integer).
- Destructive Interference occurs when the path length difference is an odd multiple of half the wavelength.
3. Condition for Destructive Interference:
- For destructive interference, the path length difference (Δx) must satisfy the condition:
Δx=(n+12)λ
- Here, n is an integer (0, 1, 2, ...), and λ is the wavelength of the waves.
4. Expressing the Condition:
- This can also be expressed as:
Δx=(2n+1)2λ
- This indicates that the path length difference must be an odd multiple of half the wavelength.
5. Analyzing the Options:
- If we analyze the options provided, we are looking for a value that matches the form of (2n+1)2λ.
- The options include values like 0, λ, λ2, and λ3.
6. Selecting the Correct Option:
- The only option that represents an odd multiple of half the wavelength is λ2 when n=0.
- Therefore, the correct answer for the path length difference for destructive interference is:
Δx=λ2 (for n=0)
Final Answer:
The path length difference between two waves coming from coherent sources for destructive interference should be λ2.
---
- Interference occurs when two coherent waves meet. Coherent waves are waves that have a constant phase difference and the same frequency.
- There are two types of interference: constructive and destructive.
- Constructive Interference occurs when the path length difference between the two waves is an integer multiple of the wavelength (nλ, where n is an integer).
- Destructive Interference occurs when the path length difference is an odd multiple of half the wavelength.
- For destructive interference, the path length difference (Δx) must satisfy the condition:
Δx=(n+12)λ
- Here, n is an integer (0, 1, 2, ...), and λ is the wavelength of the waves.
- This can also be expressed as:
Δx=(2n+1)2λ
- This indicates that the path length difference must be an odd multiple of half the wavelength.
- If we analyze the options provided, we are looking for a value that matches the form of (2n+1)2λ.
- The options include values like 0, λ, λ2, and λ3.
- The only option that represents an odd multiple of half the wavelength is λ2 when n=0.
- Therefore, the correct answer for the path length difference for destructive interference is:
Δx=λ2 (for n=0)
The path length difference between two waves coming from coherent sources for destructive interference should be λ2.
Topper's Solved these Questions
Similar Questions
Write the relationship between the path difference and the wavelength of light used for constructive and destructive interference of light.
View SolutionIn experimental set up of interference the twol interfering sources S1andS2 have an initial phase difference corresponding to a path difference of λ/4. The phase difference due to path difference between the twoll interfering waves at a point of constructive and destructive interference respectively is
View Solution
Knowledge Check
The path difference between two waves is
AxDdBxdDCxDλDxλdInterference is an important property of light. In the given table, Column I shows the properties of interfering waves, Column II shows the phase difference, and Column III shows the path difference between two interfering waves
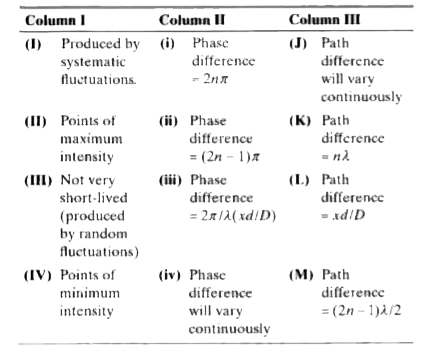
What are the conditions for destructive interference?A(I) (ii) (J)B(IV) (ii) (M)C(II) (iii) (L)D(I) (i) (M)What is the path difference of destructive interference
A(a) nλB(b) n(λ+1)C(c) (n+1)λ2D(d) (2n+1)λ2
Path difference between two wavefronts emitted from coherent sources is 2.1μm. Phase difference between the wavefronts at that point is 7.692 πthe wavelength of light emitted by source will be
View SolutionIn X-ray diffraction experiment at which one of the following path difference between the two waves , destructive interference is observed (γ=wavelength of x-rays)
View SolutionIn x-ray diffraction experiment at which one of the following path difference between the two waves, destructive interference is observed ( λ = wavelength of x-rays)
View SolutionWhich of the following is the path difference for destructive interference ?
View SolutionPath difference between two wavefronts emitted from coherent sources is 2.1μm. Phase difference between the wavefronts at that point is 7.692 πthe wavelength of light emitted by source will be
View Solution
