Derive an expression for the induced emf and induced current produced by changing the area of a rectangular coil placed perpendicular to a uniform magnetic field.

Text Solution

Verified by Experts

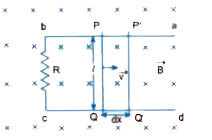Consider a U-shaped conductor abcd lying normal to a uniform magnetic field $\vec{B}$ . Let a conductor rod PQ is placed on frame abed and let it be moving with a uniform velocity $\vec{v}$ . As the rod moves by a small distance dx = v dt, the area enclosed changes from QcbP to Qʻcbp.
Thus, change in magnetic flux
$d f_{B} = B (d A) = B [a r e a Q c b P \to Q . c b P .]$
= B (area Q.QPP.) = B l dx = B lvdt
$∴$ magnitude for induced emf $| ɛ | = \frac{d ϕ_{B}}{d t} = \frac{B l v d t}{d t} = B l v$
If R be the resistang of the loop then induced current $| I | = \frac{| ɛ |}{R} = \frac{B l v}{R} .$

Updated on:21/07/2023

An emf induced in a coil rotating in a uniform magnetic field is given by
A $e = e_{0} sin ω t$
B $e_{0} = e sin ω t$
C $e = sin ω t$
D $e = e_{0} sin ω$
Production of induced emf in a coil due to the changes of current in the same coil is
Aself induction
Bmutual induction
Cdynamo
Dnone of these
Mutual induction is the produced of induced emf in a coil due to the change of current in the
Asame coil
Bneighbouring coil
Cboth a and b
Dneigher a nor b