Let ABCbe an isosceles triangle inscribed ina circle having radius r. Then by figure area of the triangle ABC is △
Find d△dθ and d2△dθ2.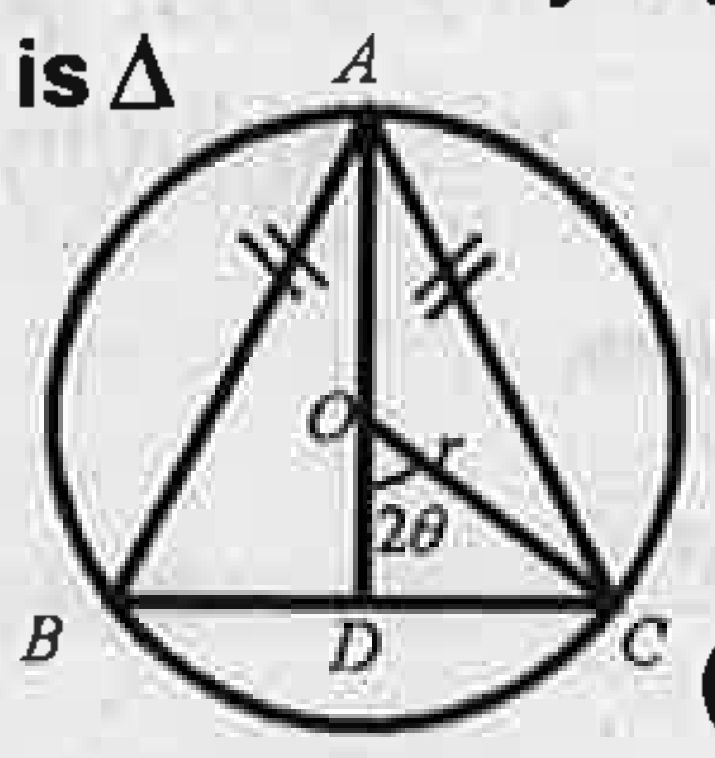 
Solution in Malayalam
Step by step video & image solution for Let ABCbe an isosceles triangle inscribed ina circle having radius r. Then by figure area of the triangle ABC is triangle Find (d triangle)/(d theta) and (d^2 triangle)/(d theta^2). by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
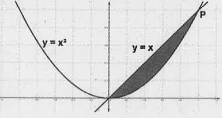
Consider the following figure
find the point of intersection (P) of the parabola and the line.
View SolutionletABC be a right angled triangle at C.If the inscribed circle touches the side ABat D and (AD)(BD)=11, then find the area of triangle ABC..
View SolutionAn isosceles triangle of vertical angle 2θ is inscribed in a circle of radius a . Show that the area of the triangle is maximum when θ=π6 .
View SolutionIn the given figure (not to scale), O is the centre of the circle. A,B,C and D are concyclic and AB=CD . If /_MON =120^(@) , then find /_ OPN .
View SolutionIn the following figure, AB and BC are equidistant from the centre 'O' of the Show that
(a) ABC is an isosceles triangle.
(b) OB bisects angle ABC.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_X_C13_E02_006_Q01.png" width="80%"gt
View SolutionIn the following figure ,ABCD is a parallelogram, CB is extended to F and the line joining D and F intersect AB at E. Then prove that ΔEAD~ΔEBF
View SolutionMatch the following Column A to Column B
View SolutionIn the given figure, DEF is a triangle. If DF is the longest side and EF is the shortest side, then which of the following is true?
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C12_E04_028_Q01.png" width="80%"gt
View SolutionIn the following figure (not to scale), ABC is a right isosceles triangle, right- angle at B and BD⊥AC. If the triangle ABC is rotated about the hypotenuse, then find the locus of the triangle ABC. ltbr gt ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C15_E02_006_Q01.png" width="80%"gt
View SolutionFind out area of triangle OAB and BCD shown in figure :- ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/ALN_PHY_R05_E01_014_Q01.png" width="80%"gt
View SolutionAn isosceles triangle of vertical angle 2θ is inscribed in a circle of radius a . Show that the area of the triangle is maximum when θ=π6 .
View SolutionLet x and y be the length and breadth of the rectangle ABCD in a circle having radius r. Let ∠CAB=θ (Ref. Figure). If △ represent area of the rectangle and r is a constant.
Find d△dθ and d2△dθ2.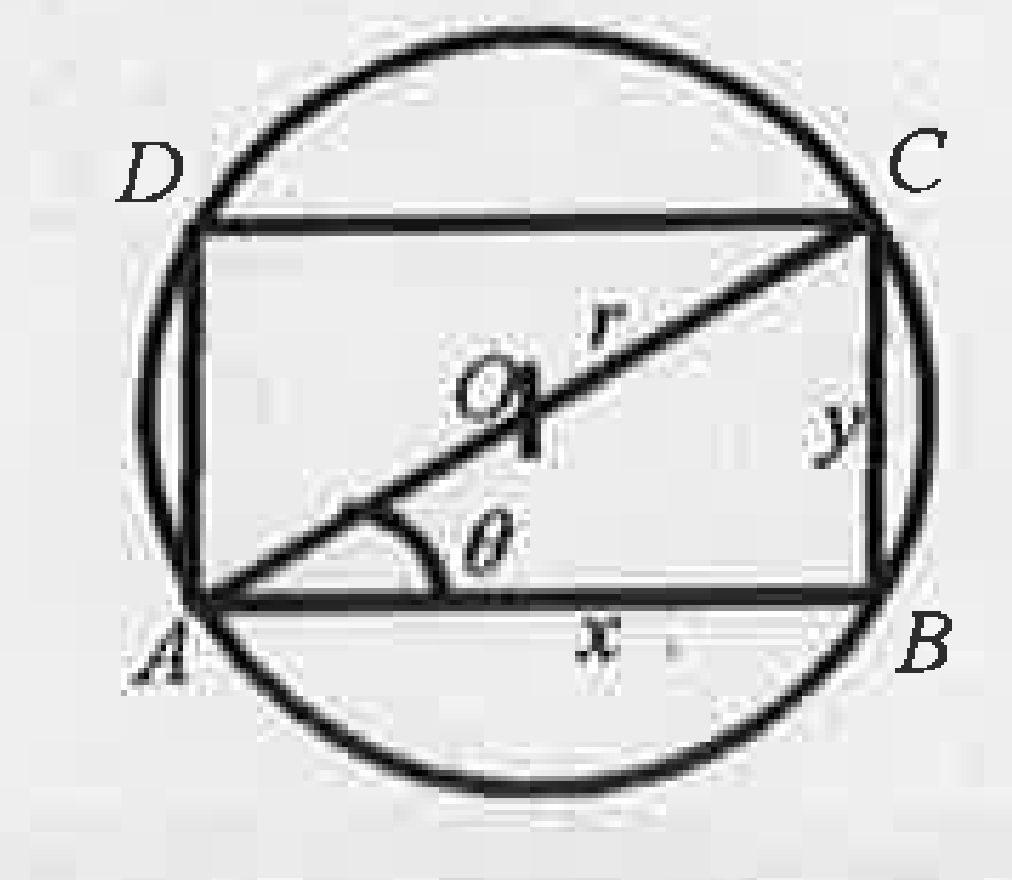
View SolutionletABC be a right angled triangle at C.If the inscribed circle touches the side ABat D and (AD)(BD)=11, then find the area of triangle ABC..
View SolutionAn isosceles triangle of vertical angle 2θ is inscribed in a circle of radius a. The area of the triangle is maximum, if θ=
View SolutionletABC be a right angled triangle at C.If the inscribed circle touches the side ABat D and (AD)(BD)=11, then find the area of triangle ABC..
View Solution