एक फलन y=f(x),x=11+t2 तथा y=1t(1+t2)∀t>0 के द्वारा दिया जाता है, तब f होगा -
(0,3/2) में वर्धमान तथा (3/2,∞) में ओसमान
(0,1) में वर्धमान
(0,∞) में वर्धमान
(0,1) में ओसमान
The correct Answer is:B
Step by step video, text & image solution for एक फलन y= f(x) , x= (1)/( 1+ t^2) तथा y= (1)/( t(1+ t^2) ) AA t gt 0 के द्वारा दिया जाता है, तब f होगा - by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MONOTONOCITYBook:ALLEN HINDIChapter:MONOTONOCITYExercise:EXERCISE - 03 MISCELLANEOUS TYPE QUESTIONS ( स्तम्भ सुमेलन (Match the column) )
Explore 1 Video
- MONOTONOCITYBook:ALLEN HINDIChapter:MONOTONOCITYExercise:EXERCISE - 03 MISCELLANEOUS TYPE QUESTIONS कथन एवं कारण (Assertion & Reason)
Explore 3 Videos
- MONOTONOCITYBook:ALLEN HINDIChapter:MONOTONOCITYExercise:EXERCISE - 01 (सही विकल्पों को चुनिए (एक या एक से अधिक सही उत्तर हैं))
Explore 5 Videos
- METHODS OF DIFFERENTIATIONBook:ALLEN HINDIChapter:METHODS OF DIFFERENTIATIONExercise:EXERCISE - 05 [B]
Explore 12 Videos
- PARABOLA Book:ALLEN HINDIChapter:PARABOLA Exercise:DO YOURSELF - 7
Explore 2 Videos
Similar Questions
निम्न प्रश्न में दो स्तम्भ में वक्तव्य (statements) दिये हुए हैं जिनका सुमेल (match) करना है।स्तम्भ-I (Column-I) के वक्तव्यों को A,B,C तथा D नामित किया गया है जबकि स्तम्भ-II (Column-II) के वक्तव्यों को p,q,r तथा s नामित किया गया है। स्तम्भ-I (Column-I) में दिए गए कोई एक वक्तव्यस्तम्भ-II (Column-II) के एक या एक से अधिक वक्तव्य (वक्तव्यों) से सही सुमेल करता है।
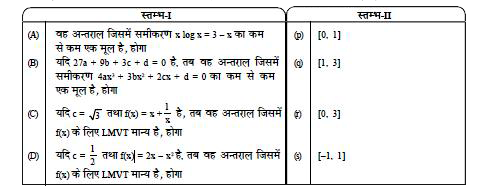
View Solutionनिम्न प्रश्नों में दो कथन दिए गए है, एक को कथन - I तथा दूसरे को कथन - II के रूप में अंकित किया गया है।
(A) कथन-I सत्य है, कथन-II सत्य है, कथन-II, कथन-I का सही स्पष्टीकरण है।
(B) कथन- सत्य है, कथन-II सत्य है , कथन-II, कथन-I का सही स्पष्टीकरण नहीं है।
(C) कथन - I सत्य है परन्तु कथन - II असत्य है।
(D) कथन - I असत्य है परन्तु कथन - II सत्य है।
कथन-I: द्विघात समीकरण 10x2−28x+17=0 का कम से कम एक मूल [1,2] में होगा।
क्योंकि
कथन-II: f(x)=e10x(x−1)(x−2),[1,2] में रोल प्रमेय के सभी प्रतिबन्धों को सन्तुष्ट करता है।
View Solutionमाना h(x)=f(x)−(f(x))2+(f(x))3,∀x∈R हो, तो -
View Solutionयदि ln (x+y) = 2xy हो , तो y'(0) =
View Solutionरेखाओं x=4,y=4 तथा निर्देशी अक्षो से बने वर्गीय क्षेत्र को परवलय y2=4x तथा x2=4y विभाजित करते है। यदि S1,S2,S3 क्रमशः इन भागो के क्षेत्रफल ऊपर से नीचे की ओर अंकित है, तो S1:S2:S3 ज्ञात कीजिए।
View SolutionDiscuss monotonocity of y=f(x) which is griven by x=11+t2andy=1t(1+t2)
View SolutionIff(y)=ey,g(y)>0,andF(t)=∫t0t(t−y)dt,then F(t)=et−(1+t) F(t)=tet F(t)=te−1 (d) F(t)=1−et(1+t)
View SolutionA function y=f(x) is given by x=(1)/(1+t^(2)) and y=(1)/(t(1+t^(2))) for all t>0 then f is
View SolutionIf a function y=f(x) is defined as y=1t2−t−6andt=1x−2,t∈R. Then f(x) is discontinuous at
View Solutionदर्शाइए की बिंदु x=2at1+t2 तथा y=a(1−t2)1+t2 जहाँ a स्थिर राशि है t प्राचल है तथा −1≤t≤1,t के प्रत्येक मान के लिए वृत्त पर स्थित है ।
View SolutionLet f(t)=|t−1|−|t|+|t+1|,∀t∈R. Find g(x)=max{f(t):x+1≤t≤x+2},∀x∈R. Find g(x) and the area bounded by the curve y=g(x), the X-axis and the lines x=−3/2andx=5.
View SolutionIf x=logt, t>0 and y=1t, then d2ydx2, is
View SolutionFor all t>0,f(x)=t2−1t−1−t . Which of the following is true about f(t) ?
View SolutionLet f(x) be differntiable on the interval (0,oo) such that f(1)=1 and lim_(t tox)(t^(2)f(x)-x^(2)f(t))/(t-x)=1 for each xgt0 . Then f(x) is :
View SolutionLet g(x)=∫x0f(t).dt,where f is such that 12≤f(t)≤1 for t∈[0,1] and 0≤f(t)≤12 for t∈[1,2].Then g(2) satisfies the inequality
View Solution
