A pendulum of mass m hangs from a support fixed to a trolley. The direction of the string (i.e., angle θ) when the trolley rolls up a plane of inclination α with acceleration 'a' is
Zero
tan−1α
tan−1(a+gsinα)gcosα
tan−1ag
The correct Answer is:C
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
108 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - I
158 Videos
- KINETIC THEORY OF GASESBOOK - AAKASH SERIESCHAPTER - KINETIC THEORY OF GASESEXERCISE - Practice Exercise
15 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-3
43 Videos
Similar Questions
A pendulum of mass m hangs from a support fixed to a trolley. The direction of the string when the trolley rolls up a plane of inclination α with acceleration a0 is
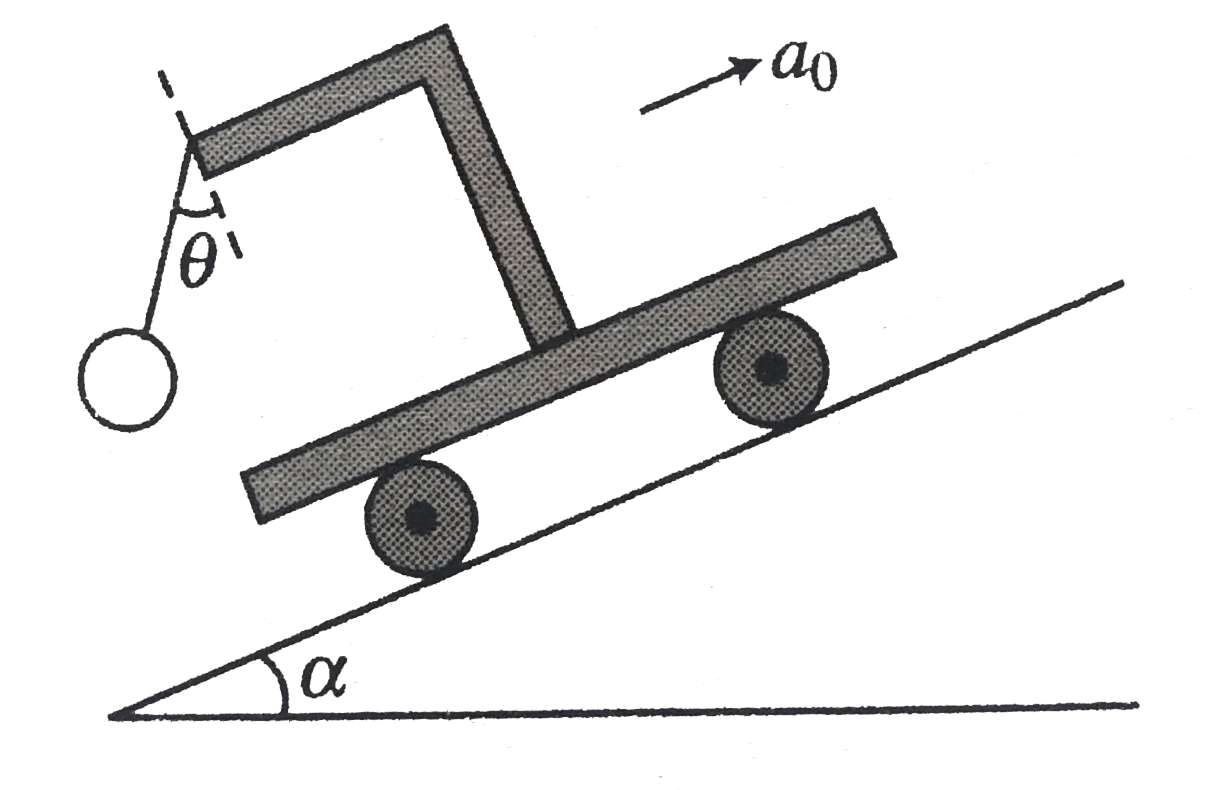
View SolutionA pendulum of mass m hangs from a support fixed to a trolley. The direction of the string when the trolley rolls up a plane of inclination α with acceleration a0 is

View Solution
Knowledge Check
A pendulum of mass m hangs from a support fixed to a trolley. The direction of the string when the trolley rolls up a plane of inclination α with acceleration a0 is
 Aθ=tan−1αBθ=tan1(a0g)Cθ=tan1(ga0)Dθ=tan1(a0+gsinαgcosα)
Aθ=tan−1αBθ=tan1(a0g)Cθ=tan1(ga0)Dθ=tan1(a0+gsinαgcosα)A pendulum of mass m hangs from a support fixed to a trolley which is moving up to the inclined plane with uniform acceleration 'a'. The angle which the string makes with vertical in equilibrium is
 A0Btan−1αCtan−1(a+gsinαgcosα)Dtan−1(acosαg+asinα)
A0Btan−1αCtan−1(a+gsinαgcosα)Dtan−1(acosαg+asinα)A pendulum has a ball of mass m attached to the stringa and is suspended from the roof of a trolley. If the trolley rolls upwards with acceleration a, then what is the angle made by the string with the inclined plane? (Given that, a=5m/s2,g=10m/s2 and the angle of inclination of the plane is 30∘)
Acos−1(2√3)Bcot−1(2√3)Csin−1(2√3)Dtan−1(2√3)
A pendulum of mass m hangs from a support fixed to a trolley which is moving up to the inclined plane with uniform acceleration 'a'. The angle which the string makes with vertical in equilibrium is

View SolutionA pendulum is hanging from the ceiling of a trolley which moves down a smooth inclined plane. Then
View SolutionA block of mass m is released from the top of fixed inclined smooth plane. if theta is the angle of inclination then vertical accelertion of block is
View SolutionA rolling object rolls without slipping down an inclined plane (angle of inclination θ), then the minimum acceleration it can have is ?
View SolutionA rolling object rolls without slipping down an inclined plane (angle of inclination θ), then the minimum acceleration it can have is.
 .
.
View Solution
