(A) : The angular momentum of a particle w.r.t origin moving parallel to x-axis with constant velocity is constant
(R) : There is no change in the perpendicular distance of the particle from the origin when it travels parallel to x-axis.
Both 'A' and 'R' are true 'R' is the correct explanation of 'A'
Both 'A' and 'R' are true 'R' is not the correct explanation of 'A'
A' is true and 'R' is false
A' is false and 'R' are true
The correct Answer is:A
Topper's Solved these Questions
- ROTATIONAL DYNAMICS BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - LEVEL - II LECTURE SHEET (ADVANCED) More than One correct answer Type Questions
14 Videos
- ROTATIONAL DYNAMICS BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - LEVEL - II LECTURE SHEET (ADVANCED) Linked comprehension Type Questions
6 Videos
- ROTATIONAL DYNAMICS BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - PRACTICE SHEET (Exercise - VI) (LEVEL - II Matrix Matching Type Questions)
3 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)
6 Videos
- ROTATIONAL MECHANICSBOOK - AAKASH SERIESCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for Descriptive Answers
20 Videos
Similar Questions
Knowledge Check

A particle mass parallel to x-axis with constant velocity v as shown in the figure. The angular velocity of the particle about the origin OAremains constantBcontinuously increasesCcontinuously decreasesDoscillates.A particle is moving along a straight line parallel to x-axis with constant velocity. Its angular momentum about the origin
Adecreases with timeBincreases with timeCremains constantDis zeroA particle is moving parallel to x-axis as shown in the figure. The angular velocity of the particle about the origin is
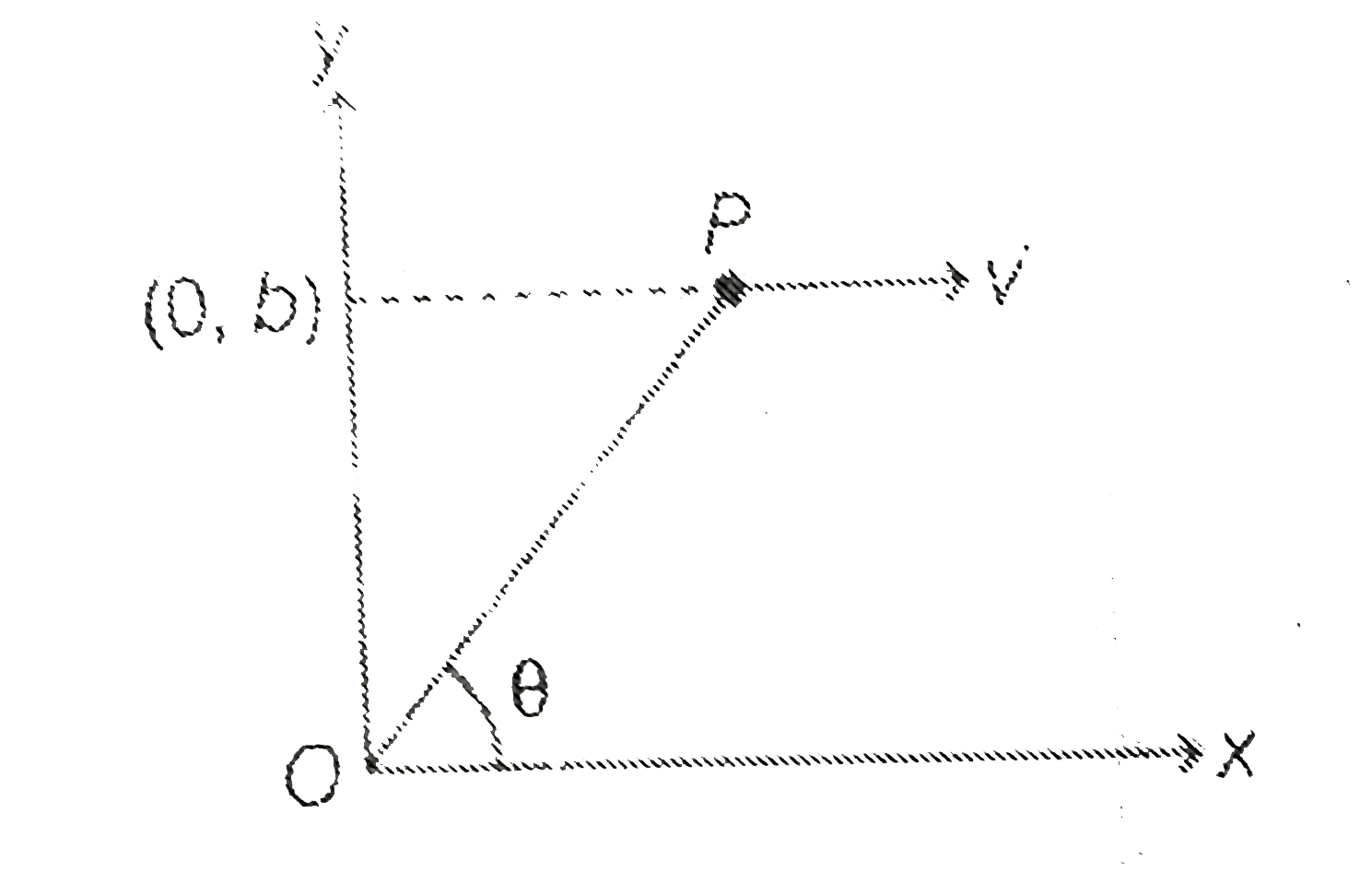 A(v/b)sin2θB(v/b)C(v/b)sinθDvbsinθ
A(v/b)sin2θB(v/b)C(v/b)sinθDvbsinθ
A particle is moving along a straight line parallel to x-axis with constant velocity. Find angular momentum about the origin in vector form :

View SolutionA particle is moving along a straight line parallel to x-axis with constant velocity. Find angular momentum about the origin in vector form :

View SolutionA particle is moving along a stright line parallel to x-axis with constant velocity. Does its angular momentum about the origin decrease with time or increase with time or remain constant ?
View SolutionA particle moving parallel to x-axis as shown in fig. such that at all instant the y-axis component of its position vector is constant and is equal to 'b'. Find the angular velocity of the particle about the origin when its radius vector makes angle θ from the axis.
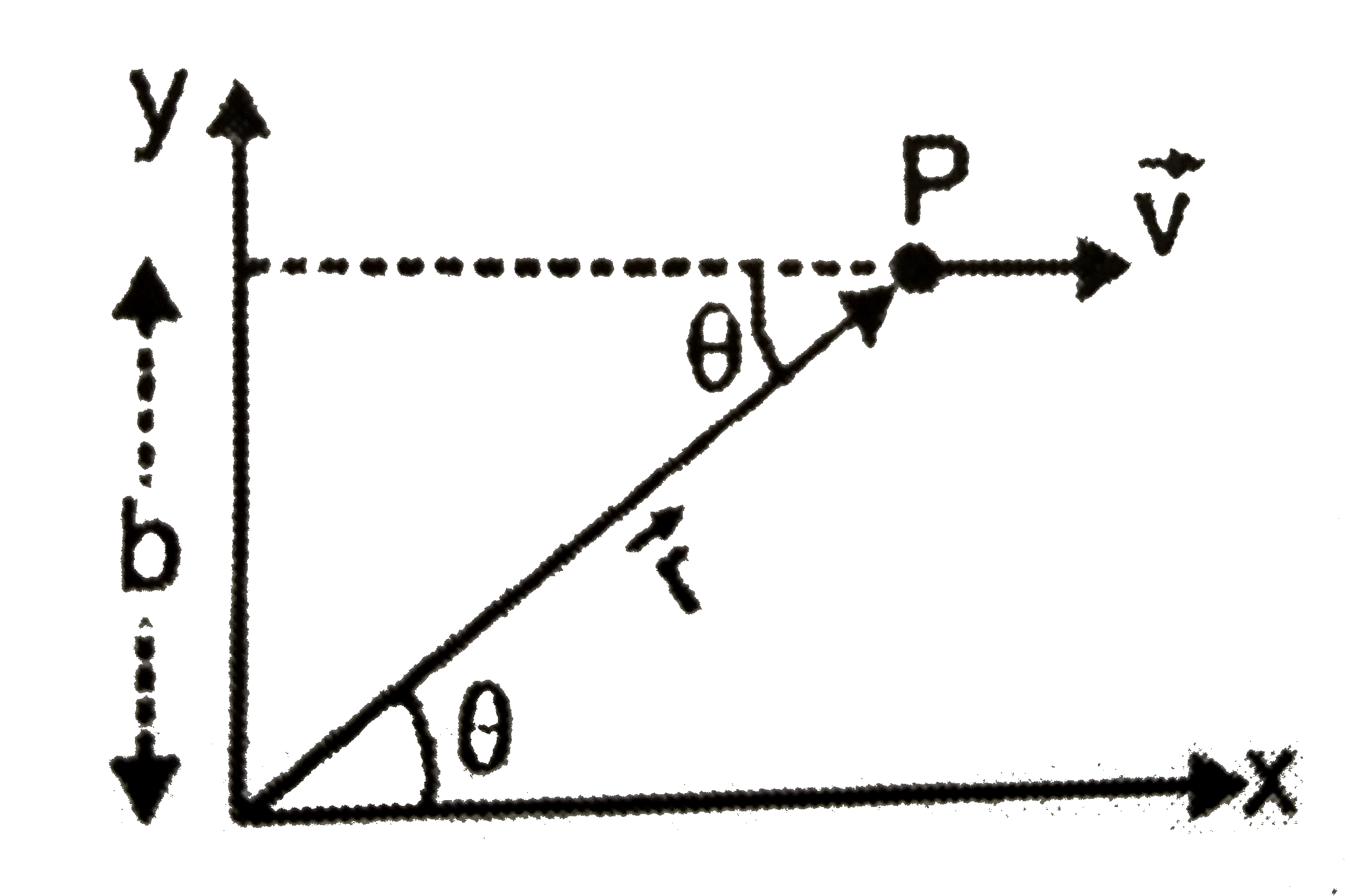
View SolutionA particle is moving in the xy-plane with a constant velocity along a line parallel to the X-axis away from the origin. The magnitude of its angular momentum about the origin.
View Solution
