Check whether the first polynomial is a factor of the second polynomial by applying the division algorithm : g(x)=3x−2,p(x)=3x3+x2−20x+12 .Solution in Punjabi
Step by step video & image solution for Check whether the first polynomial is a factor of the second polynomial by applying the division algorithm : g(x)=3x-2,p(x)=3x^3+x^2-20x+12 . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The graphs of y = p (x) are given in figure below, for some polynomials p (x). Find the number of zeroes of p (x), in each case.
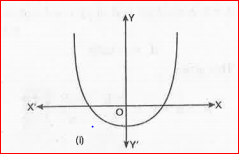 .
.
View Solution4 chairs and 3 tables cost ? 2100 and 5 chairs and 2 tables cost ? 1750. Represent this situation algebraically and graphically.
View SolutionIf a die is thrown, what is the probability that an 'ace' turns up ?
View SolutionCheck whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial :x3−3x+1,x5−4x3+x2+3x+1
View Solutionविभाजन एल्गोरिथ्म का प्रयोग करके यह जाँच करें कि क्या प्रथम बहुपद, दूसरे बहुपद का एक गुणनखंड है ?
x−2,x3+3x2−12x+4
View Solutionविभाजन एल्गोरिथ्म का प्रयोग करके यह जाँच करें कि क्या प्रथम बहुपद, दूसरे बहुपद का एक गुणनखंड है ?
x2−4x+3,x3−3x2−x+3
View Solutionभाग एल्गोरिदम के प्रयोग से जाँचें कि क्या बहुपद x2+3x+1, बहुपद 3x4+5x3−7x2+2x+2 का एक गुणनखंड है ।
View SolutionCheck whether the first polynomial is a factor of the second polynomial by dividing :
x3−3x+1,x5−4x3+x2+3x+1
View SolutionCheck whether the first polynomial is factor of Second polynomial by dividing:
(x3−3x+1),(x5−4x3+x2+3x+1)
View Solution(i) Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial:
t2−3,2t4+3t3−2t2−9t−12
(ii) Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial
x2+3x+1,3x4+5x3−7x2+2x+2
(iii) Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial:
x3−3x+1,x5−4x3+x2+3x+1
View SolutionDivide the second polynomial by the first polynomial and check whether the first polynomial is a factor of the second polynomial. x^3-3x+1,x^5-4x^3+x^2+3x+1
View SolutionCheck whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial :
x3−3x+1,x5−4x3+x2+3x+1
View SolutionCheck whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial :x3−3x+1,x5−4x3+x2+3x+1
View SolutionCheck whether the first polynomial is a factor of the second polynomial by dividing :
x3−3x+1,x5−4x3+x2+3x+1
View SolutionCheck whether the first polynomial is a factor of the second polynomial by applying the division algorithm : t2−3,2t4+3t3−2t2−9t−12 .
View SolutionCheck whether the first polynomial is a factor of the second polynomial by applying the division algorithm : x2+3x+1,3x4+5x3−7x2+2x+2 .
View Solution
