A monkey of mass m clings to a rope over a fixed pulley .
The opposite ends of the rope is tide to a weight of mass M lying on a horizontal plate neglecting friction, find the acceleration of both bodies relative to the plate and tension of the rope in the following case:
(i)the monkey does not move with respect to rope.
(ii)the monkey moves upwards with respect to rope with acceleration β
(iii) the monkey moves downwards with respect to the rope with acceleration β
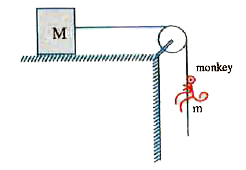
(i) If the monkey is at rest on the rope , then acceleratiion of both mass M and monkey are same , equal to a, (say)
If T is tension in the rope , then the equation of motion of monkey is
mg−T=ma1 ….(1)
and equation of motion of mass M is
T=Ma1 ....(2)
Adding (1) and (2) mg=(M+m)a1<br>:. a_(1)=m/(M+m)g....(3)<br>(ii)Whenmonkeymovesupwardwithae≤rationbeta,thereactionmbetaactsdownward,∴equationsofmotionnowtaketheform(a_(2)isae≤rationofmass,Mrelative→plate)<br>mg+mbeta-T=ma_(2)(formonkey)....(4)<br>T=Ma_(2)(formassM)....(5)<br>Add∈g,we≥t,mg+mbeta=(M+m)a_(2)<br>:. a_(2)=(m(g+beta))/(M+m)<br>:.Theae≤rationofmassrelative→plate<br>a_(2)=(m(g+beta))/(M+m)....(6)<br>andae≤rationofmonkeyMrelative→plate<br>a_(2)=a_(2)-beta=(m(g+beta))/(M+m)-beta =(m(g+beta)-beta(M+m))/(M+m)=(mg+mbeta-mbeta-mbeta)/(M+m)=(Mg-Mbeta)/(M+m)<br>(iii)Whenmonkeymovesdownwardwithae≤rationbeta,tehreaction(R=mbeta)actsupward,∴theequationsofmotiontaketheform(a_(3)isae≤rationofblockrelative→plate)<br>mg-mbeta-T=ma_(3)....(8)<br>T=Ma_(3)....(9)<br>Add∈gwe≥tmg-mbeta=(M+m)a_(3)<br>:. a_(3)=(m(g-beta))/(M+m)<br>andae≤rationofmonkeyrelative→plate<br>a_(3)^(1)=a_(3)+beta=(m(g-beta))/(M+m)+beta=(mg-mbeta+Mbeta+mbeta)/(M+m)<br>:. a_(3)^(1)=(mg+Mbeta)/(M+m)
Similar Questions
A monkey of massm clings to a rope slung over a fixed pulley. The opposite end of the rope is tied to a weight of
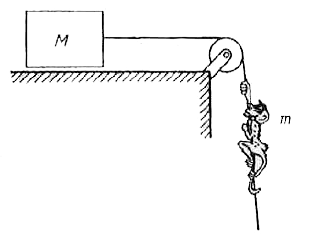
mass M lying on a horizontal plat (Fig.). Neglecting friction find the acceleration of both bodies (relative to the plate) and the tension of the rope for the three cases:
(1) the monkey does not move with respect to the rope,
(2) the monkey moves upwards with respect to the rope with acceleration b,
(3) the monkey moves downwards with respect to the rope with an acceleration b.View SolutionA monkey of mass m climbs up to a rope hung over a fixed pulley. The opposite end of the rope is tied to a weight of mass M lying on a horizontal plane. Neglecting the friction, find the acceleration of both the bodies (relative to the plane) and the tension of the rope for the three cases:
(i) The monkey does not move with respect to the rope
(ii) The monkey moves upwards with respect to the rope with acceleration a.
(iii) The monkey moves downwards with respect to the rope, with acceleration a.View Solution
Knowledge Check
- Question 1 - Select One
A monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight and the tension of the rope for two cases .The monkey move downward with respect to the rope with an acceleration b.
The tension of rope isAMm(μg+g+b)M+mBMm(μg−g+b)M+mCMm(μg+g−b)M+mDMm(μg−g−b)M+m - Question 2 - Select One
A monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight and the tension of the rope for two cases .The monkey move downward with respect to the rope with an acceleration b.
The acceleration of weight isA2m(g+b)−μMgM+2mBm(g+b)−μMg2(M+m)Cm(g+b)−3μMgM+3mDm(g+b)−μMgM+2m - Question 3 - Select One
A cat of mass m = 1 kg climbs to a rope hung over a leight frictionless pulley. The opposite end of the rope is tied to a weight of mass M = 2m lying on a smooth horizontal plane. What is the tension of the rope where the cat move upwards with in acceleration a=2m/s2 relative to the rope ?
 A2 NB12NC8ND4N
A2 NB12NC8ND4N
A monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight and the tension of the rope for two cases .The monkey move downward with respect to the rope with an acceleration b.

The tension of rope isView SolutionA monkey of mass m clings a rope to a slung over a fixed pulley .The opposite end of the rope is tried to a weight of mass M tying on a horizontal table is μ Find the acceleration of weight .The monkey move downward with respect to the rope with an acceleration b.

The acceleration of weight isView SolutionA monkey of mass 'm' climbs up to a rope hung over a fixed pulley with an acceleration g/4. The opposite end of the rope is tied to a block of mass M lying on a rough horizontal plane. The coefficient of friction between the block and horizontal plane is μ. Find the tension in the rope.
 View Solution
View SolutionA monkey of mass 'm' climbs up to a rope hung over a fixed pulley with an acceleration g/4. The opposite end of the rope is tied to a block of mass M lying on a rough horizontal plane. The coefficient of friction between the block and horizontal plane is μ. Find the tension in the rope.
 View Solution
View SolutionA cat of mass m = 1 kg climbs to a rope hung over a leight frictionless pulley. The opposite end of the rope is tied to a weight of mass M = 2m lying on a smooth horizontal plane. What is the tension of the rope when the cat move upwards with in acceleration a=2m/s2 relative to the rope ?
 View Solution
View Solution
