If a solid cylinder of area of cross-section A is moving with velocity V in medium of density p then power loss of cylinder is
pAV2
12pAV2
13pAV3
12pAVv2
To find the power loss of a solid cylinder moving through a medium, we can use the following steps:
1. Understand Power Loss: Power loss can be defined as the work done per unit time. In the context of a solid cylinder moving through a fluid, the power loss is due to the drag force acting against the motion of the cylinder.
2. Identify the Drag Force: The drag force Fd experienced by the cylinder moving through a medium can be expressed as:
Fd=Cd⋅12⋅p⋅A⋅V2
where:
- Cd is the drag coefficient (depends on the shape of the object),
- p is the density of the medium,
- A is the cross-sectional area of the cylinder,
- V is the velocity of the cylinder.
3. Calculate Power Loss: The power loss P due to the drag force can be calculated using the formula:
P=Fd⋅V
Substituting the expression for Fd:
P=(Cd⋅12⋅p⋅A⋅V2)⋅V
Simplifying this gives:
P=Cd⋅12⋅p⋅A⋅V3
4. Final Expression: Thus, the power loss of the cylinder moving in a medium is given by:
P=Cd⋅12⋅p⋅A⋅V3
Fd=Cd⋅12⋅p⋅A⋅V2
where:
- Cd is the drag coefficient (depends on the shape of the object),
- p is the density of the medium,
- A is the cross-sectional area of the cylinder,
- V is the velocity of the cylinder.
P=Fd⋅V
Substituting the expression for Fd:
P=(Cd⋅12⋅p⋅A⋅V2)⋅V
Simplifying this gives:
P=Cd⋅12⋅p⋅A⋅V3
P=Cd⋅12⋅p⋅A⋅V3
Similar Questions
Consider a solid cylinder of the density ρs cross section area A and h ploating in a liquid of density ρl as shown in figure (ρl>ρs) . It is depressed sligtly and allowed to oscillation.
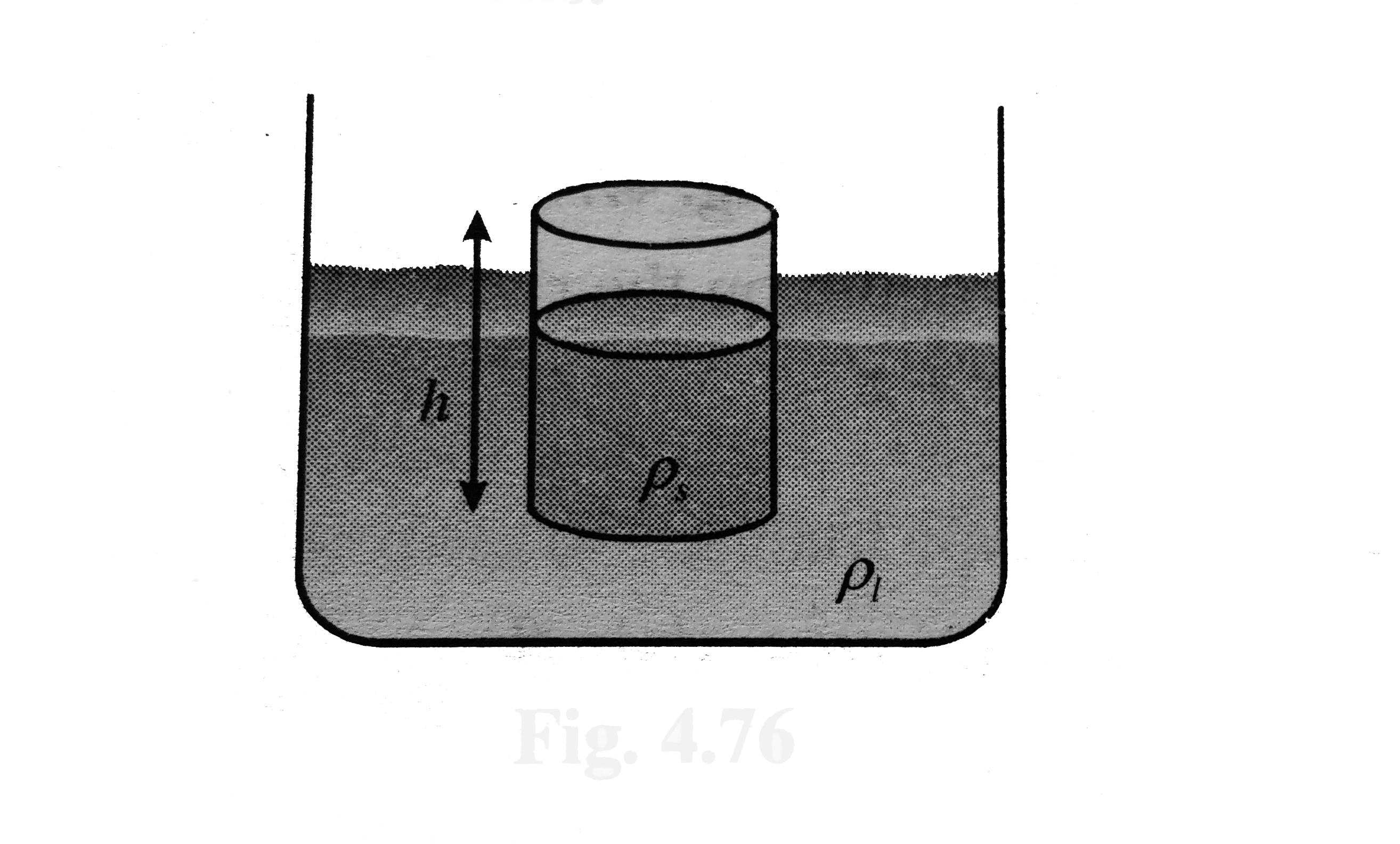
View SolutionConsider a solid cylinder of height h, density ρs, cross-sectional area A is floating in a liquid of density ρl. The frequency of vertical oscillations was found to be gahbρci,ρds when depressed slightly and released. Then what is the value of a2bcd ?
View Solution
Knowledge Check
A solid cylinder of length l and cross-sectional area A is made of a material whose resistivity depends on the distance r from the axis of the cylinder as ρ=k/r2 where k is constant . The resistance of the cylinder is -
AπklA2B2πklA2CπklADNoneA solid cylinder rolls up an inclined plane of inclination θ with an initial velocity v. How far does the cylinder go up the plane ?
A3v22gsinθBv24gsinθC3v2gsinθD3v24gsinθA cylinder of mass m and density ρ hanging from a string is lowered into a vessel of cross-section area s containing a liquid of density σ(<ρ) unit it is fully immersed. The increase in pressure at the bottom of the vessel is
AmρgσsBmgsCmσgρsDzero
A liquid of density ρ is filled in a beaker of cross section A to a height H and then a cylinder of mass M and cross-section a is made to float in it as shown in

If the atmospheric pressure is P0 find the pressure (a) at the top face B of the cylinder (b) at the bottom face C of the cylinder and (c ) at the base D of the beaker. (d) Can ever these pressure be equal ?
View SolutionA solid cylinder of mass 20kg rotates about its axis with angular velocity of 100 radian s−1. The radius of the cylinder is 0.25m. The magnitude of the angular momentum of the cylinder about its axis of rotation is
View SolutionA solid cylinder of mass 'M' and radius 'R' is rotating along its axis with angular velocity ω without friction. A particle of mass 'm' moving with velocity v collides aganist the cylinder and sticks to its rim. After the impact calculate angular velocity of cylinder .
View SolutionIf A is the area of cross section of conductor, vd is the drift velocity of electrons and n is the number of electrons per unit volume, then current density through conductor is
View SolutionVelocity of the centre of a small cylinder is v. There is no slipping anywhere. The velocity of the centre of the larger cylinder is

View Solution
