Father (80 kg) and son (30 kg) are sitting at one of the ends of a 4 m long boat (40 kg) standing still on water. They start to shift slowly. Father stopped at centre of boat but son stopped at other end. Neglecting friction with water, how far does the boat move on the water during the process?
1.25 m
1.2 m
1.87 m
1.5 m
To solve the problem, we will use the concept of the center of mass and the principle of conservation of momentum. Here are the steps:
Step 1: Define the system
We have a father (mass = 80 kg), a son (mass = 30 kg), and a boat (mass = 40 kg). The total length of the boat is 4 m. Initially, both the father and son are sitting at one end of the boat.
Step 2: Determine the initial position of the center of mass
To find the center of mass (CM) of the system, we can use the formula:
CM=M1⋅d1+M2⋅d2+M3⋅d3M1+M2+M3
Where:
- M1 = mass of the father = 80 kg
- M2 = mass of the son = 30 kg
- M3 = mass of the boat = 40 kg
Assuming the left end of the boat is at position 0 m, the initial positions are:
- Father at 0 m
- Son at 0 m
- Boat's center of mass at 2 m (since the boat is 4 m long)
The distances from the left end (0 m) to the center of mass of the system can be calculated as:
- d1=0 m (father)
- d2=0 m (son)
- d3=2 m (boat)
Step 3: Calculate the initial center of mass
Substituting the values into the center of mass formula:
CM=(80⋅0)+(30⋅0)+(40⋅2)80+30+40=0+0+80150=80150≈0.53 m
Step 4: Determine the final positions after movement
The father moves to the center of the boat (2 m from the left end), and the son moves to the opposite end of the boat (4 m from the left end).
Step 5: Calculate the new center of mass
Now, we recalculate the center of mass with the new positions:
- Father at 2 m
- Son at 4 m
- Boat's center of mass remains at 2 m
The new distances are:
- d1=2 m (father)
- d2=4 m (son)
- d3=2 m (boat)
Substituting these values into the center of mass formula:
CM=(80⋅2)+(30⋅4)+(40⋅2)80+30+40=160+120+80150=360150=2.4 m
Step 6: Calculate the movement of the boat
Since the center of mass of the system must remain constant and the initial center of mass was at approximately 0.53 m, the boat must move to keep the center of mass at the same position.
Let x be the distance the boat moves. The new center of mass position must equal the initial center of mass position:
Initial CM=Final CM
0.53=(80⋅(2+x))+(30⋅(4+x))+(40⋅(2+x))150
Solving for x:
0.53⋅150=80(2+x)+30(4+x)+40(2+x)
79.5=160+80x+120+30x+80+40x
79.5=360+150x
150x=79.5−360
150x=−280.5
x=−1.87 m
Step 7: Conclusion
The negative sign indicates that the boat moves 1.87 m in the opposite direction to the movement of the son.
Final Answer
The boat moves approximately 1.87 m in the opposite direction.
---
We have a father (mass = 80 kg), a son (mass = 30 kg), and a boat (mass = 40 kg). The total length of the boat is 4 m. Initially, both the father and son are sitting at one end of the boat.
To find the center of mass (CM) of the system, we can use the formula:
CM=M1⋅d1+M2⋅d2+M3⋅d3M1+M2+M3
Where:
- M1 = mass of the father = 80 kg
- M2 = mass of the son = 30 kg
- M3 = mass of the boat = 40 kg
- Father at 0 m
- Son at 0 m
- Boat's center of mass at 2 m (since the boat is 4 m long)
- d1=0 m (father)
- d2=0 m (son)
- d3=2 m (boat)
Substituting the values into the center of mass formula:
CM=(80⋅0)+(30⋅0)+(40⋅2)80+30+40=0+0+80150=80150≈0.53 m
The father moves to the center of the boat (2 m from the left end), and the son moves to the opposite end of the boat (4 m from the left end).
Now, we recalculate the center of mass with the new positions:
- Father at 2 m
- Son at 4 m
- Boat's center of mass remains at 2 m
- d1=2 m (father)
- d2=4 m (son)
- d3=2 m (boat)
CM=(80⋅2)+(30⋅4)+(40⋅2)80+30+40=160+120+80150=360150=2.4 m
Since the center of mass of the system must remain constant and the initial center of mass was at approximately 0.53 m, the boat must move to keep the center of mass at the same position.
Initial CM=Final CM
0.53=(80⋅(2+x))+(30⋅(4+x))+(40⋅(2+x))150
0.53⋅150=80(2+x)+30(4+x)+40(2+x)
79.5=160+80x+120+30x+80+40x
79.5=360+150x
150x=79.5−360
150x=−280.5
x=−1.87 m
The negative sign indicates that the boat moves 1.87 m in the opposite direction to the movement of the son.
The boat moves approximately 1.87 m in the opposite direction.
Topper's Solved these Questions
Similar Questions
Mr. Verma (50kg) and Mr. Mathur (60kg) are sitting at the two extremes of a 4 m long boat (40 kg) standing still in water. To discuss a mechanics problem, they come to the middle of the boat. Neglecting frictioin with water how far does the boat move on the water during the process?
View SolutionTwo boys A and B of masses 60kg and 40kg respectively are at the ends of a 4m long boat of mass 100kg which is on water in lake and the system is at rest.If the boys meet at the centre of the boat then find the displacement of centre of mass of the boat in meter (neglect friction between water and boat)
View Solution
Knowledge Check
Which of the following statements are true ?
(i) A uniform wooden plank of mass 150kg and length 8m is floating on still water with a man of 50kg at one end of it. The man walks to the other end of the plank and stops. The distance covered by the plank is 2m.
(ii) A kid of mass 15kg is sitting in a boat of mass 45kg in a lake. The distance of kid from the bank of lake is 12m. Now the body moves inside the boat at a distance 4m towards the bank and stops. The distance of the kid from the bank (there is no friction between the water and the boat ) is 11m.
(iii) Two persons of masses 40kg and 60kg are sitting at the midpoint of a 12−m- long boat (140kg) standing still in water. Now they move to opposite ends of the boat. Neglecting the friction between boat and water, the distance traveled by the boat in water is 50cm.
(iv) In a gravity free space , a man of mass m standing at a height h above the floor, throws a ball of mass m0 straight down with a speed v0. When the ball reaches the floor, the distance of the man above the floor is (1+m0m)h.A(i),(ii)B(i) ,(iii) ,(iv)C(ii),(iii),(iv)Dall of the aboveA boy (massof40kg) is standing at one end of a boat (mass of 60 kg) in still water. The length of the boat is 10m and the boy takes 2s to reach at other end of boat moving with constant speed. Assuming no friction between the boat and the water.
(i) The distance covered by the boat is 4m
(ii) The distance covered by the boy with respect to the ground is 6m.
(iii) The velocity of the boy with respect to the ground is <5m/s
(iv) The velocity of the boy is 3m/sA(i),(ii)B(ii),(iii)C(i),(ii),(iii)Dall options are correctA boy of mass 50kg is standing at one end of a boat of length 9m and mass 400kg. He runs to the other end. The distance through which the centre of mass of the boat boy system moves is
A0B1mC2mD3m
Two men, of masses 60 kg and 80 kg are sitting at the ends of a boat of mass 60 and length 4 m. the boat is stationary. If the men now exchange their position, then:
View SolutionA dog of mass 10 kg is standing on a flat 10 m long boat so that it is 20 m meters from the shore. It walks 8 m on the boat towards the shore and then stops. The mass of the boat is 40 kg and friction between the boat and the water surface is negligible. How far is the dog from the shore now ?

View SolutionA boat of length 10m and mass 450kg is floating without motion in still water. A man of mass 50kg standing at one end of it walks to the other end of it and stops. The magnitude of the displacement of the boat in metres relative to ground is
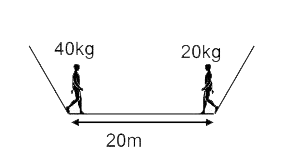
View SolutionTwo men of masses 40 kg and 20 kg are standing on a boat of mass 100 kg. Length of boat is 20m. Neglect the friction between water and boat. Find the displacement of the boat when both the persons reach at middle of boat.
View SolutionA man of mass 60 kg is standing on a boat of mass 140 kg, which is at rest in still water. The man is initially at 20 m from the shore. He starts walking on the boat for 4 s with constant speed 1.5 m/s towards the shore. The final distance of the man from the shore is
View Solution
