The change in internal energy of a gas kept in a closed container when 50 cal heat is supplied to it is
10 cal
40 cal
50 cal
100 cal
To find the change in internal energy of a gas kept in a closed container when 50 calories of heat is supplied, we can follow these steps:
Step 1: Understand the System
The gas is in a closed container, which means that the volume of the gas remains constant.
Step 2: Recall the First Law of Thermodynamics
The first law of thermodynamics states:
ΔQ=ΔU+W
where:
- ΔQ is the heat added to the system,
- ΔU is the change in internal energy,
- W is the work done by the system.
Step 3: Determine Work Done
Since the volume is constant, there is no expansion or compression of the gas. Therefore, the work done W is zero:
W=0
Step 4: Substitute into the First Law
Now, substituting W=0 into the first law equation:
ΔQ=ΔU+0
This simplifies to:
ΔQ=ΔU
Step 5: Apply the Given Heat
We are given that the heat supplied ΔQ is 50 calories:
ΔQ=50 cal
Step 6: Conclude the Change in Internal Energy
From the equation ΔQ=ΔU, we can conclude that:
ΔU=50 cal
Thus, the change in internal energy of the gas is 50 calories.
Final Answer:
The change in internal energy of the gas is 50 calories.
---
The gas is in a closed container, which means that the volume of the gas remains constant.
The first law of thermodynamics states:
ΔQ=ΔU+W
where:
- ΔQ is the heat added to the system,
- ΔU is the change in internal energy,
- W is the work done by the system.
Since the volume is constant, there is no expansion or compression of the gas. Therefore, the work done W is zero:
W=0
Now, substituting W=0 into the first law equation:
ΔQ=ΔU+0
This simplifies to:
ΔQ=ΔU
We are given that the heat supplied ΔQ is 50 calories:
ΔQ=50 cal
From the equation ΔQ=ΔU, we can conclude that:
ΔU=50 cal
The change in internal energy of the gas is 50 calories.
Topper's Solved these Questions
Similar Questions
(a) An ideal gas contained in a cylinder is given 200 J of heat. The gas does 60 J of work in the expansion resulting from heating. Calculate the increase in internal energy in the process.
(b) Calculate the change in internal energy of a gas kept in a rigid container when 150 J of heat is supplied to it.
View Solutioncalculate the change in internal energy of a gas kept in a rigid container when 100 J of heat is supplied to it.
View Solution
Knowledge Check
The change in internal energy of a gas kept in a rigid cylinder on supplying 120 J of heat is
A0 JB60 JC100 JD120 J
A cylinder contains 3 moles of oxygen at a temperature of 27∘C. The cylinder is provided with a frictionless piston which maintains a constant pressure of 1 atm on the gas. The gas is heated until its temperature rises to 127∘C.
a. How much work is done by the gas in the process ?
b. What is the change in the internal energy of the gas ?
c. How much heat was supplied to the gas ?
For oxygen CP=7.03calmol−1.∘C−1.
View SolutionCalculate the change in internal energy when 5g of air is heated from internal energy when 5g of air is heated from 0∘Cto2∘C. Specific heat of air at constant volume is 0.172cal/g/∘C.
View SolutionFind the change in internal energy (in joule) of a gas when it absorbs 40calories of heat and performs work equal to 16J.
View SolutionFigure. Shows an ideal gas changing its state A to state C by two different path ABC and AC.
a. Find the path along which the work done is the least.
b. The internal energy of the gas at A is 10J and the amount of heat supplied to change its state to C through the path AC is 200J. Find the internal energy at C.
c. The internal energy of the gas at state B is 20J. Find the amount of heat supplied to the gas to go from state A to state B.
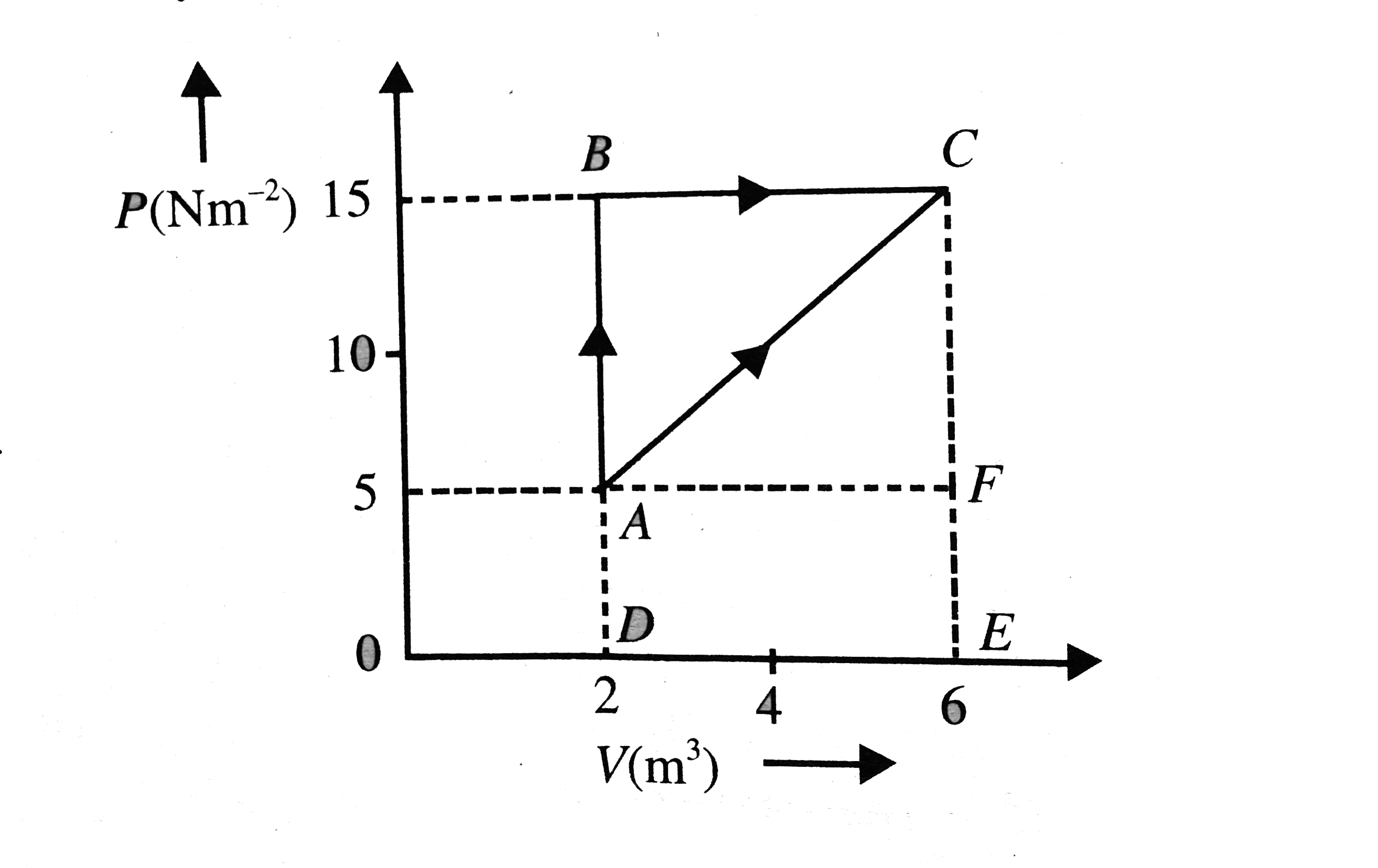
View Solution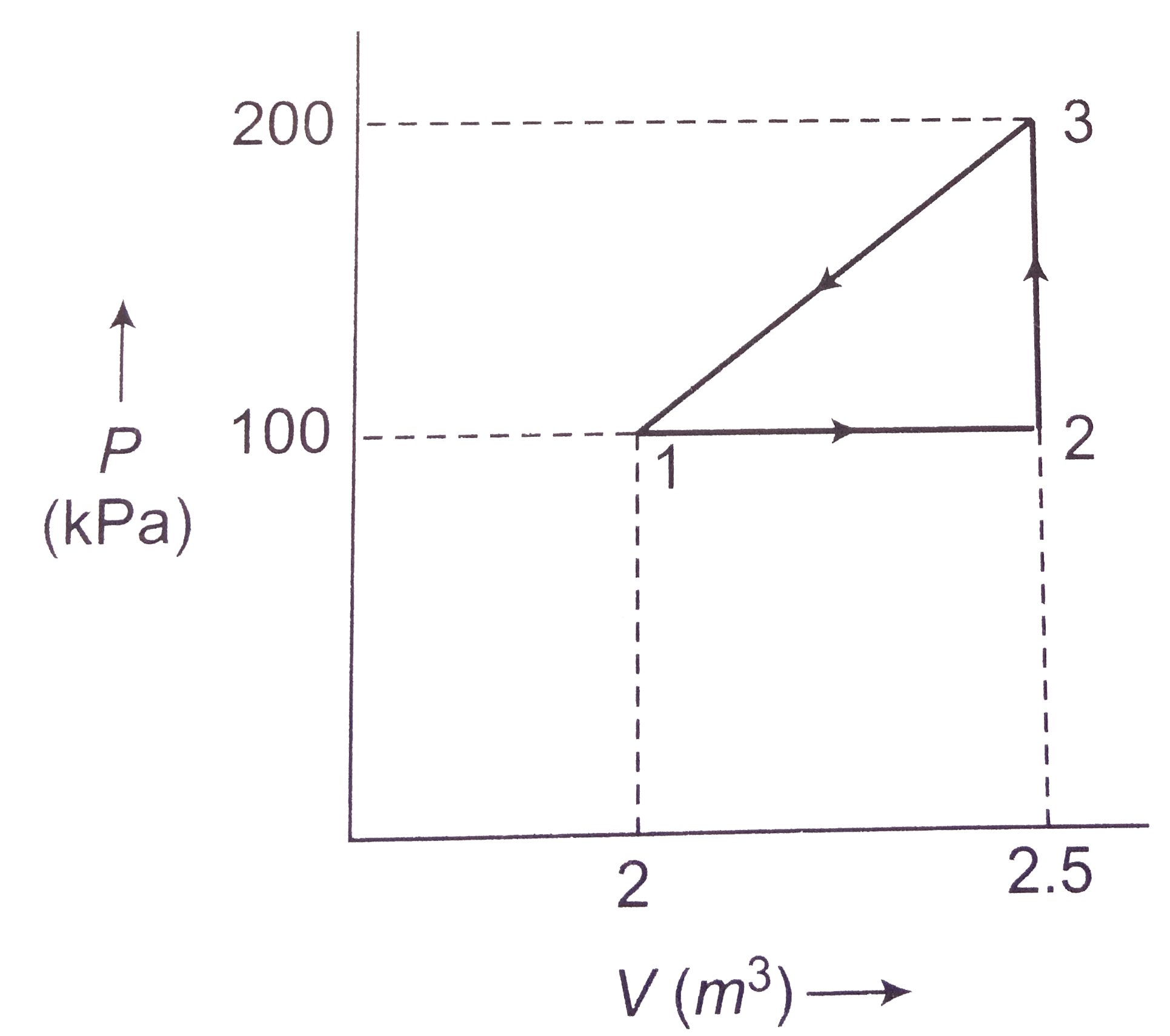
In the figure an ideal gas changes is state from state A to state C by two paths ABC and AC.
(a) Find the path along which the work done is least.
(b) The internal energy of the gas at A is 10 J and the amount of heat supplied to change its state to C through the path AC is 200 J. Calculate the internal energy at C.
(c) The internal energy of the gas at state B is 20 J. Fid the amount of the heat supplied to gas to go from A to B.
View SolutionThe molar heat capacity for an ideal monatomic gas is 3R.If dQ is the heat supplied to the gas and dU is the change in its internal energy then for the process the ratio of work done by the gas and heat supplied is equal to
View SolutionIn the given figure, an ideal gas changes it state from A to state C by two paths ABC and AC.
(i) Find the path along which work done is the least.
(ii) The internal energy of the gas at A to 10J and the amount of heat supplied to change its state to C through the path AC is 200J. Calculate the internal energy at C.
(iii) The internal energy of the gas at state B is 30J. Find the amount of heat supplied to the gas to go from A→B.

View Solution