A uniform rope of length 12 m and mass 4 kg is hung vertically from a rigid support transverse wave pulse is produced at lower end. The speed of the wave pulse al x = 2.5 m from lower and will be kg=10 m/s)
10 m/s
5 m/s
7.5 m/s
2.5 m/s
To solve the problem of finding the speed of the wave pulse at a distance of x=2.5m from the lower end of a uniform rope, we can follow these steps:
Step 1: Identify the parameters
- Length of the rope, L=12m
- Mass of the rope, M=4kg
- Distance from the lower end, x=2.5m
- Acceleration due to gravity, g=10m/s2
Step 2: Calculate the mass per unit length (μ)
The mass per unit length of the rope can be calculated using the formula:
μ=ML=4kg12m=13kg/m
Step 3: Determine the tension (T) at distance x
The tension in the rope at a distance x from the lower end is due to the weight of the rope below that point. The weight of the rope segment below x can be calculated as:
T=Weight of the rope below x=mass of the rope below x⋅g
The mass of the rope below x is given by:
mass below x=μ⋅x=(13kg/m)⋅(2.5m)=2.53kg
Thus, the tension T becomes:
T=(2.53kg)⋅(10m/s2)=253N
Step 4: Calculate the speed of the wave pulse (v)
The speed of the wave pulse in a rope is given by the formula:
v=√Tμ
Substituting the values of T and μ:
v=
⎷253N13kg/m=√25=5m/s
Final Answer
The speed of the wave pulse at x=2.5m from the lower end is 5m/s.
---
- Length of the rope, L=12m
- Mass of the rope, M=4kg
- Distance from the lower end, x=2.5m
- Acceleration due to gravity, g=10m/s2
The mass per unit length of the rope can be calculated using the formula:
μ=ML=4kg12m=13kg/m
The tension in the rope at a distance x from the lower end is due to the weight of the rope below that point. The weight of the rope segment below x can be calculated as:
T=Weight of the rope below x=mass of the rope below x⋅g
The mass of the rope below x is given by:
mass below x=μ⋅x=(13kg/m)⋅(2.5m)=2.53kg
Thus, the tension T becomes:
T=(2.53kg)⋅(10m/s2)=253N
The speed of the wave pulse in a rope is given by the formula:
v=√Tμ
Substituting the values of T and μ:
v=
⎷253N13kg/m=√25=5m/s
The speed of the wave pulse at x=2.5m from the lower end is 5m/s.
Topper's Solved these Questions
Similar Questions
A uniform rope of length 12m and mass 6kg hangs vertically from a rigid support . A block of mass 2kg is attached to the free end of the rope . A transverse pulse of wavelengths 0.06m is produced at the lower end of the rope . What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
View Solution
Knowledge Check
A Uniform rope having mass m hags vertically from a rigid support. A transverse wave pulse is produced at the lower end. The speed v of wave pulse varies with height h from the lower end as
A B
B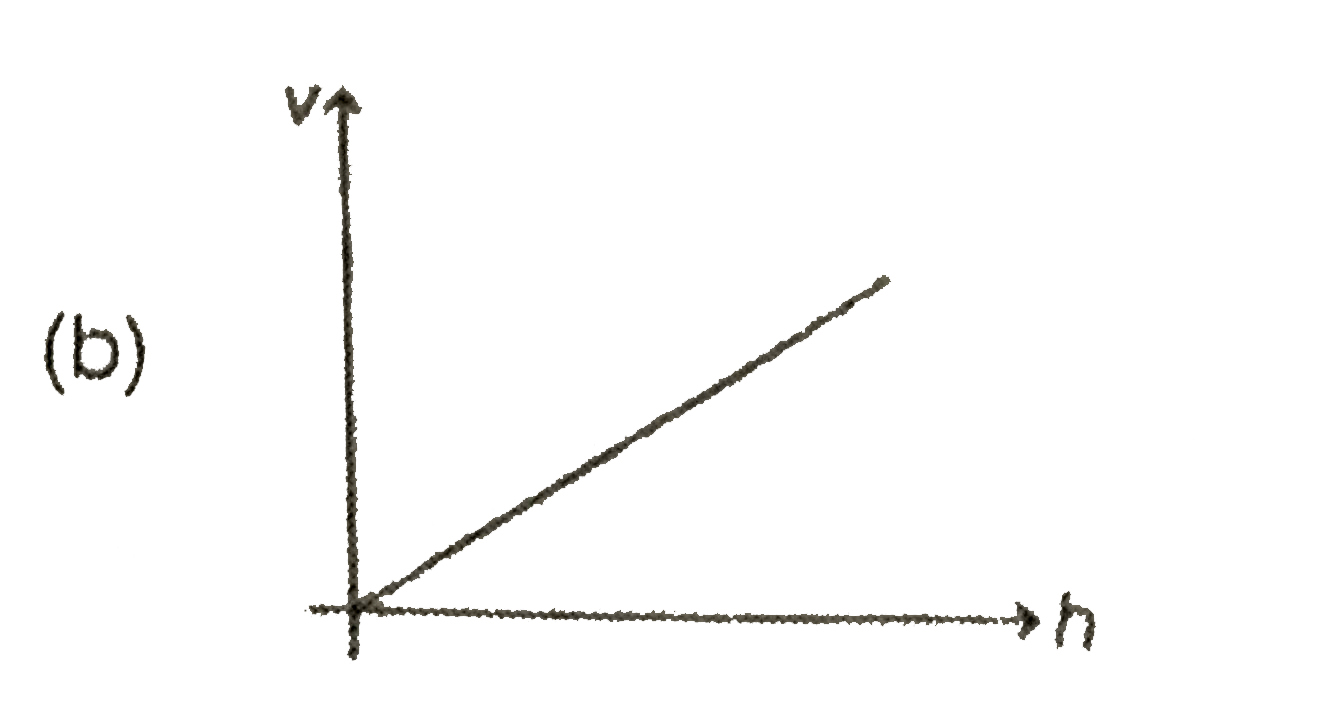 C
C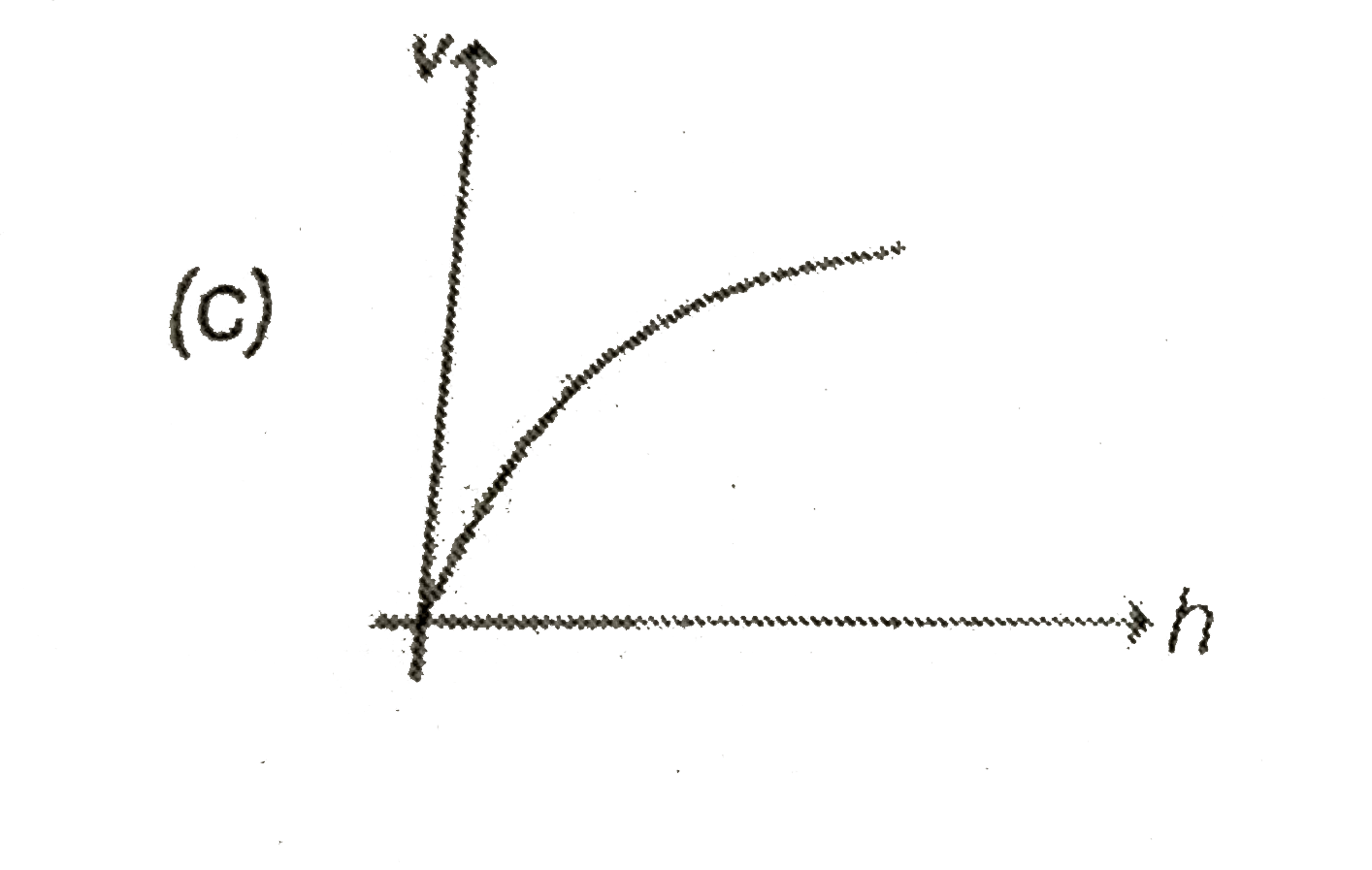 D
D
A uniform rope having some mass hinges vertically from a rigid support. A transverse wave pulse is produced at the lower end. The speed (v) of the wave pulse varies with height (h) from the lower end as :-
A B
B C
C D
D
A uniform rope having some mass hinges vertically from a rigied support. A transverse wave pulse is produced at the lower end. The speed (v) of the wave pulse varies with height (h) from the lower end as :-
A_E01_020_O01.png) B
B_E01_020_O02.png) C
C_E01_020_O03.png) D
D_E01_020_O04.png)
A uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 12m and mass 12kg hangs vertically from a rigid support. A block of mass 2kg is attached to free end of the rope. A traverse pulse of wave length 0.05 m is produced at the lower end of the rope. If λ is the wave length when pulse reached at mid point of the rope, then write 10λ in the OMR sheet.
View SolutionA uniform rope of length 20m and mass 5kg is hanging vertically from a rigid support. A block of mass 4kg is attached to the free end. The wave length of the transverse wave pulse at the lower end of the rope is 0.04 m. The wavelength of the same pulse as it reaches the top is
View SolutionA uniform rope of length 10 m and mass 3 kg hangs vertically from a rigid support. A block of mass 1 kg is attached to the free end of the rople. A transverse pulse of wavelength 0.05 m is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is
View SolutionA uniform string of length 20 m & mass 1 kg is hung vertically. Find the speed of wave at the mid point of the string :
View Solution