A particle executes simple harmonic motion according to the equation x=5sin(2π3t) Find the period of the oscillationSolution in Malayalam
Step by step video & image solution for A particle executes simple harmonic motion according to the equation x=5sin((2π)/3t) Find the period of the oscillation by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Fill in the blanks:
A girl is swinging on a swing in a sitting position. When she stands up, the period of the swing will...........
View SolutionWhich of the following curves does not represent motion in one dimension?
View SolutionThe word 'Science' originated from a Latin verb. Which is that verb?
View SolutionThe maximum velocity a particle, executing simple harmonic motion with an amplitude 7 mm, 4.4 m//s. The period of oscillation is.
View SolutionThe equation of motion of a particle executing simple harmonic motion is a+16π2x=0 In this equation, a is the linear acceleration in m/s2 of the particle at a displacement x in meter. The time period in simple harmonic motion is
View SolutionThe acceleration displacement graph of a particle executing simple harmonic motion is shown in figure. The time period of simple harmonic motion is
View SolutionThe acceleration-displacement (a−X) graph of a particle executing simple harmonic motion is shown in the figure. Find the frequency of oscillation.

View SolutionFigure shows the graph of velocity versus displacement of a partciel executing simple harmonic motion. Find the period of oscillation of the particle.
View SolutionA particle executes simple harmonic motion according to equation 4d2xdt2+320x=0. Its time period of oscillation is :-
View SolutionThe maximum velocity of a particle , executing simple harmonic motion with an amplitude 7mm is 4.4ms−1 . The period of oscillation is
View SolutionThe maximum velocity of a particle executing simple harmonic motion with an amplitude 7 mm is 4.4 m/s. The period of oscillation is :
View SolutionA particle executing simple harmonic motion with time period T. the time period with which its kinetic energy oscillates is
View SolutionFigure shows the graph of velocity versus displacement of a particle executing simple harmonic motion. Find the period of oscillation of the particle

View SolutionFigure shows the variation of force acting on a particle of mass 400g executing simple harmonic motion. Find the frequency of oscillation of the particle
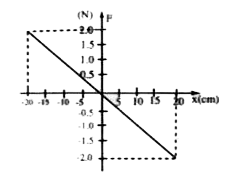
View SolutionA particle executes simple harmonic motion according to the equation x=5sin(2π3t) what is the minimum time required for the particle to move between two points 2.5 cm on either side of the mean position?
View Solution
