[Marathi] A particle of mass m and charge q is accelerated through a p

The region surrounding a stationary electric dipole has

When a bus suddenly takes a turn, the passengers are thrown outwards because of

The property of attracting small pieces of iron by a substance is referred as

A charged particle of mass $m$ and charge $q$ is accelerated through a potential differences of $V$ volts. It enters of uniform magnetic field $B$ which is directed perpendicular to the direction of motion of the particle. The particle will move on a circular path of radius

View Solution

A charged particle of mass m and charge q is accelerated through a potential difference of V volts. It enters a region of uniform magnetic field which is directed perpendicular to the direction of motion of the particle. Find the radius of circular path moved by the particle in magnetic field.

View Solution

A charged particle moving with a uniform velocity $\to v$ enters a region where uniform electric and magnetic fields $\to E$ and $\to B$ are present. It passes through the region without any change in its velocity. What can we conclude about the (i) relative direction of $\to E$ , $\to V$ and $\to B$ ? (ii) magnetic of $\to E$ and $\to B$ ?
or
Find the condition under which the charged particle moving with different speeds in the presence of electric and magnetic field vectors can be used to select charged particles of a particular speeds.

View Solution

A particle of mass m and charge q is accelerated through a potential differece V to a velocity $\to v$ towards south. The particle enters a region with both a magnetic field $\to B$ (pointing eastwards) and electric field $\to E$ (pointing downwards). The particle travels with a constant velocity through this region. The potential difference V through this region should be equal to

View Solution

A charge particle of charge q, mass m is projected with a velocity $\to v = v ˆ i$ in a region of space where electric field strength $\to E = E ˆ k$ and magnetic induction $\to B = B ˆ j$ are present. The acceleration of the particle is:
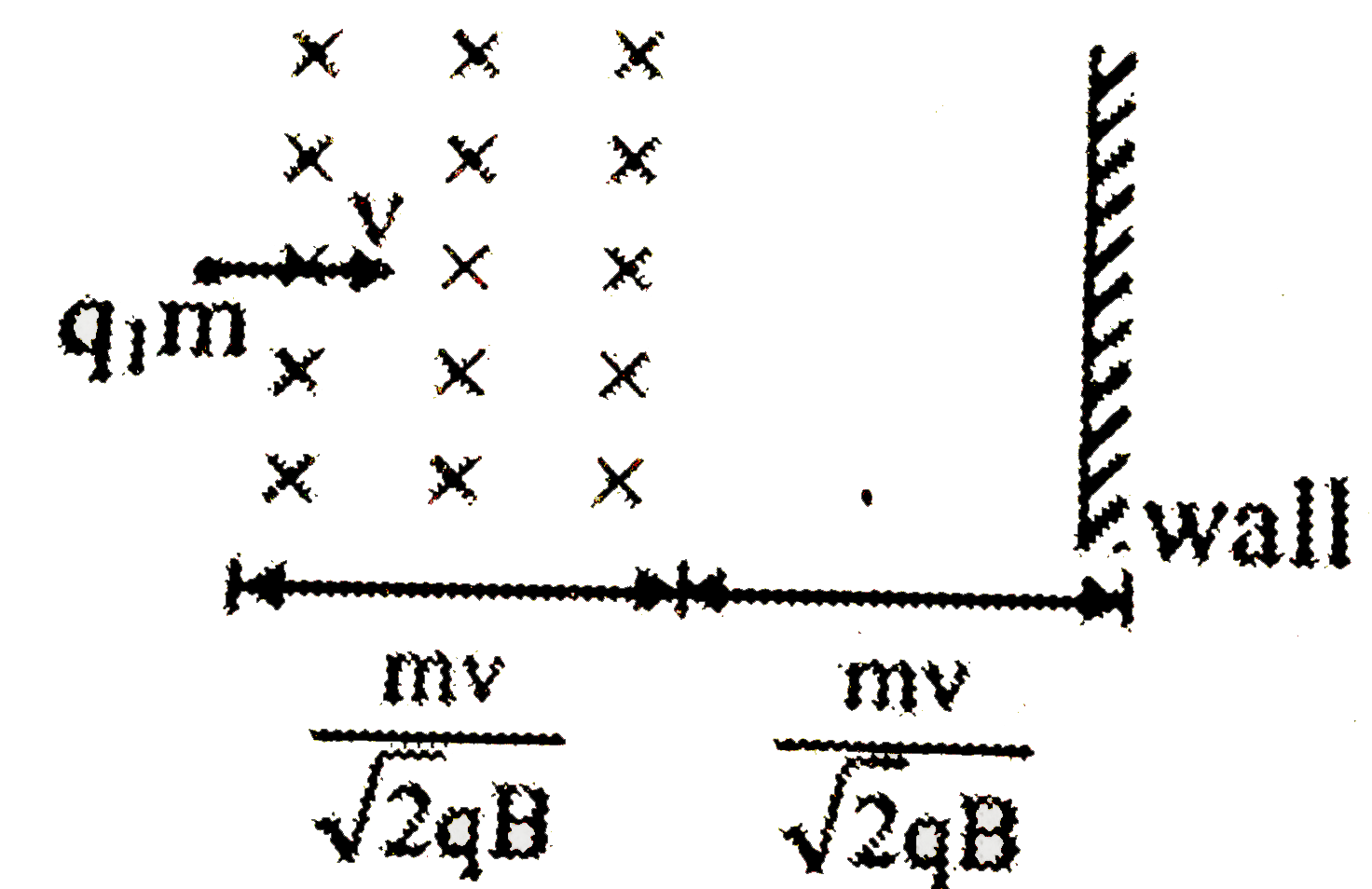
ocr_image

View Solution

A particle of mass m and charge q enters a region of magnetic field (as shown) with speed v at t = 0. There is a region in which the magnetic field is absent as shown. The particle after entering the region collide elastically with a rigid wall. Time t after which the velocity of particle become antiparallel to its initial velocity is -
ocr_image

View Solution

The region between y =0 and y=d constains a magnetic field vecB = Bvecz . A particle of mass m and charge q enters of the region with a velocity vecv = v veci . If d =(mv)/(2qB) the acceleration of the charged particle at the point of its emergence at the other side is :

View Solution

In a region, electric field $\to E$ and magnetic field $\to B$ are mutually perpendicular. If a partical of charge q enters that region with velocity $\to v$ normal to both the fields, under what condition does the direction of motion of the particle remain unchanged ?

View Solution

Write the expression for the force vecF acting on a particle of charge q moving with a velocity vecv in the prensnece of both electric field vecE and magnetic field vecB . Obtain the condition under which the particle moves undeflected through the fields.

View Solution

The force experienced by a particle having mass m and charge q accelerated through a potential difference V when it is kept under perpendicular magnetic field vecB is

View Solution

A charged particle, having charge $q_{1}$ accelerated through a potential difference V enter a perpendicular magnetic field region in which it experiences a force F. If V is increased to 5V, the particle will experience a force

View Solution

A charged particle with charge q enters a region of constant, uniform and mutually orthogonal fields vecE and vecB with a velocity vecv perpendicular to both vecE and vecB , and comes out without any change in magnitude or direction of vecv . Then

View Solution

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

TARGET PUBLICATION-MAGNETIC EFFECT OF ELECTRIC CURRENT -Evaluation Test