When some food particle enters the windpipe instead of oesphagus it is expelled by the process of
(a) sneezing
(b) coughing
(c) yawning
(d) hiccupping
The correct Answer is:B
Step-by-Step Solution:
1. Understanding the Problem: The question states that food particles can sometimes enter the windpipe (trachea) instead of the esophagus (food pipe). This can lead to choking, which is a serious situation.
2. Identifying the Body's Response: When food particles enter the windpipe, it creates an obstruction in the respiratory tract. The body has mechanisms to respond to such obstructions.
3. Role of the Medulla Oblongata: The medulla oblongata is a part of the brain that controls various involuntary functions, including the respiratory center. When an obstruction occurs, it sends signals to the medulla oblongata.
4. Cough Reflex: The medulla oblongata activates the cough center in response to the obstruction. This initiates the cough reflex, which is a protective mechanism to expel foreign particles from the respiratory tract.
5. Expulsion of Food Particles: Through the process of coughing, the food particles that entered the windpipe are expelled out from the mouth, thereby clearing the airway.
6. Conclusion: The correct answer to the question is that food particles that enter the windpipe are expelled by the process of coughing.
Final Answer: Coughing (Option B).
---
Topper's Solved these Questions
Similar Questions
When a charged particle enters a uniform magnetic field its kinetic energy
View SolutionWhen only one type of solute molecule enters the cell with the help of carrier protein, the process is
View SolutionA negatively charged particle enters in a semi-infinite uniform magnetic field vecB, directed out the plane of paper as shown in the figure. Through what angle it is is deviated when it leaves the magnetic field?
View SolutionA positively charged particle of mass m and charge q is projected on a rough horizontal x-y plane surface with z-axis in the vertically upward direction. Both electric and magnetic fields are acting in the region and given by →E=−E0ˆkand→B=−B0ˆk , respectively. The particle enters into the field at (a0,0,0) with velocity →v=v0ˆj. The particle starts moving in some curved path on the plane. If the coefficient of friction between the particle and the plane is μ. Then calculate the
(a) time when the particle will come to rest
(b) distance travelled by the particle when it comes to rest.

View SolutionA positively charged particle of mass m and charge q is projected on a rough horizontal x-y plane surface with z-axis in the vertically upward direction. Both electric and magnetic fields are acting in the region and given by →E=−E0ˆkand→B=−B0ˆk , respectively. The particle enters into the field at (a0,0,0) with velocity →v=v0ˆj. The particle starts moving in some curved path on the plane. If the coefficient of friction between the particle and the plane is μ. Then calculate the
(a) time when the particle will come to rest
(b) distance travelled by the particle when it comes to rest.

View SolutionWhen a particle is projected at some angle with the horizontal, the path of the particle is parabolic. In the process the horizontal velocity remains constant but the magnitude of vertical velocity changes. At any instant during flight the acceleration of the particle remains g in vertically downward direction. During flight at any point the path of particle can be considered as a part of circle and radius of that circle is called the radius of curvature of the path
Consider that a particle is projected with velocity u=10m/s at an angle θ=60∘ with the horizontal and take value of g=10m/s2. Now answer the following questions.
The radius of curvature of path of particle at the instant when the velocity vector of the particle becomes perpendicular to initial velocity vector is
View SolutionA charged particle enters a uniform magnetic field with velocity v0=4m/s perpendicular to it, the length of magnetic field is x=(√32)R, where R is the radius of the circular path of the particle in the field. Find the magnitude of charge in velocity (in m/s) of the particle when it comes out of the field.
View SolutionA charged particle enters into a uniform magnetic field with velocity v0 perpendicular to it , the length of magnetic field is x=√32R, where R is the radius of the circular path of the particle in the field .The magnitude of charge in velocity of the particle when it comes out of the field is

View SolutionA charged particle of mass m & charge q enters a zone of uniform magnetic field B with a velocity v making an angle θ with the boundary of the zone, having width d, when the particle penetrates half-way into the zone, the change in energy of the particel is
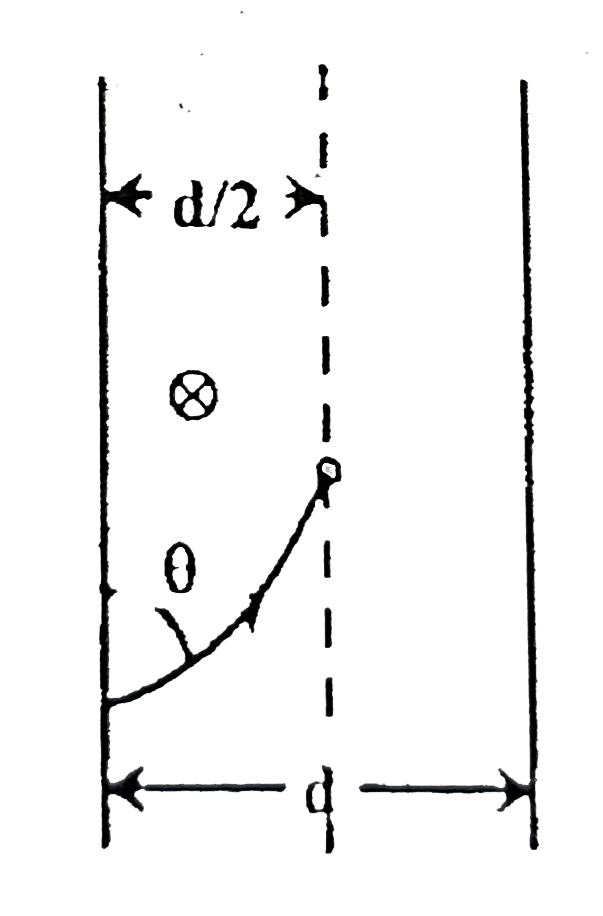
View SolutionWhen a particle is projected at some angle with the horizontal, the path of the particle is parabolic. In the process the horizontal velocity remains constant but the magnitude of vertical velocity changes. At any instant during flight the acceleration of the particle remains g in vertically downward direction. During flight at any point the path of particle can be considered as a part of circle and radius of that circle is called the radius of curvature of the path
Consider that a particle is projected with velocity u=10m/s at an angle θ=60∘ with the horizontal and take value of g=10m/s2. Now answer the following questions.
The magnitude of acceleration of particle at that instant is
View Solution
