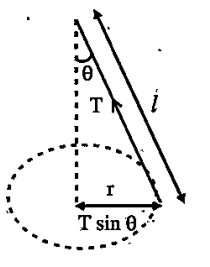
A string of length L fixed at one end carries a mass M at the other end. The string makes 2π revolutions per second around the vertical axis through the fixed end as shown in the figure what is the tension in the string ?
 Solution in Odia
Solution in Odia
Step by step video & image solution for A string of length L fixed at one end carries a mass M at the other end. The string makes 2/pi revolutions per second around the vertical axis through the fixed end as shown in the figure what is the tension in the string ? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The nucleus of an atom consists of:
View SolutionFrom which region of the electromagnetic spectrum, the radiations useful for telecommunication are selected?
View SolutionA string of length l=1m is fixed at one end carries a mass of 100gm at other end. The string makes sqrt(5)//(pi) revolutions per second about a verticle axis passing through its second end. What is the angle of inclination of the string with the vertical?
View SolutionA string of length L is fixed at one end and carries a mass M at the other end. The string makes 2/π revolution per second around the vertical axis through the fixed end as shown in the figure, then tension in the string is.

View SolutionA string of length 50cm is fixed at one end and carries a mass of 200g at the other end. The string makes 5/2π revolution per second around the verticle axis through the fixed end. Calculate (a) the tension in the string (b) the angle of incilination of the string with verticle and (c) linear velocity of the mass.
View SolutionA string of length 1 m is fixed at one end and carries a mass of 100 gm at the other end. The string makes 2/πrevolutions per second about a vertical axis through the fixed end. The angle of inclination of the string with the vertical, and the linear velocity of the mass will respec- tively be - (in M.K.S. system)
View SolutionA string of length 1m is fixed at one end and carries a mass of 1 kg at the other end. The string makes 2//pi revolutions per second around a vertical axis passing through the fixed end. Calculate (i) angle of inclination of the string with the vertical, (ii) the tension in the strong and (iii) the linear velocity of the mass.
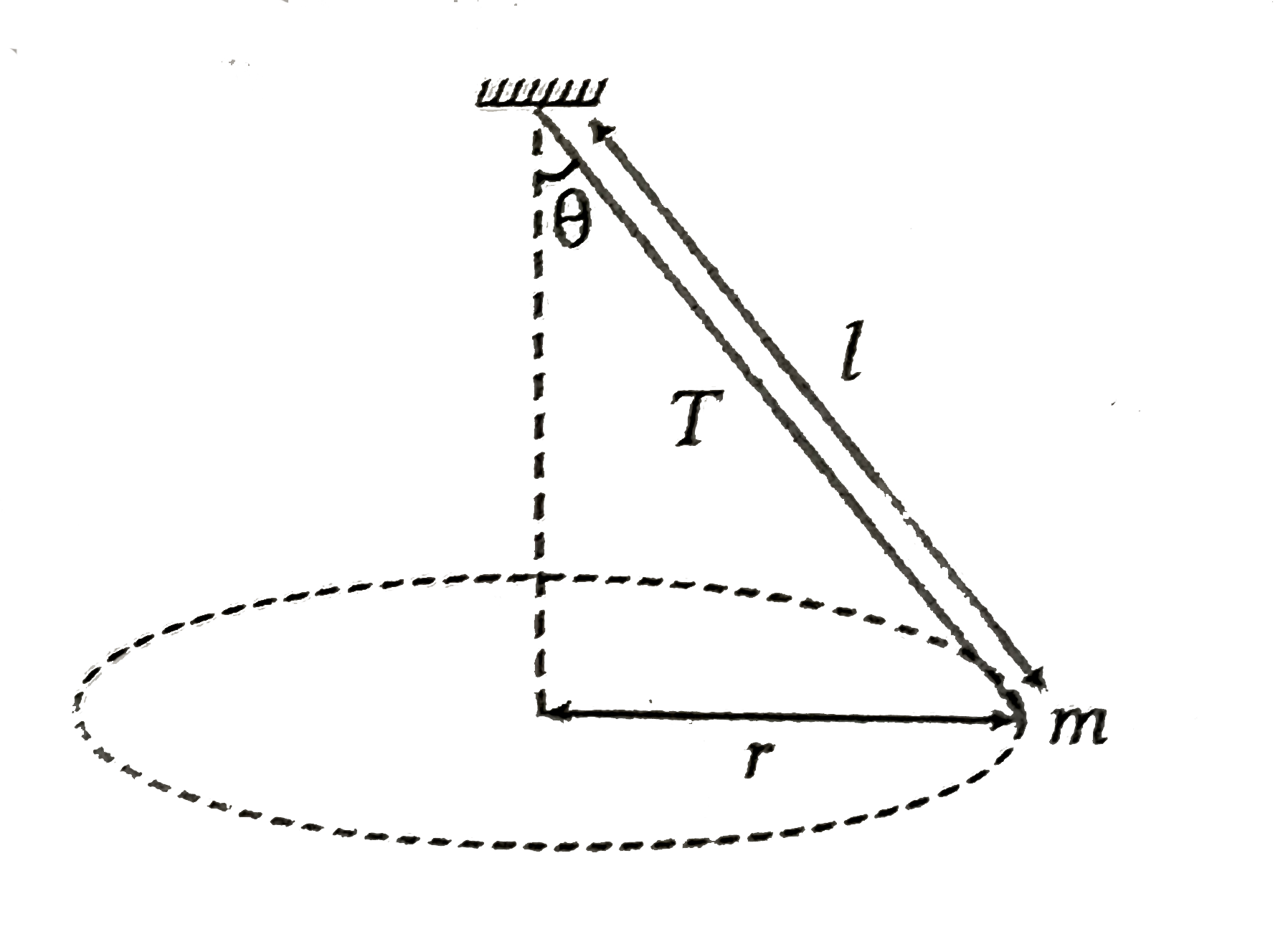
View SolutionA string of length l fixed at one end carries a mass m at the other end. The strings makes 2πrevs−1 around the axis through the fixed end as shown in the figure, the tension in the string is
View SolutionA string of length L is fixed at one end and carries a mass M at the other end. The string makes 2π rev/s around the vertical axis through the fixed end as shown in figure, then tension in string is
View SolutionA string of length 1 m is fixed at one end and carries a mass of 100 gm at the other end. The string makes (2/ pi ) revolutions per second around vertical axis through the fixed end. Calculate the tension in the string-
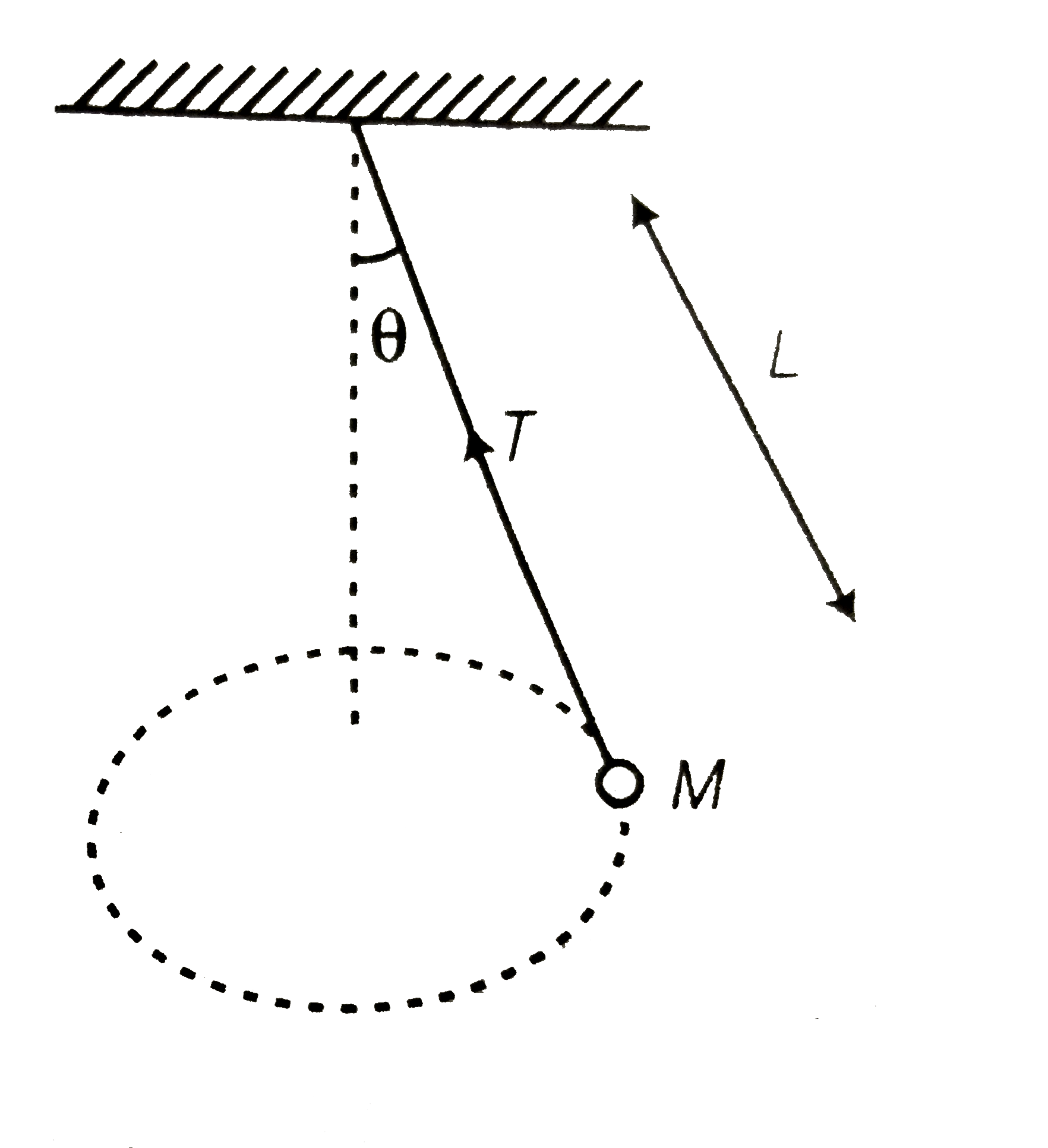
View SolutionA string of length 1 m is fixed at one end to a rigid support and carries a sphere of 100 g at the other end. The string makes 2π revolutions per second around a vertical axis through the fixed end. Calculate
The angle of inclination of the string with the vertical axis.
View SolutionA string of length 1 m is fixed at one end to a rigid support and carries a sphere of 100 g at the other end. The string makes 2π revolutions per second around a vertical axis through the fixed end. Calculate
The tension in the string and.
View SolutionA string of length L is fixed at one end and carries a mass M at the other end . The string makes 2/π revlutions per second around the vertical axis through the fixed end as shown in the figure, then tension in the string is
View SolutionA string of lenth l fixed at one end carries a mass m at the other end. The strings makes 2πrevs−1 around the axis through the fixed end as shown in the figure, the tension in the string is

View SolutionA string of length L is fixed at one end and the string makes 2π rev/s around the vertical axis through the fixed end as shwon in thefigure, then tension in the string is
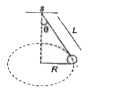
View Solution
