Deduce expression for moment of inertia about a vertical axis through the centre of gravity and perpendicular to the following cases. (I) uniform ring of mass 'm' and radius 'r'.Solution in Odia
Step by step video & image solution for Deduce expression for moment of inertia about a vertical axis through the centre of gravity and perpendicular to the following cases. (I) uniform ring of mass 'm' and radius 'r'. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A body starting from rest accelerates uniformly and attains a velocity of 20m/s in 30 seconds. Find its acceleration and the distance it covers in that time
View SolutionA proton of charge 1.6×10−19C is moving with a velocity 105m/s in auniform magnetic inductation 10−4(Wb)/m2,perpendicular to the direction of the motion of the proton ,force acting on the proton is :
View SolutionCalculate the moment of inertia of a ring having mass M , radius R and having uniform mass distribution about an axis passing through the centre of the ring and perpendicular to the plane of the ring?
View SolutionThe moment of inertia of a uniform semicircularwire of mass M and radius r about a line perpendicular to the plane of the wire through the centre is :
View SolutionThe mass of a uniform circular ring of radius 0.2m is 0.1kg . Calcuate the moment of inertia of the ring about an axis passing through its centre an perpendicular to its surface.
View SolutionState the expression for the moment of inertia of a thin uniform disc about an axis perpendicular to its plane and through its centre. Hence deduce the expression for its moment of inertia about a tangential axis perpendicular to its plane.
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionFind the moment of inertia of a uniform half-disc about an axis perpendicular to the plane and passing through its centre of mass. Mass of this disc is M and radius is R.
View SolutionMoment of inertia of a circular ring of mass m and radius r about the normal axis passing through its centre is
View SolutionThe moment of Inertia of a uniform semicircular disc of mass M and radius 'r' about a line perpendicular to the plane of the disc through the centre is :
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is I. What is the moment of inertia about its diameter ?
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the centre is ………..
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is I. What is the moment of inertia about its diameter?
View SolutionDeduce expression for moment of inertia about a vertical axis through the center of gravity and perpendicular to the cross section for uniform disc of mass 'm' and radius 'r'.
View SolutionCalculate the moment of inertia of a ring having mass M, radius Rand having uniform mass distribution about an axis passing through the centre of the ring and perpendicular to the plane of the ring?
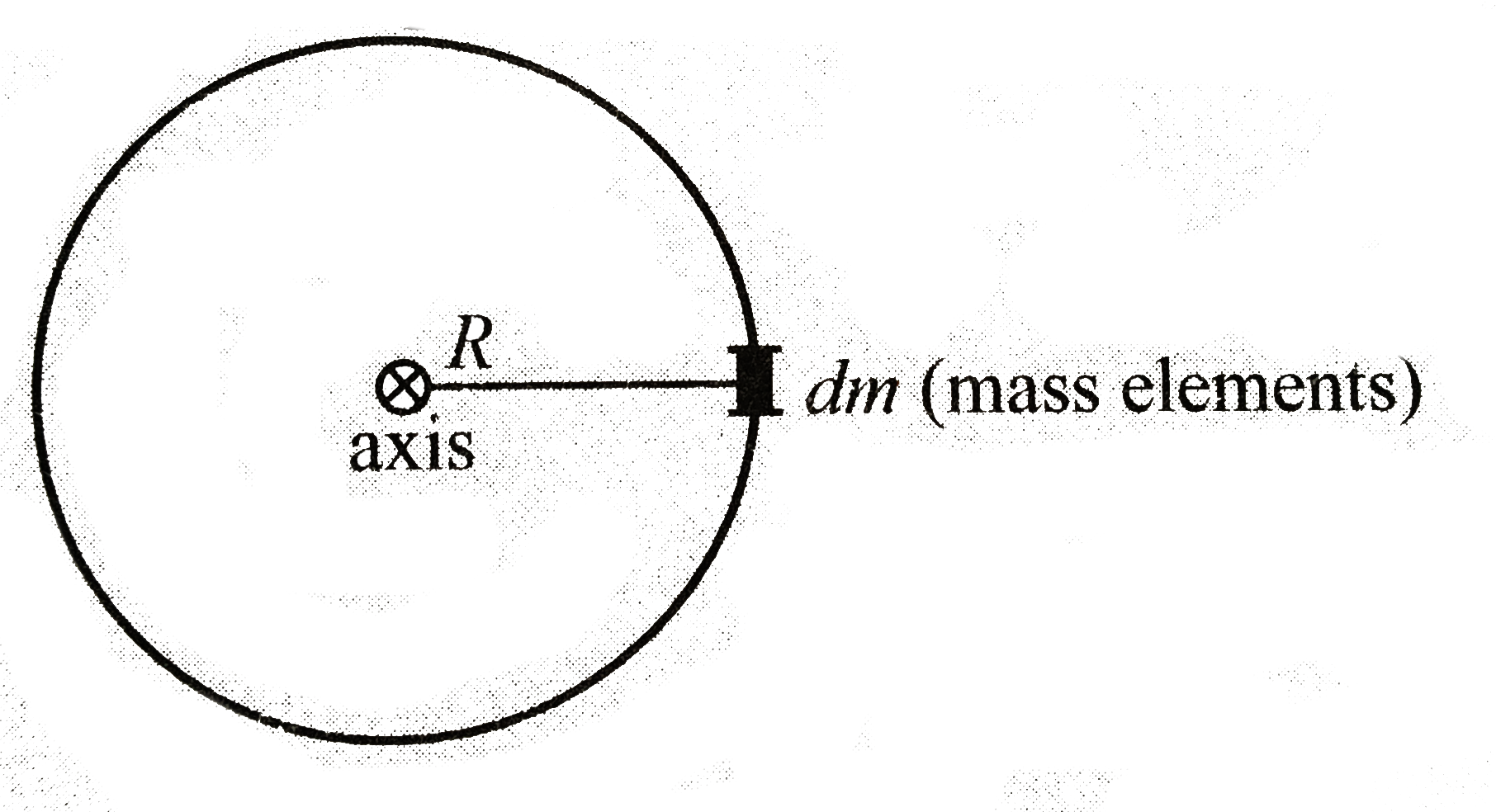
View Solution
