A stone is released from an elevator going up with an acceleration of What is the acceleration of the stone just after release ?
The correct Answer is:A, D
To solve the problem of finding the acceleration of a stone just after it is released from an elevator that is moving upwards with an acceleration of g2, we can follow these steps:
1. Understand the Situation:
- The stone is released from an elevator that is accelerating upwards with an acceleration of g2.
- The only force acting on the stone after it is released is the gravitational force.
2. Identify the Forces Acting on the Stone:
- When the stone is released, the only force acting on it is its weight, which is given by F=mg, where m is the mass of the stone and g is the acceleration due to gravity.
3. Determine the Acceleration of the Stone:
- According to Newton's second law, the net force acting on the stone will cause it to accelerate. Since the only force acting on the stone is the gravitational force, the acceleration a of the stone can be calculated using the formula:
F=ma
- Here, F=mg. Therefore, we can set up the equation:
mg=ma
- Dividing both sides by m (assuming m≠0):
g=a
- This shows that the acceleration of the stone just after release is equal to g.
4. Conclusion:
- The acceleration of the stone just after it is released from the elevator is g, which is the acceleration due to gravity.
Final Answer:
The acceleration of the stone just after release is g.
- The stone is released from an elevator that is accelerating upwards with an acceleration of g2.
- The only force acting on the stone after it is released is the gravitational force.
- When the stone is released, the only force acting on it is its weight, which is given by F=mg, where m is the mass of the stone and g is the acceleration due to gravity.
- According to Newton's second law, the net force acting on the stone will cause it to accelerate. Since the only force acting on the stone is the gravitational force, the acceleration a of the stone can be calculated using the formula:
F=ma
- Here, F=mg. Therefore, we can set up the equation:
mg=ma
- Dividing both sides by m (assuming m≠0):
g=a
- This shows that the acceleration of the stone just after release is equal to g.
- The acceleration of the stone just after it is released from the elevator is g, which is the acceleration due to gravity.
The acceleration of the stone just after release is g.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.42videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.510videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.23videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
Similar Questions
Knowledge Check
- Question 1 - Select One
Assertion : The moment after a stone is released out of an accelerated train, there is no horizontal force or acceleration on the stone.
Reason : Force on a body at a given time is determined by the situation at the location of the body at that time.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true and reason is the not correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.
A stone is released from a rising balloon accelerating upward with acceleration a. The acceleration of the stone just after the release is
View SolutionIn the arrangement shown in the figure the elevator is going up with an acceleration of g/10. If the pulley and the string are light and the pulley is smooth, the tension in the string AB is
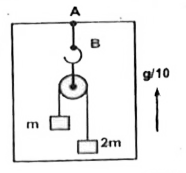 View Solution
View SolutionA block of mass m is suddenly released from the top of a spring of stiffness constant k.
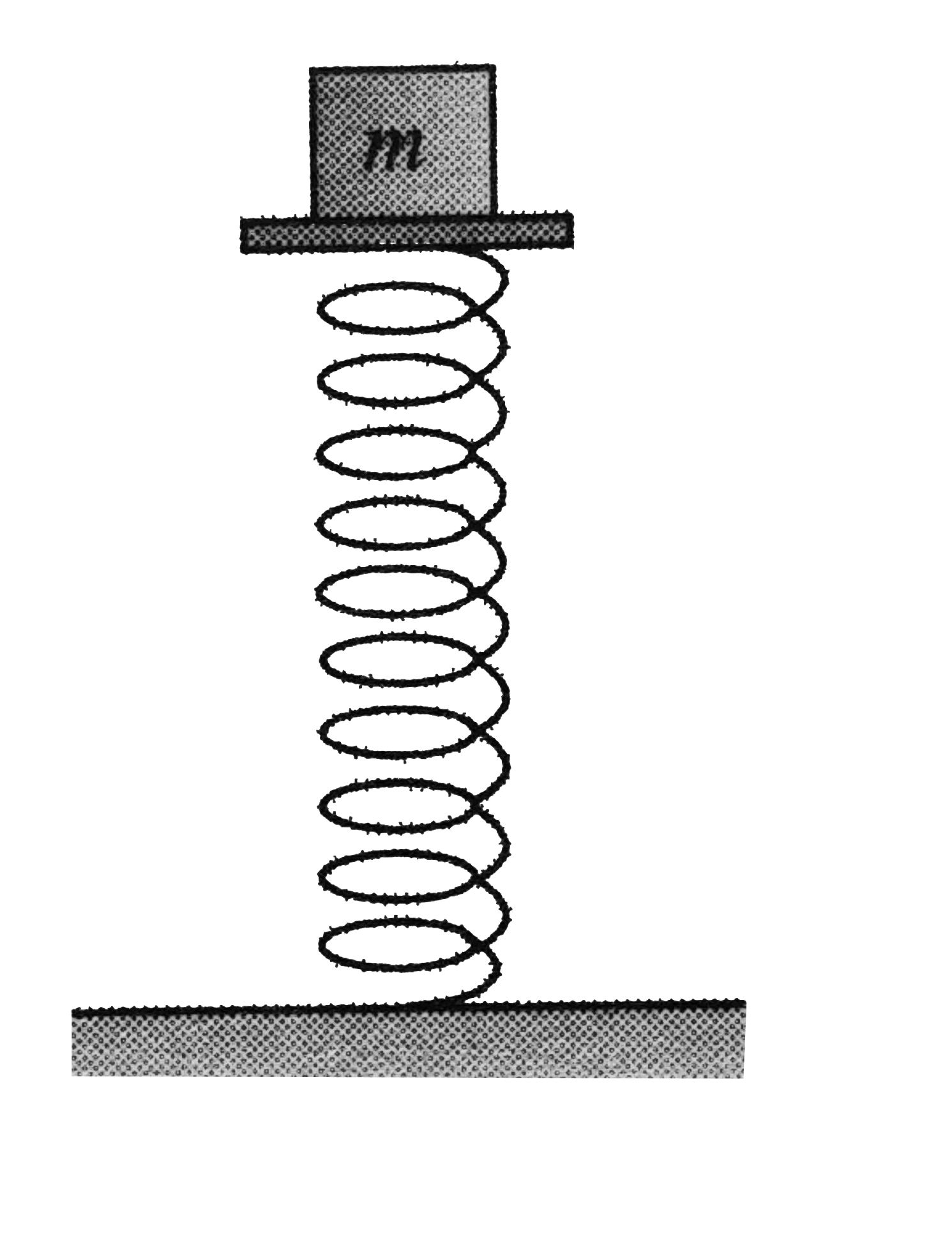
a. Find the acceleration of block just after release.
b. What is the compression in the spring at equilibrium.
c. What is the maximum compression in the spring.
d. Find the acceleration of block at maximum compression in the spring.View SolutionIn the following figure all surfaces are smooth. The system is released from rest , then
A. acceleration of wedge greater then gtanθ
B. acceleration of m is g√(1+2cos2θ)
C. acceleration of m is g
D. acceleration of wedge is gsinθView SolutionA block slides down from top of a smooth inclined plane of elevation ● fixed in an elevator going up with an acceleration a0 The base of incline hs length L Find the time taken by the block to reach the bottom
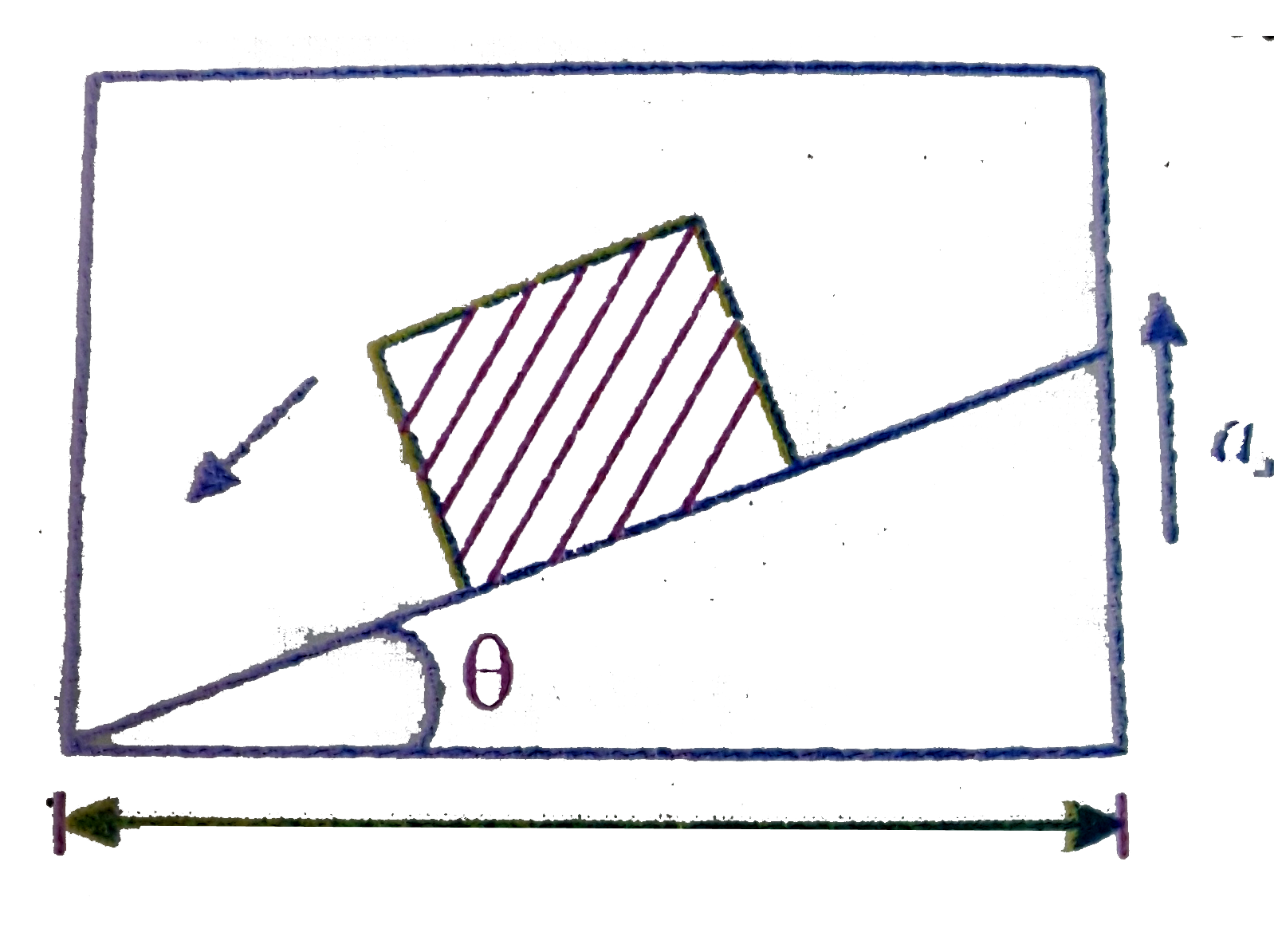 .View Solution
.View SolutionConsider the stituation shown in figure. The elevator is going up with an acceleration of 2.00 ms^-2 and the focal length of the mirror is 12.0 cm. All the surfaces are smooth and the pulley is light. The mass-pulley system is released from rest (with respect to the elevator) at t=0 when the distance of B from the mirror is 42.0 cm. Find the distance between the image of the block B and the mirror at t=0.200 s. Take g=10ms−2
 View Solution
View SolutionA stone is released from an aeroplane which is rising with upward acceleration 5ms−2 . Here g=10ms−1 The two seconds after the release , the saparation between stone and aeroplane will be
View Solution

