Find the average velocity of a particle released from rest from a height of 125m over a time interval till it strikes the ground. Take g=10m/s2.
The correct Answer is:A, B, D
To find the average velocity of a particle released from rest from a height of 125 m, we can follow these steps:
Step 1: Identify the known values
- Initial height (h) = 125 m
- Initial velocity (u) = 0 m/s (since the particle is released from rest)
- Acceleration due to gravity (g) = 10 m/s² (acting downwards)
Step 2: Determine the displacement
Since the particle is released from a height of 125 m and falls to the ground, the total displacement (s) is:
s=−125m
(The negative sign indicates that the displacement is downward.)
Step 3: Use the kinematic equation to find the time taken (t)
We can use the equation of motion:
s=ut+12at2
Substituting the known values:
−125=0⋅t+12(−10)t2
This simplifies to:
−125=−5t2
t2=1255
t2=25
Taking the square root of both sides gives:
t=5s
(Since time cannot be negative, we take the positive root.)
Step 4: Calculate the average velocity
The average velocity (v_avg) is given by the formula:
vavg=Total DisplacementTotal Time
Substituting the values we have:
vavg=−125m5s
vavg=−25m/s
Step 5: Interpret the result
The negative sign indicates that the average velocity is directed downward.
Final Answer
The average velocity of the particle is:
vavg=−25m/s
---
- Initial height (h) = 125 m
- Initial velocity (u) = 0 m/s (since the particle is released from rest)
- Acceleration due to gravity (g) = 10 m/s² (acting downwards)
Since the particle is released from a height of 125 m and falls to the ground, the total displacement (s) is:
s=−125m
(The negative sign indicates that the displacement is downward.)
We can use the equation of motion:
s=ut+12at2
Substituting the known values:
−125=0⋅t+12(−10)t2
This simplifies to:
−125=−5t2
t2=1255
t2=25
Taking the square root of both sides gives:
t=5s
(Since time cannot be negative, we take the positive root.)
The average velocity (v_avg) is given by the formula:
vavg=Total DisplacementTotal Time
Substituting the values we have:
vavg=−125m5s
vavg=−25m/s
The negative sign indicates that the average velocity is directed downward.
The average velocity of the particle is:
vavg=−25m/s
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.6
5 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.7
3 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.4
2 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
A particle is projected upwards from the roof of a tower 60 m high with velocity 20m/s. Find
(a) the average speed and
(b) average velocity of the particle upto an instant when it strikes the ground. Take g=10m/s2
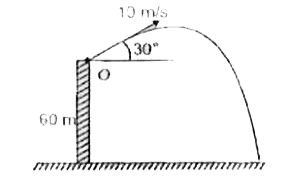
View SolutionA ball is projected from a point O as shown in figure it will strike the ground after (g = 10 m/s2 )
View SolutionA ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
View SolutionA ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
View SolutionWhat is the average velocity of a particle projected from the ground with speed u at an angle alpha with horizontal over a time interval from beginning till it strikes the ground again?
View SolutionA ball is thrown upwards from the ground with an initial speed of u. The ball is at height of 80m at two times, the time interval being 6 s. Find u. Take g=10m/s2.
View SolutionA balloon is going upwards with velocity 12m/sec it releases a packet when it is at a height of 65 m from the ground. How much time the packet will take to reach the ground (g=10m/sec2)
View SolutionAn object is thrwon vertically upwards with a velocity of 60 m/s. After what time it strike the ground ? Use g=10m/s2.
View SolutionA particle is thrown vertically up from the top of a building of height 20m with initial velocity u. A second particle is released from the same point 1 second later. If both particles reach the ground at the same instant ,3u=m/s. [Takeg=10m/s2]
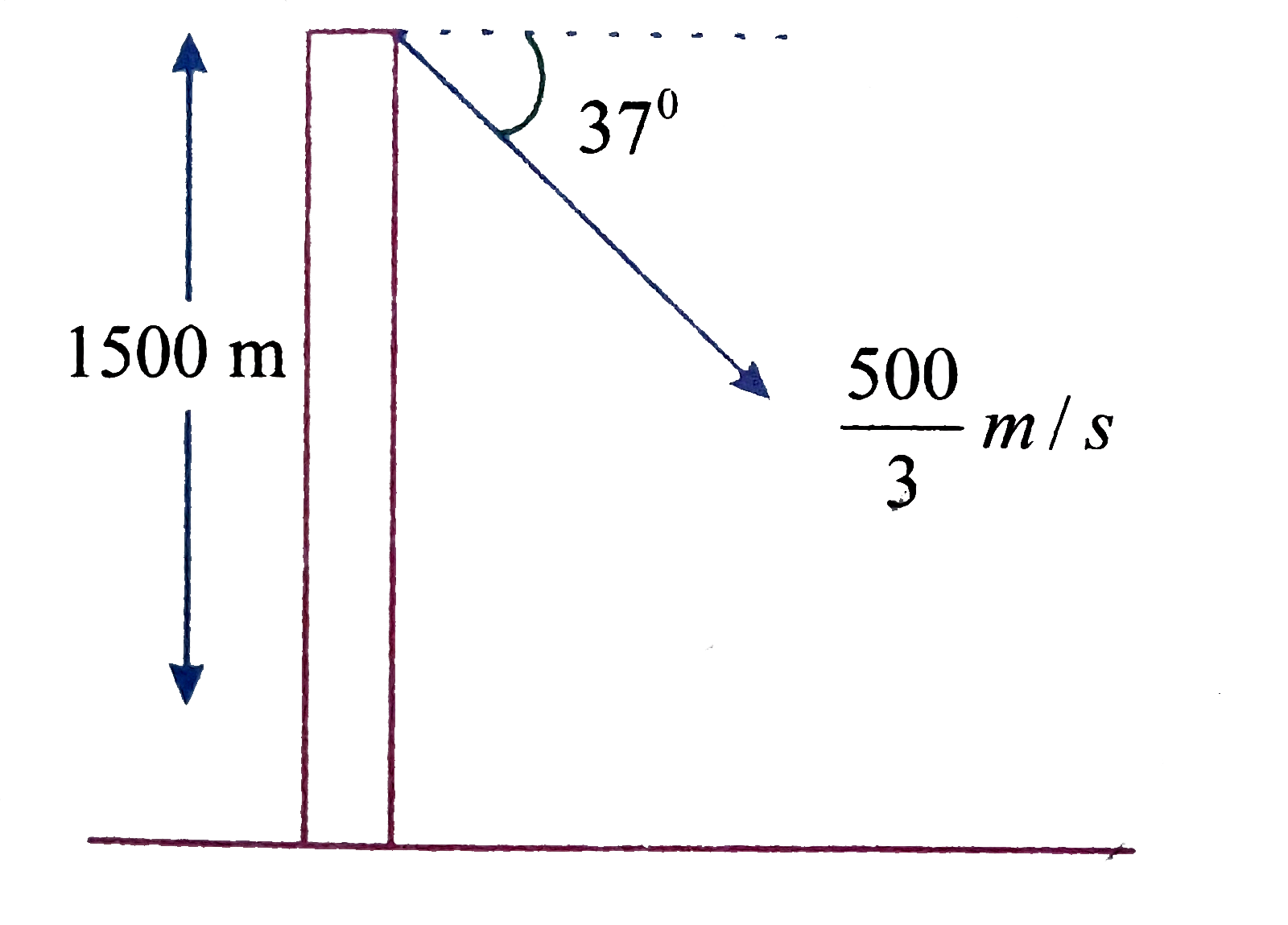
View SolutionA particle is projected from a tower as shown in figure, then find the distance from the foot of the tower where it will strike the ground. (g=10m/s2)
View Solution
