Assertion : Displacement-time graph is a parabola corresponding to straight line velocity-time graph.
Reason : lf v=u+at then s=ut+12at2
If the both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:D
To solve the given question, we will analyze both the assertion and the reason step by step.
Step 1: Understand the Assertion
The assertion states that the displacement-time graph is a parabola corresponding to a straight line velocity-time graph.
Step 2: Analyze the Velocity-Time Graph
A straight line in a velocity-time (VT) graph indicates that the velocity is either constant or changing at a uniform rate (constant acceleration). If the line is horizontal, the velocity is constant. If it has a slope, it indicates constant acceleration.
Step 3: Determine the Nature of the Displacement-Time Graph
If the velocity is constant (which corresponds to a horizontal line in the VT graph), the displacement-time (DT) graph will be a straight line. This is because displacement is calculated as:
s=ut+12at2
If acceleration a=0 (which is the case for a horizontal line in the VT graph), the equation simplifies to:
s=ut
This shows that the displacement increases linearly with time, resulting in a straight line.
If the VT graph has a constant slope (indicating constant acceleration), then the displacement-time graph will be a parabola, as the displacement will depend on the square of time due to the 12at2 term.
Step 4: Conclusion on the Assertion
Given that the assertion claims that the displacement-time graph is a parabola corresponding to a straight line velocity-time graph, and we have established that a straight line VT graph (with zero acceleration) results in a straight line DT graph, the assertion is false.
Step 5: Analyze the Reason
The reason states:
v=u+at
s=ut+12at2
These are standard equations of motion. They are indeed true and valid for uniformly accelerated motion.
Step 6: Conclusion on the Reason
The reason is true as it correctly represents the equations of motion.
Final Conclusion
- Assertion: False (displacement-time graph is a straight line for a constant velocity).
- Reason: True (the equations of motion are correct).
Answer
The correct answer is that the assertion is false, but the reason is true.
---
The assertion states that the displacement-time graph is a parabola corresponding to a straight line velocity-time graph.
A straight line in a velocity-time (VT) graph indicates that the velocity is either constant or changing at a uniform rate (constant acceleration). If the line is horizontal, the velocity is constant. If it has a slope, it indicates constant acceleration.
If the velocity is constant (which corresponds to a horizontal line in the VT graph), the displacement-time (DT) graph will be a straight line. This is because displacement is calculated as:
s=ut+12at2
If acceleration a=0 (which is the case for a horizontal line in the VT graph), the equation simplifies to:
s=ut
This shows that the displacement increases linearly with time, resulting in a straight line.
Given that the assertion claims that the displacement-time graph is a parabola corresponding to a straight line velocity-time graph, and we have established that a straight line VT graph (with zero acceleration) results in a straight line DT graph, the assertion is false.
The reason states:
v=u+at
s=ut+12at2
These are standard equations of motion. They are indeed true and valid for uniformly accelerated motion.
The reason is true as it correctly represents the equations of motion.
- Assertion: False (displacement-time graph is a straight line for a constant velocity).
- Reason: True (the equations of motion are correct).
The correct answer is that the assertion is false, but the reason is true.
Topper's Solved these Questions
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:Objective
Explore 45 Videos
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:Subjective
Explore 50 Videos
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:Exercise 6.9
Explore 6 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:(C) Chapter Exercises
Explore 45 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
Similar Questions
Displacement time graph of a particle moving in a straight line is a shown in figure.
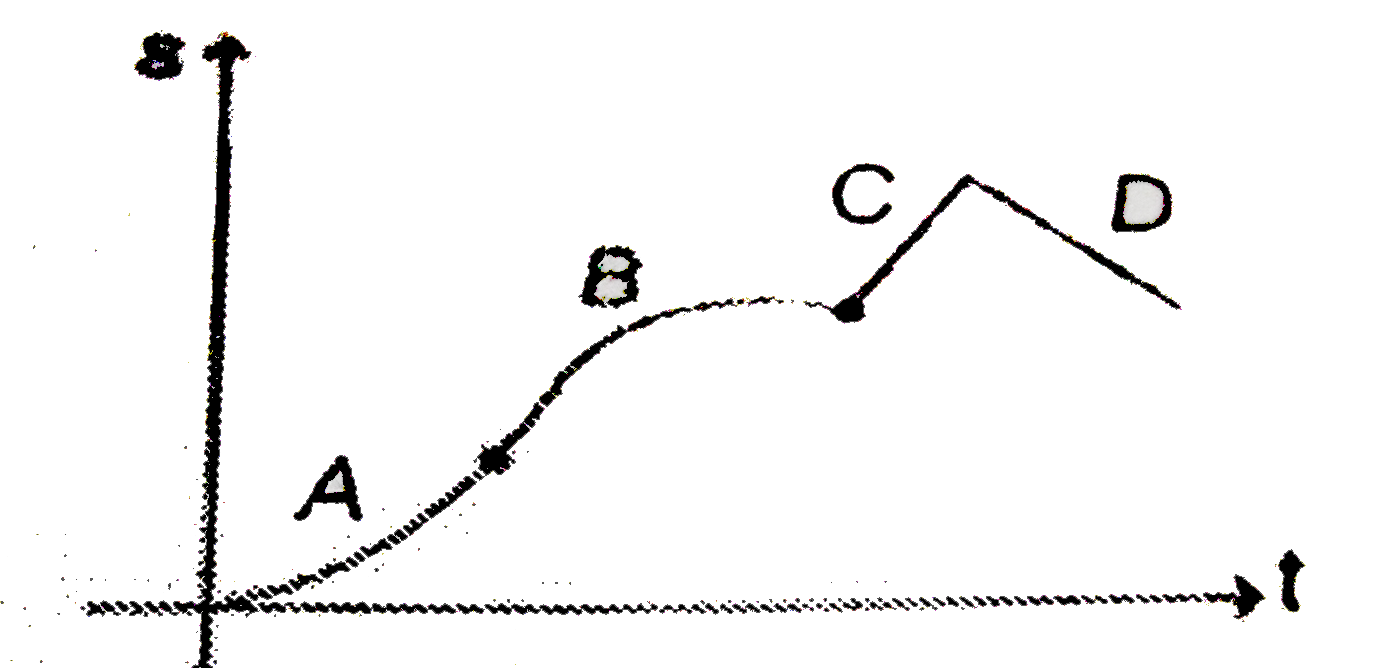
View SolutionThe displacement-time graph of a body is shown in.
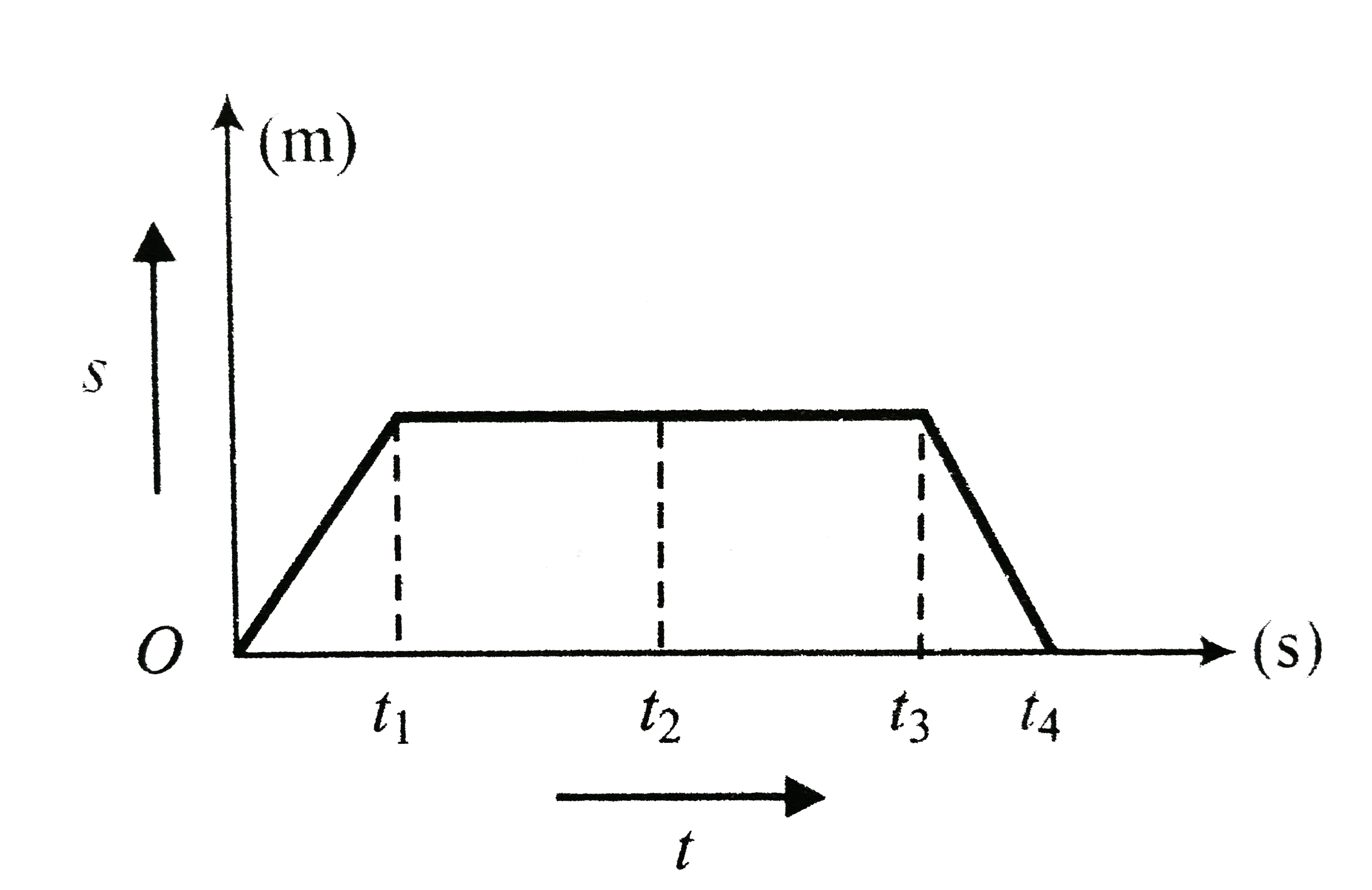 .
.
The velocity-time graph of the motion of the body will be .
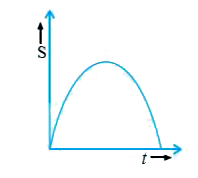
View SolutionThe graph of displacement v/s time is given below. Its corresponding velocity-time graph will be :
View SolutionThe displacement-time (x - t) graph of a body is given as
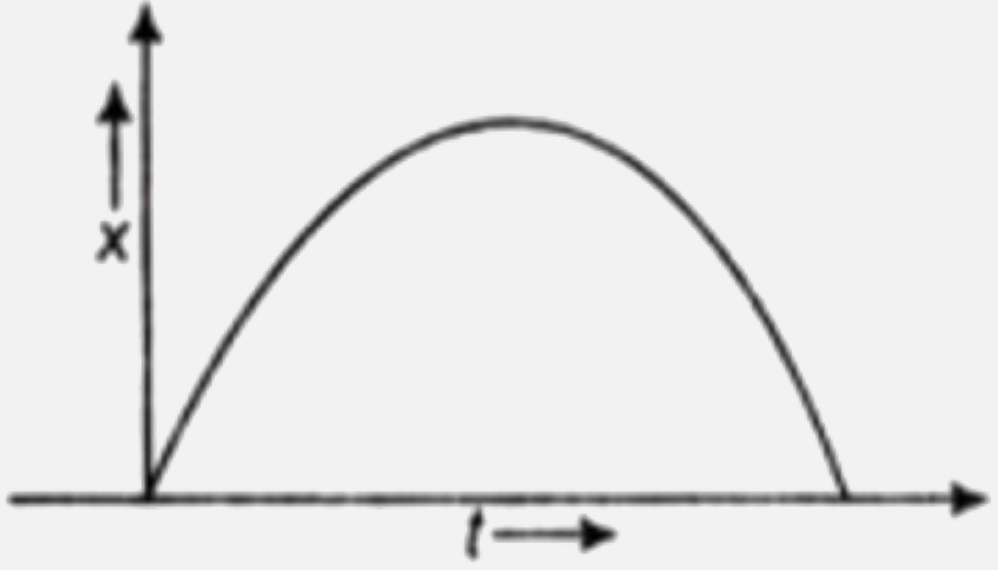
The corresponding velocity -time (v - t) graph will be
View SolutionThe displacement -time (x– t) graph of a body is given as
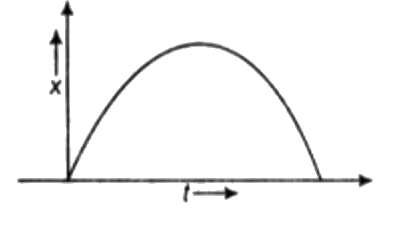
The corresponding velocity -time (v – t ) graph will be
View SolutionDraw displacement time and acceleration -time graph for the given velocity-time graph
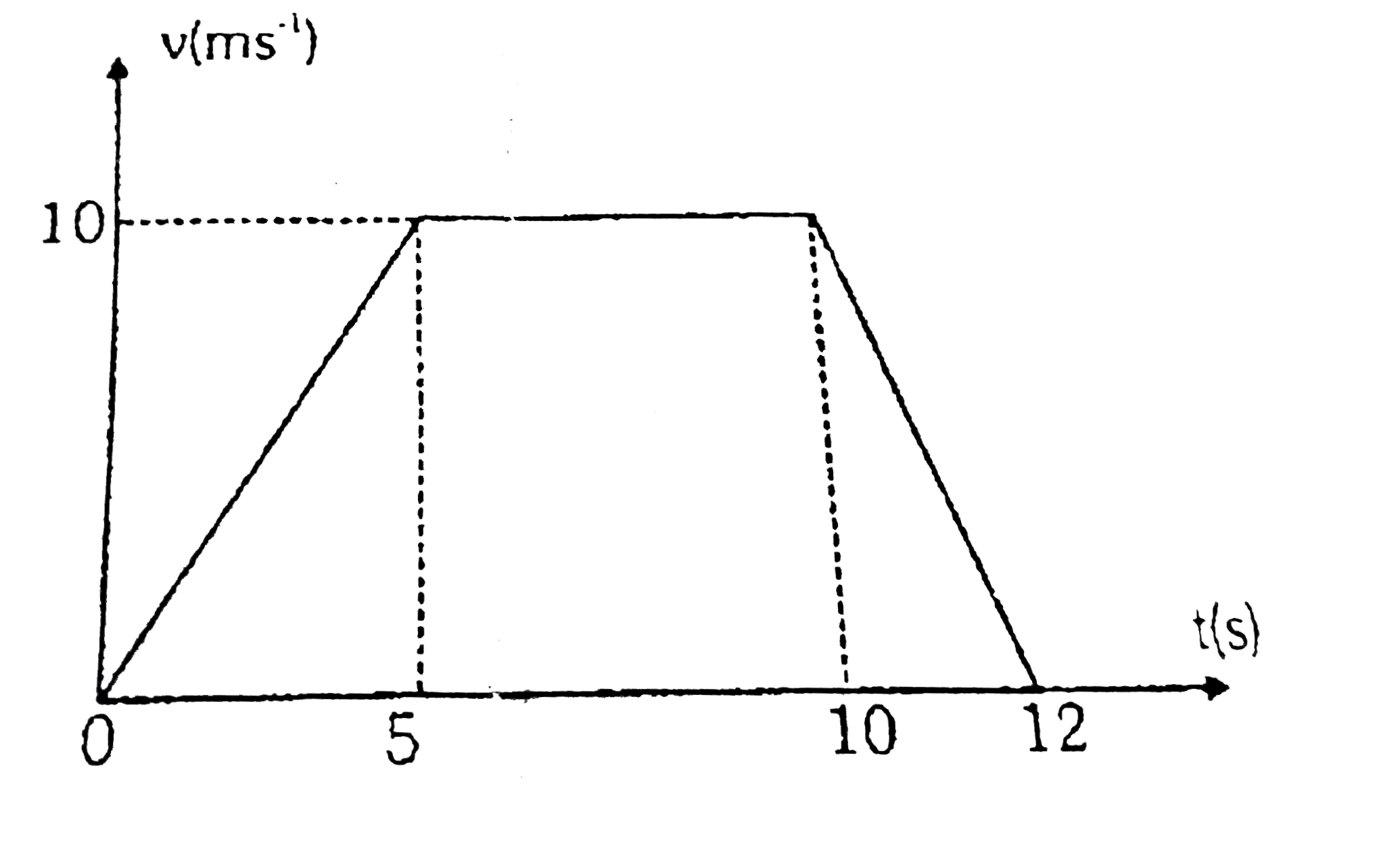
View SolutionAcceleration-time graph of a body is shown. The corresponding velocity-time graph of the same body is.

View SolutionDisplacement-time graph of a particle executing SHM is as shown

The corresponding force-time graph of the particle can be
View SolutionVelocity displacement graph of a particle moving in a straight line is as shown in figure.
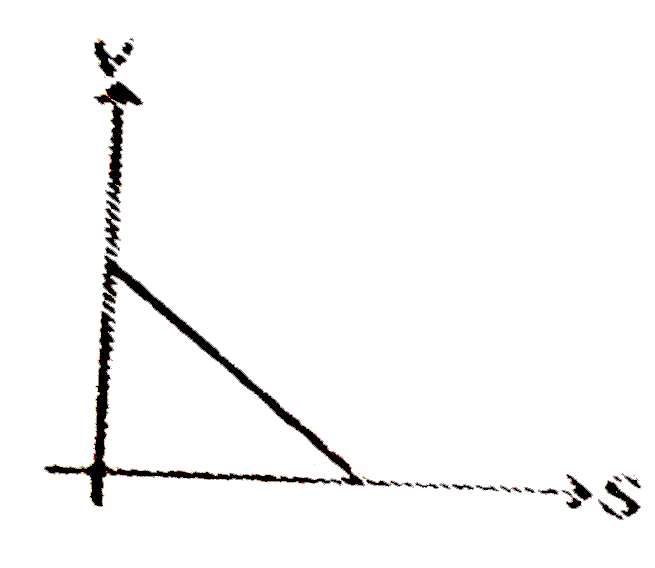
View SolutionCorresponding to given v-s graph of a particle moving in a straight line, plot a-s graph.
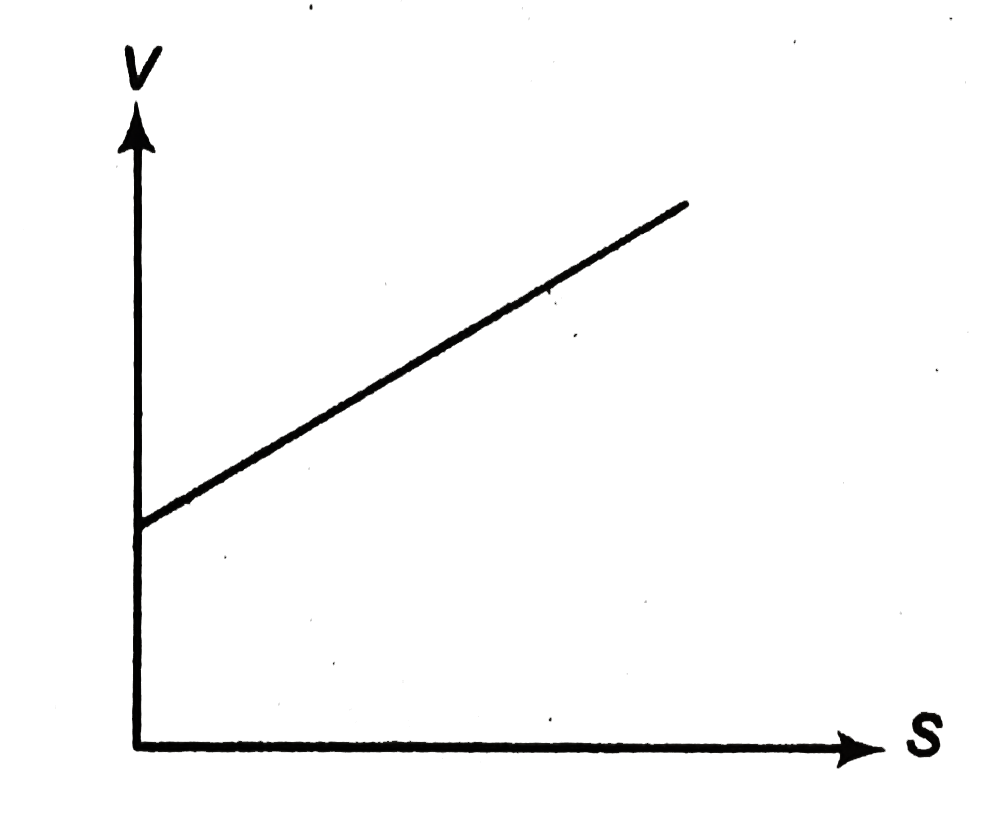
View Solution