A stone is projected from the ground in such a direction so as to hit a bird on the top of a telegraph post of height h and attains the maximum height of 2h above the ground. If at the insatant of projection, the bird were to fly away horizontally with a uniform speed, find the ratio between the horizontal velocity of bird and the horizontal component of velocity of stone, if the stone hits the bird while descending.
The correct Answer is:A, B
To solve the problem step by step, we will follow the reasoning laid out in the video transcript and derive the necessary equations.
Step 1: Determine the vertical component of the initial velocity of the stone
The stone reaches a maximum height of 2h. Using the kinematic equation for vertical motion:
hmax=u2y2g
where uy is the vertical component of the initial velocity, and g is the acceleration due to gravity. Setting hmax=2h:
2h=u2y2g
Rearranging gives:
u2y=4gh⟹uy=2√gh
Step 2: Determine the time taken to reach height h
Using the vertical motion equation again for the height h:
h=uyt1−12gt21
Substituting uy=2√gh:
h=(2√gh)t1−12gt21
Rearranging gives us a quadratic equation:
12gt21−2√ght1+h=0
Step 3: Solve the quadratic equation for t1
Using the quadratic formula t=−b±√b2−4ac2a:
Here, a=12g, b=−2√gh, and c=h:
t1=2√gh±√(2√gh)2−4⋅12g⋅hg
Calculating the discriminant:
(2√gh)2−2gh=4gh−2gh=2gh
Thus,
t1=2√gh±√2ghg
Taking the positive root (since time cannot be negative):
t1=2√gh+√2ghg
Step 4: Determine the total time of flight to reach maximum height 2h
The total time to reach maximum height 2h is given by:
t2=uyg=2√ghg=2√h√g
Step 5: Calculate the ratio of the horizontal velocities
Let v0 be the horizontal velocity of the bird and ux be the horizontal component of the stone's velocity. The horizontal distance traveled by both the bird and the stone is the same when the stone hits the bird:
x=v0t2
x=ux(t2−t1)
Setting these equal gives:
v0t2=ux(t2−t1)
Rearranging for the ratio:
v0ux=t2−t1t2
Step 6: Calculate t1/t2
From previous calculations, we can substitute t1 and t2 into the ratio.
After substituting and simplifying, we find:
v0ux=1−t1t2
Final Result
After substituting the values and simplifying, we find that:
v0ux=2√2
x=ux(t2−t1)
Topper's Solved these Questions
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 More Than One Correct
8 Videos
- MOTION IN A PLANEBOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
Similar Questions
A stone is projected from the ground with a velocity of 20 m/s at angle 30∘ with the horizontal. After one second it clears a wall then find height of the wall. (g=10ms−2)
View SolutionA particle is projected from the ground with velocity u making an angle θ with the horizontal. At half of its maximum heights,
View Solution
Knowledge Check
If R and H represent horizontal range and maximum height of the projectile, then the angle of projection with the horizontal is
Atan−1(HR)Btan−1(2HR)Ctan−1(4HR)Dtan−1(4HH)
A body is thrown horizontally from the top of a tower. It reaches the ground after 4s at an angle 45° to the ground. The velocity of projection is
View SolutionA body of mass m is projected from ground with speed u at an angle θ with horizontal. The power delivered by gravity to it at half of maximum height from ground is
View SolutionA stone is projected vertically up from the ground with velocity 40ms−1 . The interval of time between the two instants at which the stone is at a height of 60 m above the ground is (g=10ms−2)
View SolutionA body is projected horizontally from the top of a tower with initial velocity 18ms−1. It hits the ground at angle 45∘. What is the vertical component of velocity when it strikes the ground ?
View Solution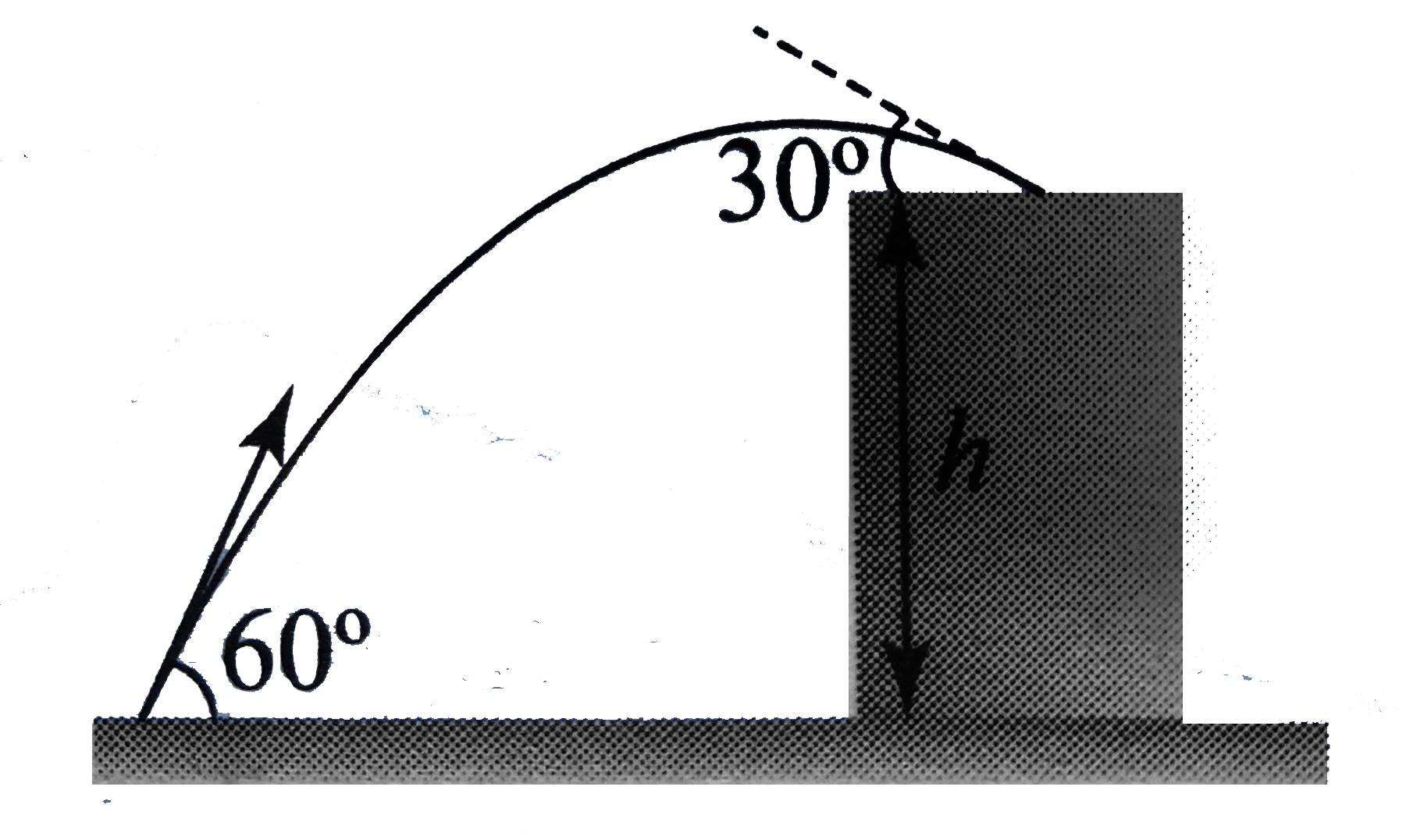
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View Solution
A stone projected at an angle of 60∘ from the ground level strikes at an angle of 30∘ on the roof of a building of height h. Then the speed of projection of the stone is:
View SolutionAssertion : Two projectile have maximum heights 4H and H respectively. The ratio of their horizontal components of velocities should be 1:2 for their horizontal ranges to be same.
Reason : Horizontal range = horizontal component of velocity xx time of flight.
View Solution
