Question

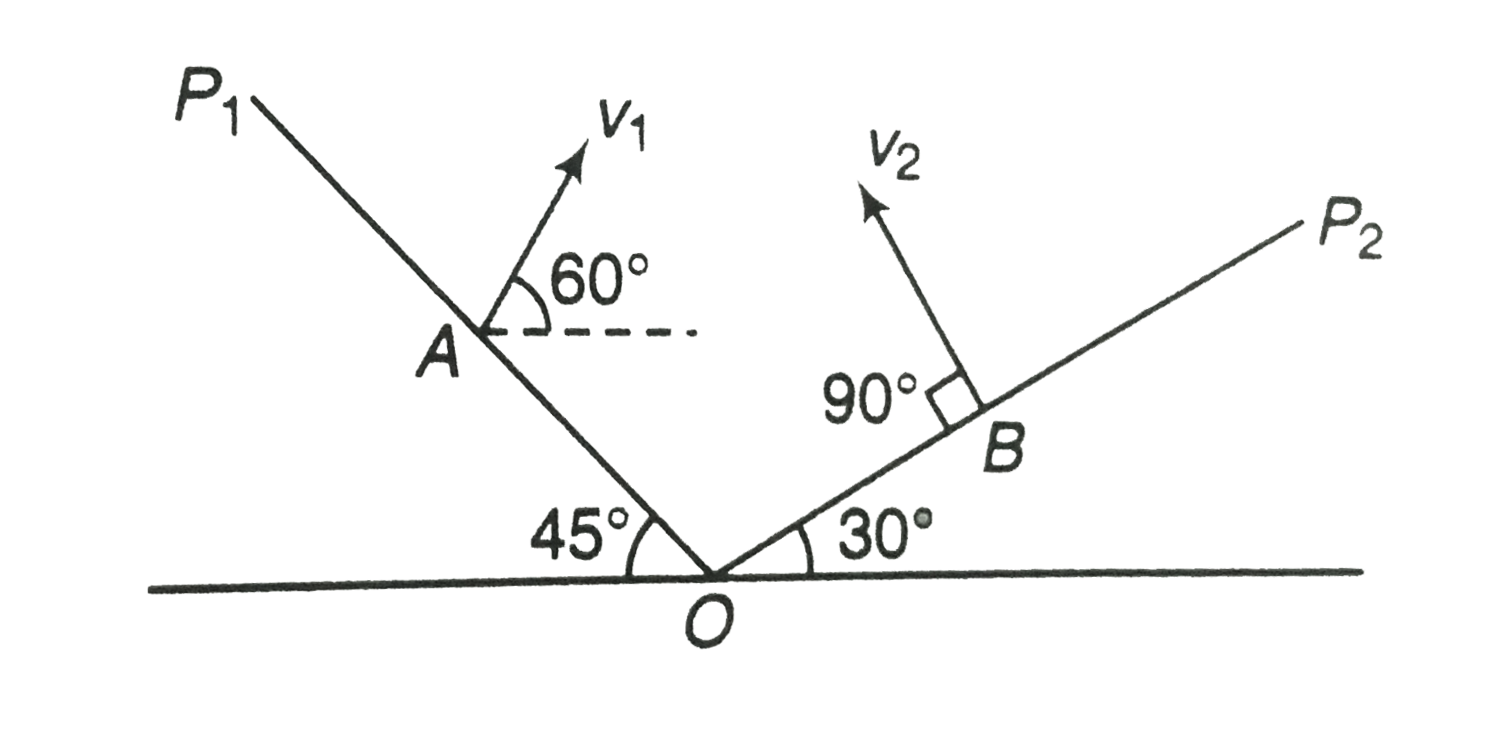
A particle is projected from an inclined plane $O{P}_1$ from A with velocity $v_1=8m{s}^{-1}$ at an angle ${60}^{\circ }$ with horizontal. An another particle is projected at the same instant from B with velocity $v_2=16m{s}^{-1}$ and perpendicular to the plane $O{P}_2$ as shown in figure. After time $10\left(\sqrt{3}\right)$ s there separation was minimum and found to be 70m. Then find distance AB.

Answer

B

Explanation

`|v_(21x)| = (v_1+v_2)cos 60^@ = 12m//s`
`|v_(21y)| = (v_2-v_1) sin 60^@ = 4(sqrt3) m//s`
`:. v_(21) = sqrt((12)^2+(4sqrt(3))^2) = (sqrt(192)) m//s`

`BC = (v_(21))t = 240m`
`AC = 70m`
Hence, `AB = sqrt((240)^2 + (70)^2) = 250m`.