A particle is released from a certain height H=400m. Due to the wind, the particle gathers the horizontal velocity component vx=aywhere a =(√5)s−1 and y is the vertical displacement of the particle from the point of release, then find
(a) the horizontal drift of the particle when it strikes the ground ,
(b) the speed with which particle strikes the ground.
The correct Answer is:A, B
To solve the problem step by step, we will break it down into two parts as specified in the question.
Given Data:
- Height of release, H=400m
- Horizontal velocity component, vx=ay where a=√5s−1
- Acceleration due to gravity, g=10m/s2
Part (a): Finding the Horizontal Drift of the Particle
1. Determine the time taken to reach the ground:
The vertical motion of the particle can be described by the equation:
H=12gt2
Substituting the known values:
400=12×10×t2
Simplifying gives:
400=5t2⟹t2=4005=80⟹t=√80=8.94s
2. Calculate the horizontal drift:
The horizontal velocity vx is given by:
vx=ay=√5y
The horizontal drift x can be found by integrating the horizontal velocity over time:
dx=vxdt=√5ydt
Since y=H−12gt2, we can express y in terms of t:
y=400−5t2
Now, substituting y into the equation for vx:
vx=√5(400−5t2)
To find the drift x, we integrate:
x=∫t0vxdt=∫8.940√5(400−5t2)dt
Solving this integral gives:
x=√5(400t−53t3)8.940
Evaluating this at t=8.94:
x=√5(400×8.94−53×(8.94)3)
After calculating, we find:
x≈2.66km
Part (b): Finding the Speed with which the Particle Strikes the Ground
1. Calculate the vertical component of the velocity when it strikes the ground:
The vertical velocity vy can be calculated using:
vy=gt=10×8.94=89.4m/s
2. Calculate the horizontal component of the velocity at the moment of impact:
Using the previously calculated vx:
vx=√5×400=400√5m/s
3. Calculate the resultant speed at impact:
The resultant speed v is given by:
v=√v2x+v2y
Substituting the values:
v=√(400√5)2+(89.4)2
After calculating, we find:
v≈400.89m/s
Final Answers:
(a) The horizontal drift of the particle when it strikes the ground is approximately 2.66km.
(b) The speed with which the particle strikes the ground is approximately 400.89m/s.
- Height of release, H=400m
- Horizontal velocity component, vx=ay where a=√5s−1
- Acceleration due to gravity, g=10m/s2
The vertical motion of the particle can be described by the equation:
H=12gt2
Substituting the known values:
400=12×10×t2
Simplifying gives:
400=5t2⟹t2=4005=80⟹t=√80=8.94s
The horizontal velocity vx is given by:
vx=ay=√5y
The horizontal drift x can be found by integrating the horizontal velocity over time:
dx=vxdt=√5ydt
Since y=H−12gt2, we can express y in terms of t:
y=400−5t2
Now, substituting y into the equation for vx:
vx=√5(400−5t2)
To find the drift x, we integrate:
x=∫t0vxdt=∫8.940√5(400−5t2)dt
Solving this integral gives:
x=√5(400t−53t3)8.940
Evaluating this at t=8.94:
x=√5(400×8.94−53×(8.94)3)
After calculating, we find:
x≈2.66km
The vertical velocity vy can be calculated using:
vy=gt=10×8.94=89.4m/s
Using the previously calculated vx:
vx=√5×400=400√5m/s
The resultant speed v is given by:
v=√v2x+v2y
Substituting the values:
v=√(400√5)2+(89.4)2
After calculating, we find:
v≈400.89m/s
(a) The horizontal drift of the particle when it strikes the ground is approximately 2.66km.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 More Than One Correct8videos
PROJECTILE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery32videos
MOTION IN A PLANE
- BOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer8videos
PROPERTIES OF MATTER
Similar Questions
In the figure shown, find
(a) the time when the particle strikes the ground at P.
(b) the horizontal distance QP
(c) velocity of the particle at P.
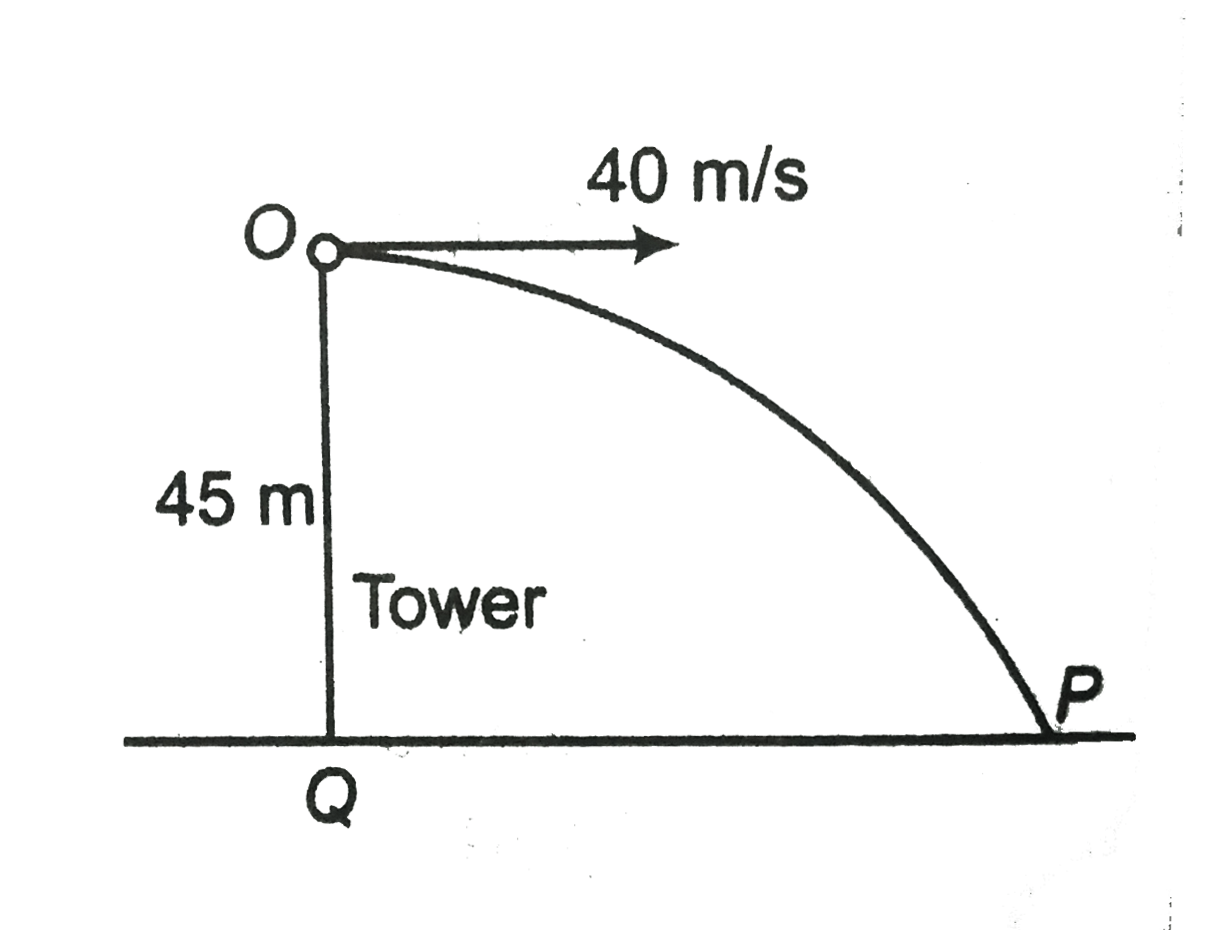 View Solution
View SolutionA particle is projected from a tower as shown in figure, then find the distance from the foot of the tower where it will strike the ground. (g=10m/s2)
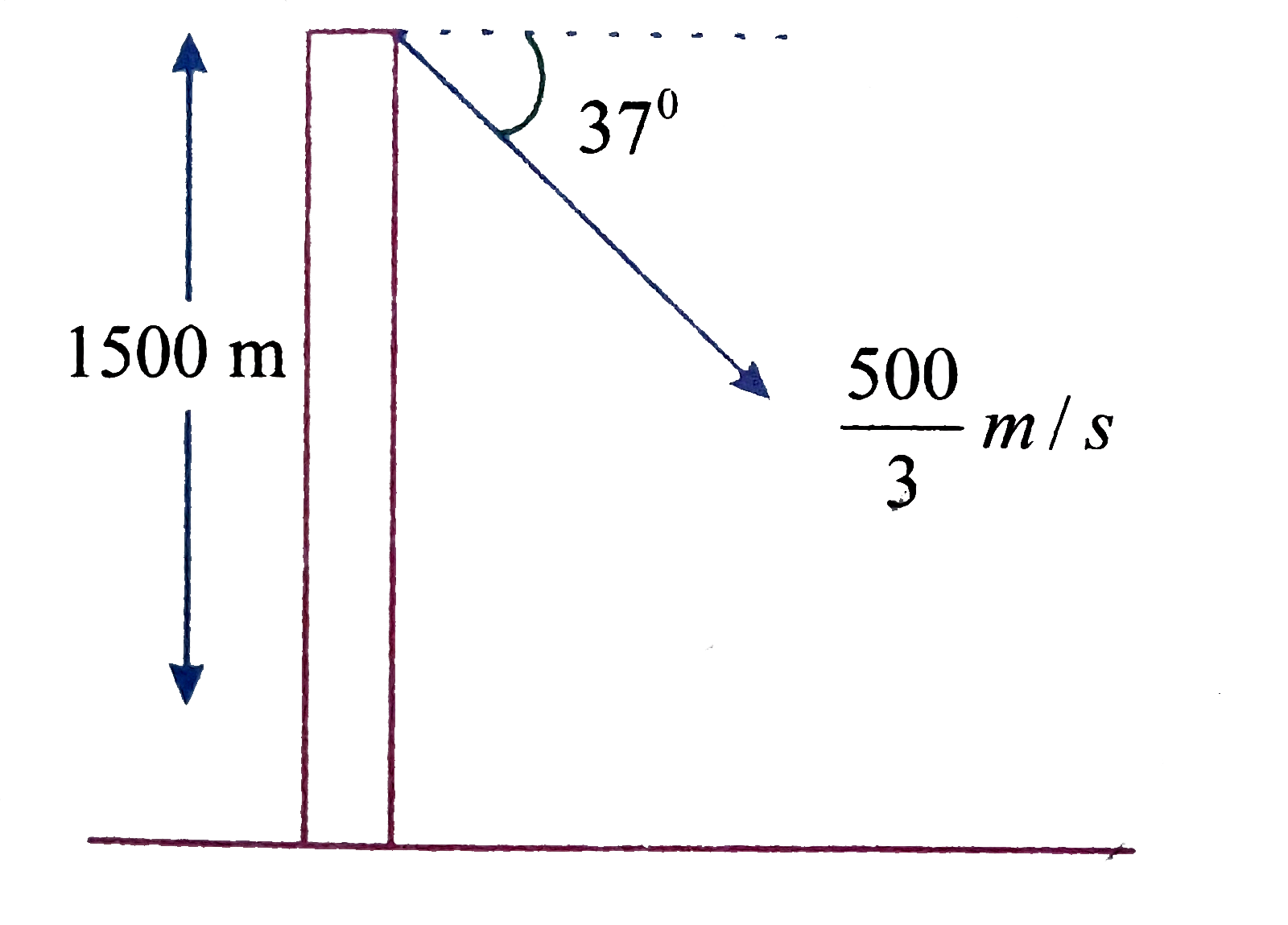 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
in the question number 4, if the horizontal displacement of the particle as seen by an observer on the ground is zero, the speed of the box with respect to the ground at the instant when particle was projected is
AucosαBusinαcosθCucos(α+θ)cosθDusinθsinαcosθ

A particle is projected from the horizontal x-z plane, in vertical x-y plane where x-axis is horizontal and positive y-axis vertically upwards. The graph of y coordinate of the particle v/s time is as shown . The range of the particle is √3. Then the speed of the projected particle is:View SolutionWhat is the average velocity of a particle projected from the ground with speed u at an angle alpha with horizontal over a time interval from beginning till it strikes the ground again?
View SolutionA heavy particle hanging from a string of length I is projected horizontally with speed √gl . Find the speed of the particle at the point where the tension in the string equals weight of the particle.
View SolutionParticle is dropped form the height of 20m from horizontal ground. There is wind blowing due to which horizontal acceleration of the particles becomes 6ms−2. Find the horizontal displacement of the particle till it reaches ground.
View SolutionFind the average velocity of a particle released from rest from a height of 125m over a time interval till it strikes the ground. Take g=10m/s2.
View SolutionA bomber plane moves horizontally with a speed of 500m/s and a bomb released from it, strikes the ground in 10s. The angle with horizontally at which it strikes the ground will be
View SolutionA particle is projected horizontally with a speed u from the top of plane inclined at an angle θ with the horizontal. How far from the point of projection will the particle strike the plane ?
 View Solution
View Solution

