If a planet was suddenly stopped in its orbit supposed to be circular, show that it would fall onto the sun in a time √28 times the period of the plant's revolution.
To solve the problem of how long it would take for a planet to fall into the Sun if it were suddenly stopped in its circular orbit, we can follow these steps:
1. Understanding the Initial Conditions:
- The planet is initially in a circular orbit around the Sun with radius R.
- The gravitational force provides the necessary centripetal force to keep the planet in orbit.
2. Identify the Semi-Major Axis of the Elliptical Path:
- When the planet is suddenly stopped, it will start to fall towards the Sun along an elliptical path.
- The semi-major axis a of this elliptical path can be determined. Since the planet was at a distance R from the Sun and is now falling towards it, the semi-major axis a is R2.
3. Relate the Time Period of Circular and Elliptical Orbits:
- The time period T of a planet in a circular orbit is given by:
T∝√R3
- For an elliptical orbit, the time period T′ is given by:
T′∝√a3
- Since a=R2, we can express T′ in terms of T.
4. Calculate the Time Period of the Elliptical Orbit:
- Substitute a=R2 into the equation for T′:
T′∝√(R2)3=√R38=12√2√R3
- Since T∝√R3, we have:
T′=T2√2
5. Determine the Time Taken to Fall into the Sun:
- The time taken for the planet to fall into the Sun is half of the time period of the elliptical orbit because it will cover half the elliptical path to reach the Sun.
- Therefore, the time t taken to fall into the Sun is:
t=T′2=12⋅T2√2=T4√2
6. Express the Final Result:
- To express this in terms of the period T:
t=√28T
Conclusion:
The time taken for the planet to fall into the Sun after being stopped in its orbit is √28 times the period of the planet's revolution.
- The planet is initially in a circular orbit around the Sun with radius R.
- The gravitational force provides the necessary centripetal force to keep the planet in orbit.
- When the planet is suddenly stopped, it will start to fall towards the Sun along an elliptical path.
- The semi-major axis a of this elliptical path can be determined. Since the planet was at a distance R from the Sun and is now falling towards it, the semi-major axis a is R2.
- The time period T of a planet in a circular orbit is given by:
T∝√R3
- For an elliptical orbit, the time period T′ is given by:
T′∝√a3
- Since a=R2, we can express T′ in terms of T.
- Substitute a=R2 into the equation for T′:
T′∝√(R2)3=√R38=12√2√R3
- Since T∝√R3, we have:
T′=T2√2
- The time taken for the planet to fall into the Sun is half of the time period of the elliptical orbit because it will cover half the elliptical path to reach the Sun.
- Therefore, the time t taken to fall into the Sun is:
t=T′2=12⋅T2√2=T4√2
- To express this in terms of the period T:
t=√28T
The time taken for the planet to fall into the Sun after being stopped in its orbit is √28 times the period of the planet's revolution.
Topper's Solved these Questions
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:Exercise 13.1
Explore 5 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:Exercise 13.2
Explore 9 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:Solved Examples
Explore 16 Videos
- GENERAL PHYSICSBook:DC PANDEY ENGLISHChapter:GENERAL PHYSICSExercise:INTEGER_TYPE
Explore 2 Videos
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:INTEGER_TYPE
Explore 10 Videos
Similar Questions
The distance of the planet Jupiter from the Sun is 5.2 times that of the Earth. Find the period of Jupiter's revolution around the Sun.
View SolutionConsider a hypothetical solar system, which has two identical massive suns each of mass M and radius r, seperated by a seperation of 2√3R (centre to centre). (R gtgtgtr). These suns are always at rest. There is only one planet in this solar system having mass m. This planet is revolving in circular orbit of radius R such that centre of the orbit lies at the mid point of the line joining the centres of the sun and plane of the orbit is perpendicular to the line joining the centres of the sun. Whole situation is shown in the figure.
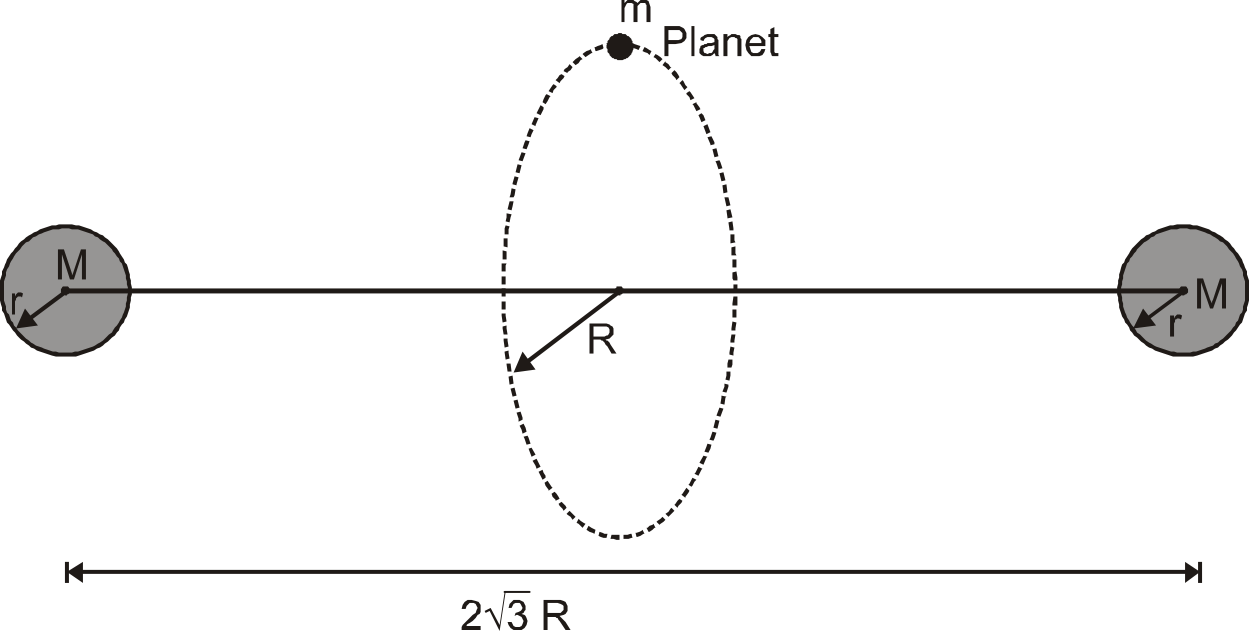
Q. Average force on the planet in half revolution is
View Solution
Knowledge Check
A planet revolves around the sun in an elliptical orbit. Suppose that the gravitational attraction between the sun and the planet suddenly disappear then the planet would move
Atowards the sun in a spiral pathBaway from the sun along a straight path with continuously increasing speedCwith constant speed along a tangential path to the oribtDtowards the sun along a straight path with constant speed
A planet of mass m is moving in an elliptical orbit about the sun (mass of sun = M). The maximum and minimum distances of the planet from the sun are r1 and r2 respectively. The period of revolution of the planet wil be proportional to :
View SolutionA planet is revolving around the sun. its distance from the sun at apogee is rA and that at perigee is rp. The masses of planet and sun are 'm' and M respectively, VA is the velocity of planet at apogee and VP is at perigee respectively and T is the time period of revolution of planet around the sun, then identify the wrong answer.
View SolutionA planet of small mass m moves around the sun of mass M along an elliptrical orbit such that its minimum and maximum distance from sun are r and R respectively. Its period of revolution will be:
View SolutionLength of a year on a planet is the duration in which it completes one revolution around the sun. Assume path of the planet known as orbit to be circular with sun at the centre. The length T of a year of a planet orbiting around the sun in circular orbit depends on universal gravitational constant G, mass ms of the sun and radius r of the orbit. if T∝Gambsrc find value of a+b+2c.
View SolutionAn artificial satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the earth.
(i) Determine the height of the satellite above the earth's surface.
(ii) If the satellite is stopped suddenly in its orbit and allowed to fall freely onto the earth, find the speed with which it hits the surface of the earth.
View SolutionAn artificial satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the earth.
(i) Determine the height of the satellite above the earth's surface.
(ii) If the satellite is stopped suddenly in its orbit and allowed to fall freely onto the earth, find the speed with which it hits the surface of the earth.
View SolutionThe planet Mars has two moons. Phobos and Delmos (i) phobos has period 7 hours, 39 minutes and an orbital radius of 9.4×103km. Calculate the mass of Mars. (ii) Assume that Earth and mars move in a circular orbit around the sun, with the martian orbit being 1.52 times the orbital radius of the Earth. What is the length of the martian year in days? (G=6.67×10−11Nm2kg−2)
View Solution
