A particle oscillates simple harmonic motion with a period of 16s. Two second after crossing the equilibrium position its velocity becomes 1m/s. The amplitude is
π4m
8√2πm
8πm
4√2πm
The correct Answer is:B
ω=2πT=2π16=π8rad/s
At t=0, particle crosses the means position. Hence its velocity is maximum. So, velocity as a function of time can be written as
v=vmaxcosωt
or v=ωAcosωt
∴1=(π8)Acos(π8)(2)
=(π8√2)A
∴A=8√2πm
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct8videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Comprehension2videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Subjective39videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
Similar Questions
A simple pendulum with a bob of mass m is suspended from the roof of a car moving with horizontal acceleration a
02:07View SolutionA 2kg block hangs without vibrating at the bottom end of a spring with a force constant of 400N/m. The top end of the spring is attached to the ceiling of an elevator car. The car is rising with an upward acceleration of 5m/s2 when the acceleration suddenly ceases at time t=0 and the car moves upward with constant speed (g=10m/s2)
What is the angular frequencyof the block after the acceleration ceases ?02:15View SolutionFind the period of oscillation of the system shown in figure.
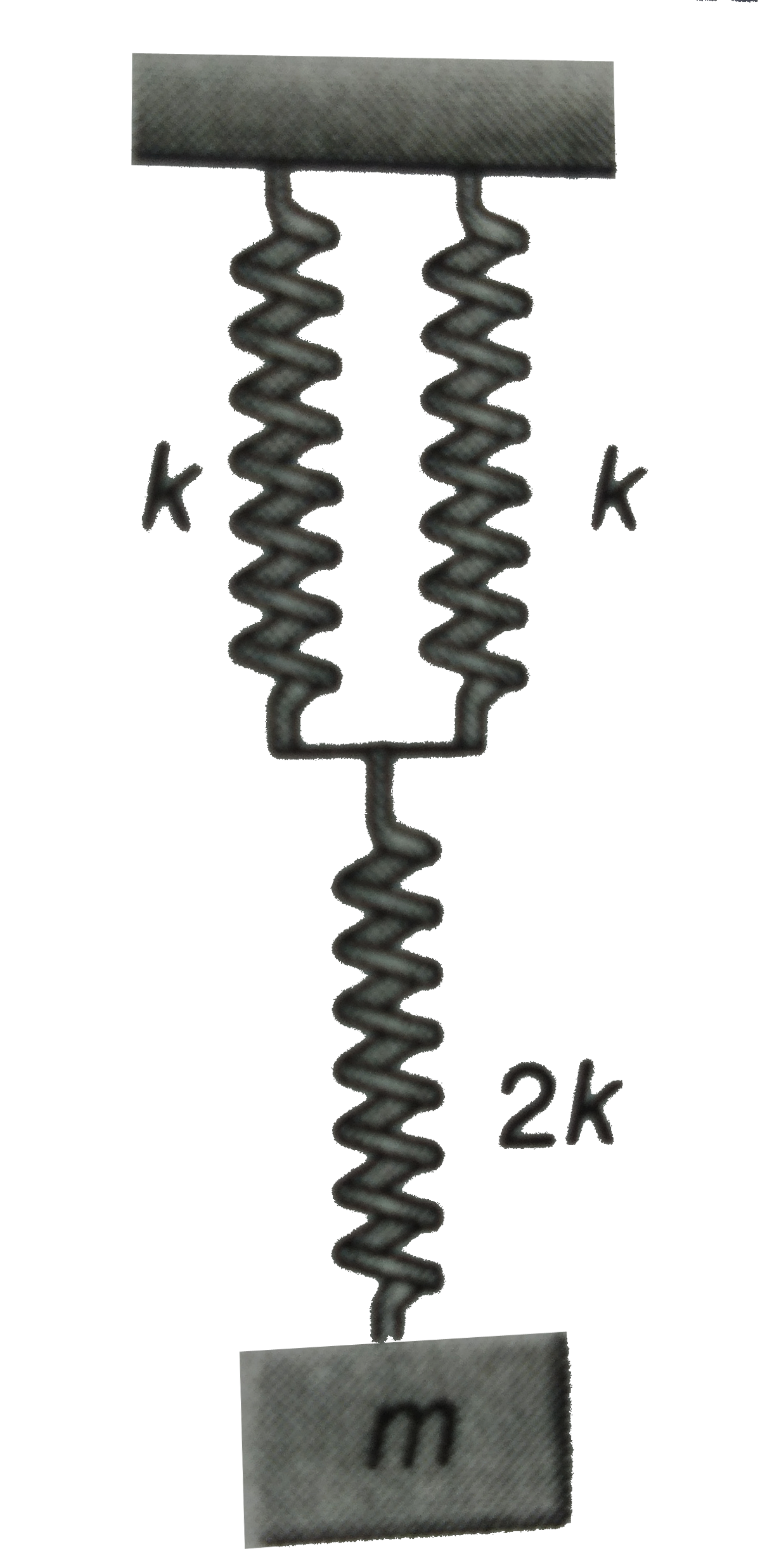 02:52View Solution
02:52View SolutionWhen an electric field is applied across a semiconductor,
02:29View SolutionIf a machine perform 4000 J output work and 1000 J is inside loss due to friction , then the find efficiency?
03:49View SolutionThe maximum velocity a particle, executing simple harmonic motion with an amplitude 7 mm, 4.4 m//s. The period of oscillation is.
03:27View SolutionA particle executes simple harmonic motion of period 16 s . Two seconds later after it passes through the center of oscillation its velocity is found to be 2 m//s . Find the amplitude.
03:08View SolutionA particle executes simple harmonic motion with frequqncy 2.5Hz and amplitude 2m. The speed of the particle 0.3s after crossing, the equilibrium position is
03:03View SolutionA particle oscillates simple harmonic motion with a period of 16 s . Two second after crossing the equilibrium position its velocity becomes 1 m//s . The amplitude is
05:03View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is πm/s. Amplitude of oscillation is
(cos45∘=1√2)02:12View SolutionThe maximum velocity of a particle executing simple harmonic motion with an amplitude 7 mm is 4.4 m/s. The period of oscillation is :
02:41View SolutionA simple harmonic oscillator has a period of 0.01 s and an amplitude of 0.2 m. The magnitude of the velocity in m/s at the centre of oscillation is……………….
01:46View Solutionएक कण 16 सेकण्ड आवर्तकाल के साथ सरल आवर्त गति कर रहा है। t=2 सेकण्ड पर कण माध्य स्थिति से गुजरता है तथा t=1 सेकण्ड पर इसका वेग 2 मी/से हो जाता है। इस गति में आयाम मीटर में है
04:56View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is pi m//s . Amplitude of oscillation is (cos 45^(@) = 1/(sqrt(2)))
03:23View SolutionA particle executes simple harmonic motion of period 16 s . Two seconds later after it passes through the center of oscillation its velocity is found to be 2 m//s . Find the amplitude.
01:22View Solution

