A body performs simple harmonic oscillations along the straight line ABCDE with C as the midpoint of AE. Its kinetic energies at B and D are each one fourth of its maximum value. If AE=2R, the distance between B and D is

√32R
R√2
√3R
√2R
The correct Answer is:C
12k(A2−x2)=14(12kA2)
∴x=√32A
∴CD=CB=√32R
or BD=2(CD)
or 2(CB)=√3R
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct8videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Comprehension2videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Subjective39videos
SIMPLE HARMONIC MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct3videos
SEMICONDUCTORS AND ELECTRONIC DEVICES
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)38videos
SOLVD PAPERS 2017 NEET, AIIMS & JIPMER
Similar Questions
Four ships A,B,C and D are at sea in the following relative positions: B is on the straight line segment AC, B is due north of D and D is due west of C. The distance B and D is 2km. ∠BDA=40∘,∠BCD=25∘. What is the distance between A and D. (sin25∘=0.423).
View SolutionA point O is taken inside a rhombus ABCD such that its distances from the vertices B and D are equal. Show that AOC is a straight line.
View SolutionA body A mass m1=1kg and body B of mass m2=4.1kg. The body A perform free vertical harmonic oscillations with the amplitude 1.6cm and frequency 25Hz. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
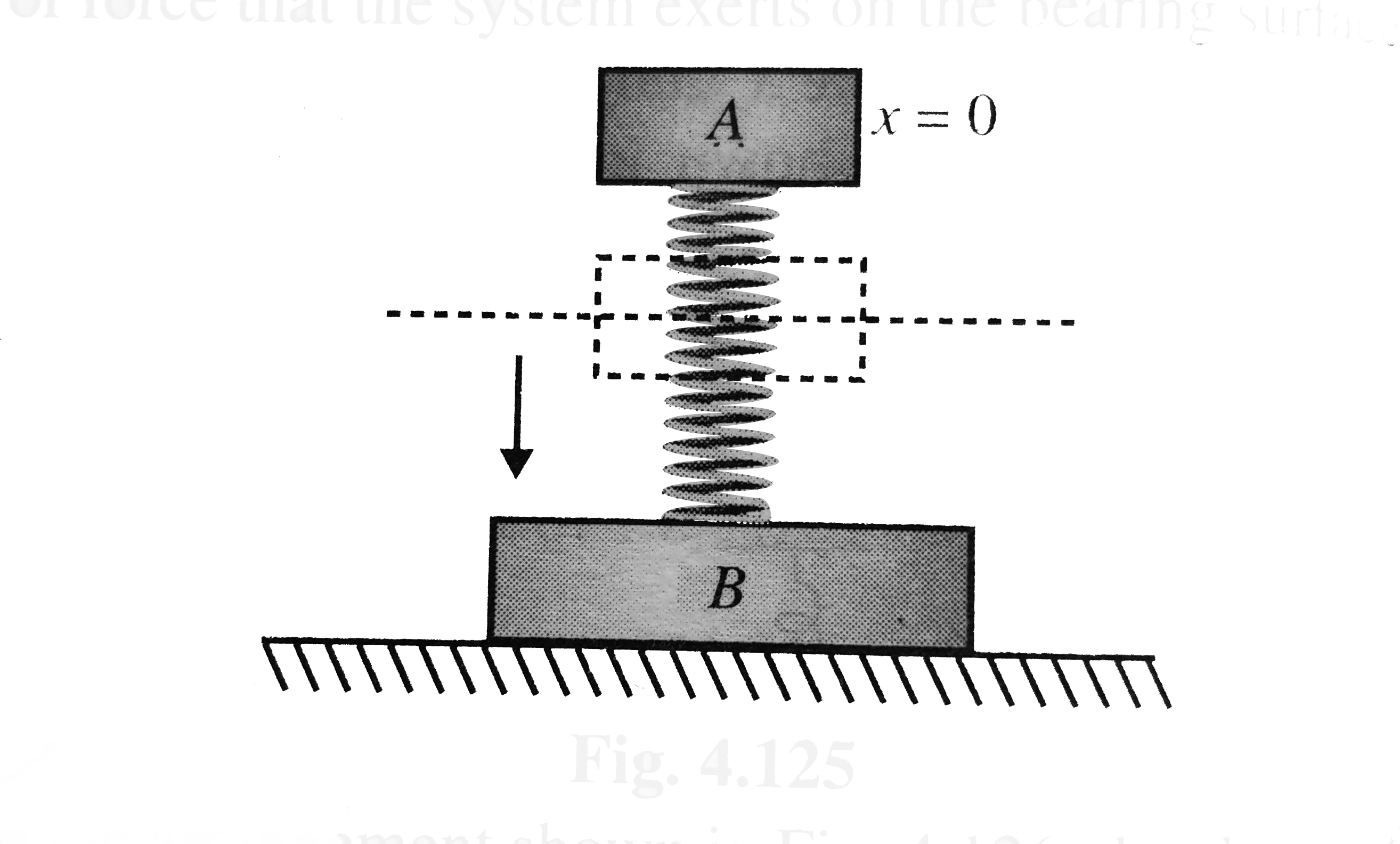 View Solution
View SolutionIn the shown arrangement, both the spring are in their natural lengths. The coefficient of friction between m2 and m1 is μ. There is no friction between m1 and the surface. If the blocks are displaced slightly, they together perform simple harmonic motion. Obtain
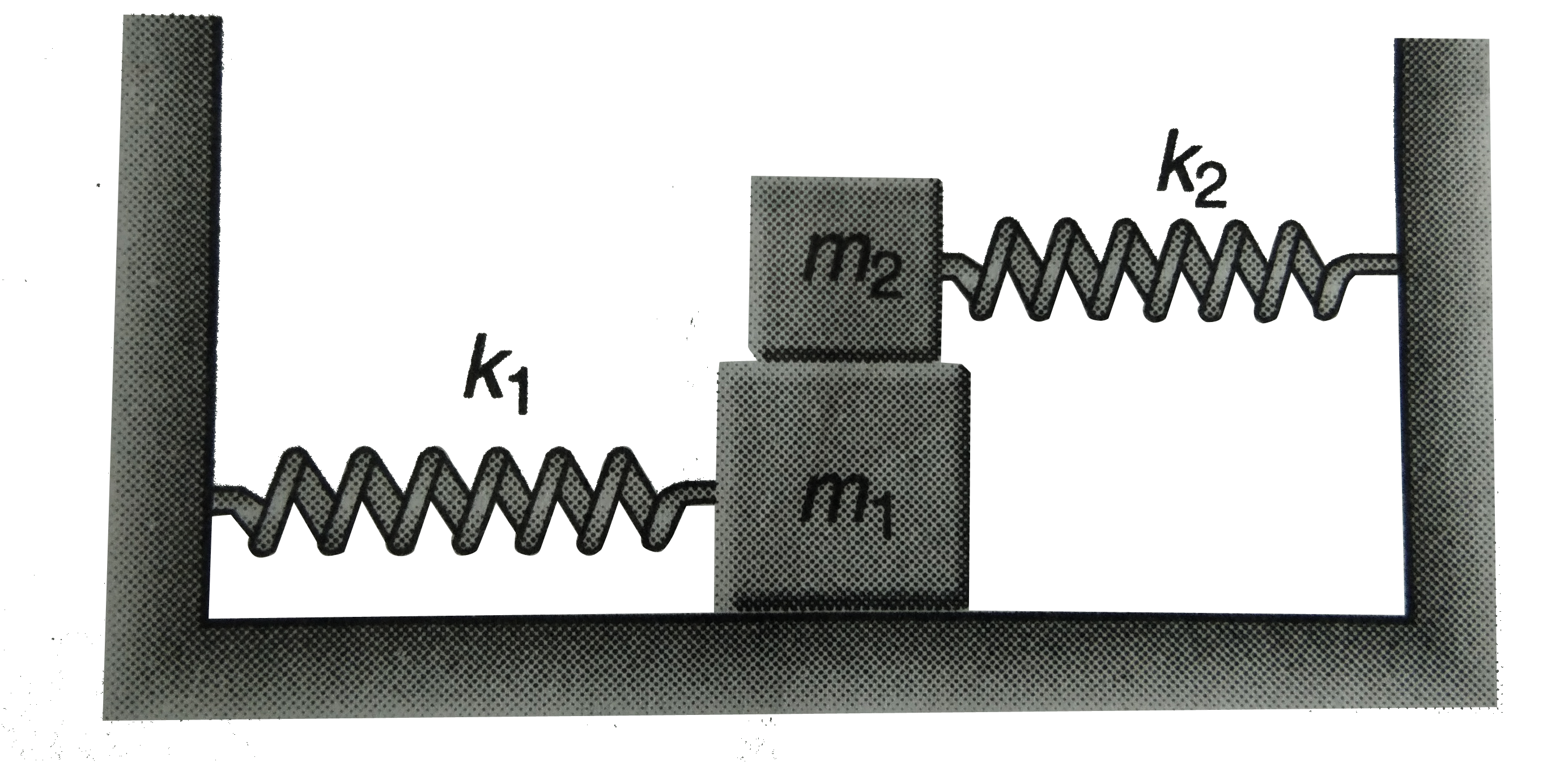
(a) Frequency of such oscillations.
(b) The condition if the friction force on clock m2 is to act in the direction of its displacement from mean position.
( c) If the condition obtained in (b) is met, what can be maximum of their oscillations ?View SolutionA body moves along a straight line OAB simple harmonically. It has zero velocity at the points A and B which are at distances 'a' and b'respectivelyom0' and has velocity 'V' when halfway between them then the period of SHM
View SolutionA particle performs SHM of amplitude A along a straight line .When it is at a distance of √32A from mean position its kinetic energy gets increased by an amount of 12mω2A2 due to an impulsive force. Then its new amplitude becomes
View SolutionA particle moves along a horizontal circle with constant speed. If 'a' is its acceleration and 'E' is its kinetic energy
A) a is constant B) E is constant C) a is variable D) E is variableView SolutionA particle moves along a horizontal circle witrr constant speed. If 'a' is its acceleration and 'E' is its kinetic energy
A) a is constant
B) E is constant
C) a is variable
D) E is variableView SolutionA particle performs SHM of time period T , along a straight line. Find the minimum time interval to go from position A to position B. At A both potential energy and kinetic energy are same and at B the speed is half of the maximum speed.
View SolutionA particle performs SHM of time period T , along a straight line. Find the minimum time interval to go from position A to position B. At A both potential energy and kinetic energy are same and at B the speed is half of the maximum speed.
View Solution

