A block of mass m, when attached to a uniform ideal apring with force constant k and free length L executes SHM. The spring is then cut in two pieces, one with free length n L and other with free length (1−n)L. The block is also divided in the same fraction. The smaller part of the block attached to longer part of the spring executes SHM with frequency f1 . The bigger part of the block attached to smaller part of the spring executes SHM with frequency f2. The ratio f1/f2 is
1
n1−n
1+nn
n1+n
The correct Answer is:A
To solve the problem, we need to analyze the situation step by step, focusing on the properties of the spring and the masses involved in the Simple Harmonic Motion (SHM).
1. Understanding the Spring Constant:
- The spring constant k is inversely proportional to the length of the spring. If the original spring has a length L and spring constant k, when the spring is cut into two parts, the spring constants of the new springs can be calculated.
- For the longer part of the spring with length nL, the spring constant k1 is given by:
k1=kn
- For the shorter part of the spring with length (1−n)L, the spring constant k2 is given by:
k2=k1−n
2. Dividing the Mass:
- The mass m is also divided in the same ratio. The smaller part of the mass m1 (attached to the longer spring) is:
m1=(1−n)m
- The bigger part of the mass m2 (attached to the shorter spring) is:
m2=nm
3. Calculating Frequencies:
- The frequency of SHM is given by the formula:
f=12π√km
- For the smaller mass m1 attached to the longer spring (with spring constant k1):
f1=12π√k1m1=12π
⎷kn(1−n)m=12π√kn(1−n)m
- For the bigger mass m2 attached to the shorter spring (with spring constant k2):
f2=12π√k2m2=12π√k1−nnm=12π√kn(1−n)m
4. Finding the Ratio of Frequencies:
- Now, we can find the ratio f1f2:
f1f2=√kn(1−n)m√kn(1−n)m=1
Final Answer:
The ratio f1f2 is 1.
- The spring constant k is inversely proportional to the length of the spring. If the original spring has a length L and spring constant k, when the spring is cut into two parts, the spring constants of the new springs can be calculated.
- For the longer part of the spring with length nL, the spring constant k1 is given by:
k1=kn
- For the shorter part of the spring with length (1−n)L, the spring constant k2 is given by:
k2=k1−n
- The mass m is also divided in the same ratio. The smaller part of the mass m1 (attached to the longer spring) is:
m1=(1−n)m
- The bigger part of the mass m2 (attached to the shorter spring) is:
m2=nm
- The frequency of SHM is given by the formula:
f=12π√km
- For the smaller mass m1 attached to the longer spring (with spring constant k1):
f1=12π√k1m1=12π
⎷kn(1−n)m=12π√kn(1−n)m
- For the bigger mass m2 attached to the shorter spring (with spring constant k2):
f2=12π√k2m2=12π√k1−nnm=12π√kn(1−n)m
- Now, we can find the ratio f1f2:
f1f2=√kn(1−n)m√kn(1−n)m=1
The ratio f1f2 is 1.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Comprehension
2 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Subjective
39 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
A block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View SolutionTime period of a block with a spring is T0. Now ,the spring is cut in two parts in the ratio 2:3. Now find the time period of same block with the smaller part of the spring.
View SolutionIn a spring- mass system , the length of the spring is L, and it has a mass M attached to it and oscillates with an angular frequency ω. The spring is then cut into two parts, one (i) with relaxed length αL and the other (ii) with relaxed length (1−α) L. The force constants of the two spring A and B are
View SolutionA block of mass m is attached to a spring of force constant k whose other end is fixed to a horizontal surface. Initially the spring is in its natural length and the block is released from rest. The average force acting on the surface by the spring till the instant when the block has zero acceleration for the first time is

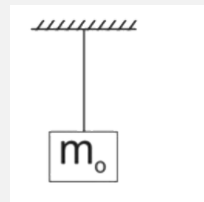
View SolutionThe block of mass m0=μL is attached to a uniform string of mass M=μL and length L as shown in the figure. If a wave pulse is produced near the block, then the time it taken to reach the ceiling is
View SolutionThe block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionA particles of mass m is fixed to one end of a light spring of force constant k and unstreatched length l. the system is rotated about the other end of the spring with an angular velocity ω in gravity free space. The increase in length of the spring is
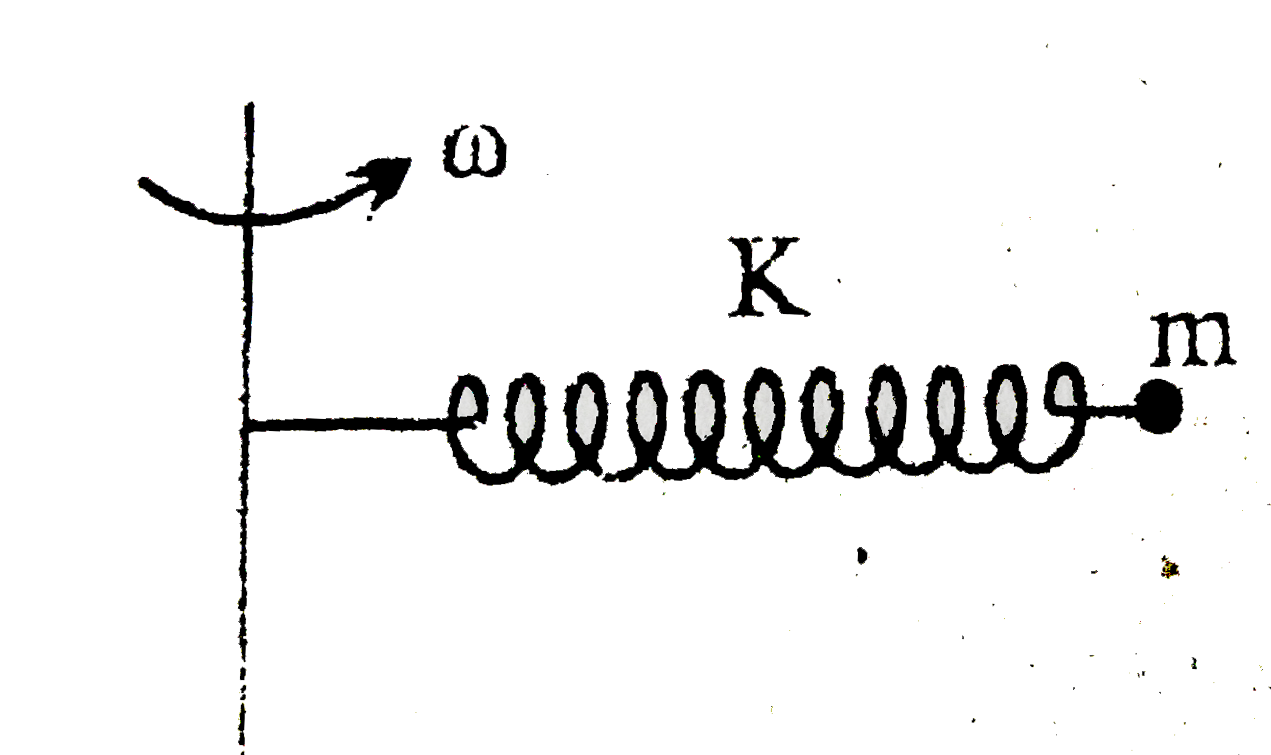
View SolutionA blocked of mass m is attached with two strings as shown in figure .Draw the free body diagram of the block.
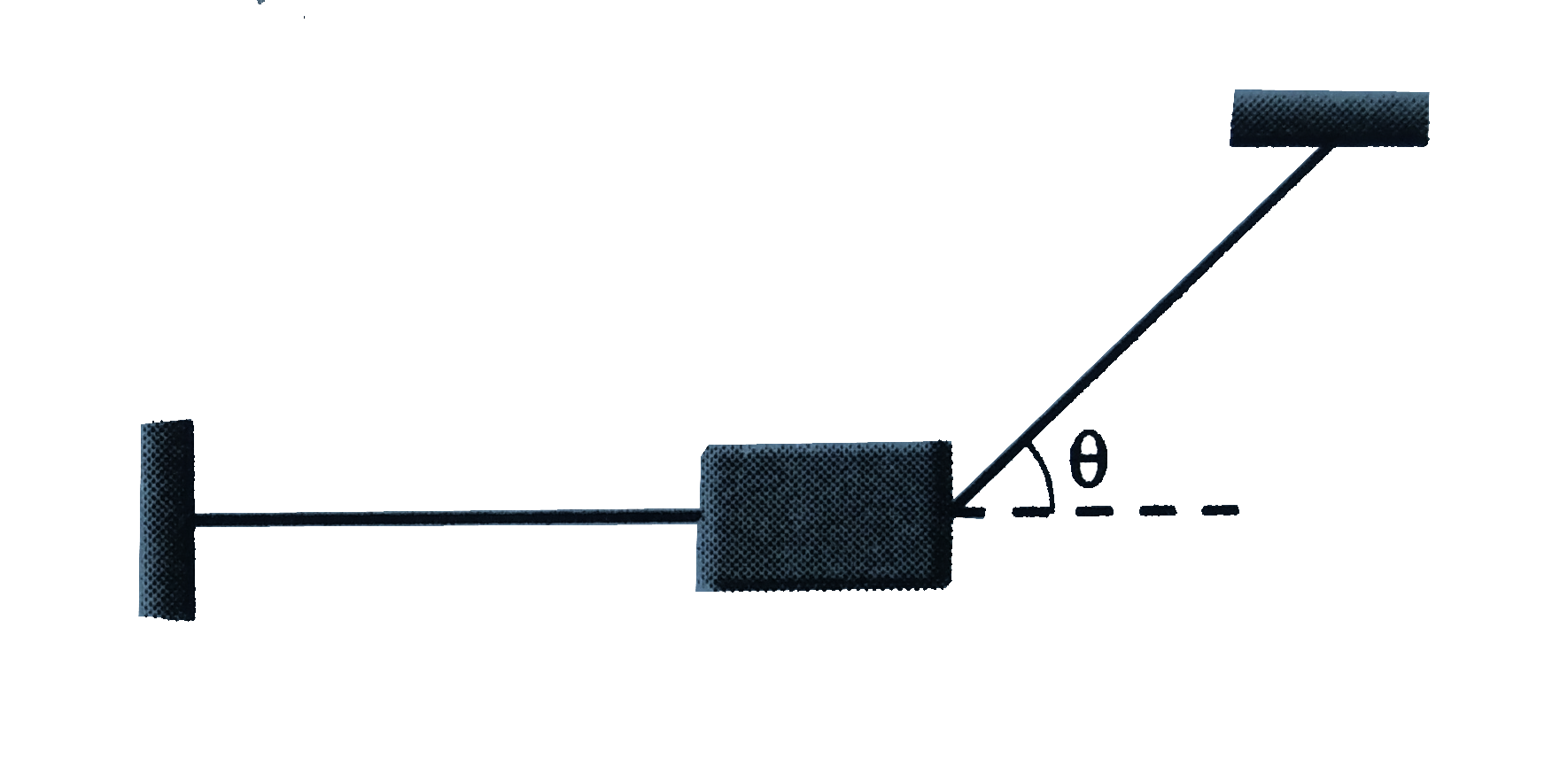
View SolutionA block of mass m is attached with two strings as shown in figure .Draw the free body diagram of the block.

View SolutionA spring of force constant k is cut into two pieces such that one piece is double the length of the other. Then the long piece will have a force constant of
View Solution
