The time taken by a particle performing SHM to pass from point A to B where its velocities are same is 2s. After another 2s it returns to B. The ratio of distance OB to its a amplitude (where O is the mean position) is
1:√2
(√2−1):1
1:2
1:2√2
The correct Answer is:A
V1=V2
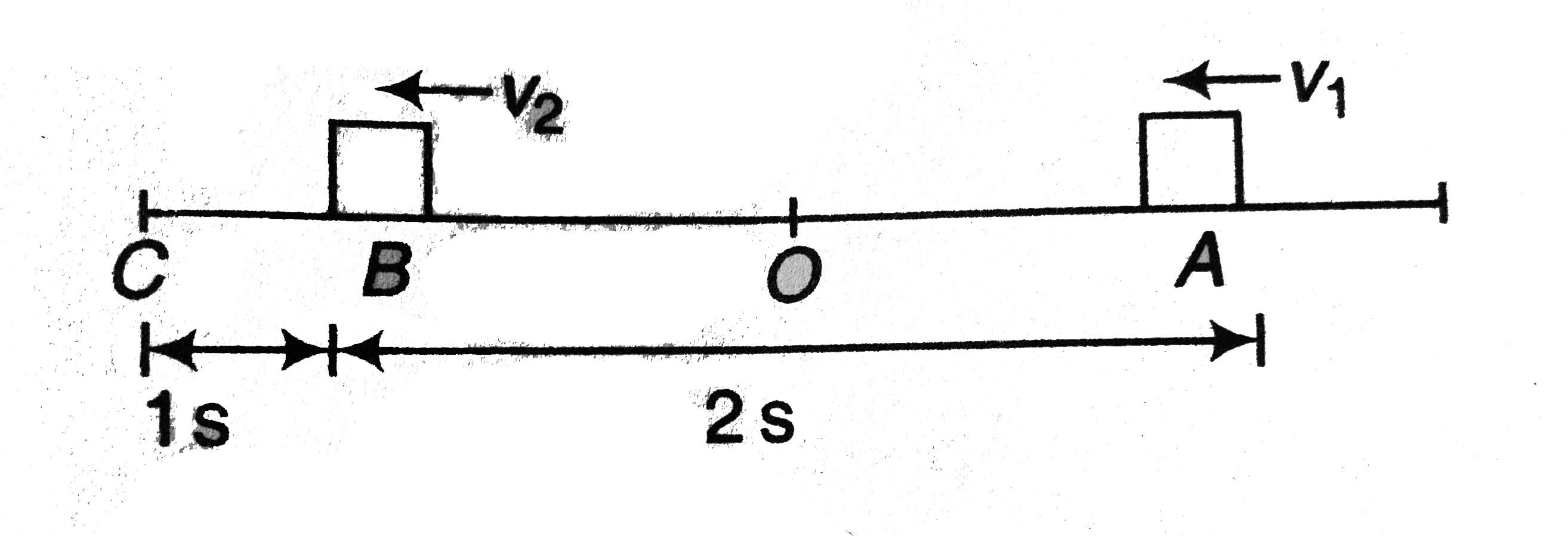
tAB=2s
∴tOB=1s
tBCB=2s
∴BC=1s
∴tOB=tBC
∴OB=A√2
or OBA=1√2.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:DC PANDEY ENGLISHChapter:SIMPLE HARMONIC MOTIONExercise:Level 2 More Than One Correct
Explore 8 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEY ENGLISHChapter:SIMPLE HARMONIC MOTIONExercise:Level 2 Comprehension
Explore 2 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEY ENGLISHChapter:SIMPLE HARMONIC MOTIONExercise:Level 1 Subjective
Explore 39 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBook:DC PANDEY ENGLISHChapter:SEMICONDUCTORS AND ELECTRONIC DEVICESExercise:More than One Option is Correct
Explore 3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBook:DC PANDEY ENGLISHChapter:SOLVD PAPERS 2017 NEET, AIIMS & JIPMERExercise:Solved paper 2018(JIPMER)
Explore 38 Videos
Similar Questions
The time taken by a particle performing SHM to pass from point A and B where it is velocities are same is 2s. After another 2 s it returns to B. The time period oscillation is (in seconds)
View SolutionThe ratio of maximum velocity to the velocity of a particle performing S.H M at a point where potential energy is 25% of total energy is
View SolutionThe particle is executing SHM on a line 4 cm long. If its velocity at mean position is 12 m/s , then determine its frequency.
View SolutionA particle executes SHM along a straight line so that its period is 12 s. The time it takes in traversing a distance equal to half its amplitude from its equilibrium position is
View SolutionA particle executes SHM along a straight line so that its period is 12 s. The time it takes in traversing a distance equal to half its amplitude from its equilibrium position is
View SolutionA particle performing SHM is found at its equilibrium position at t = 1s and it is found to have a speed 0.25 = m//s at t=2s. If the period of oscillation is 6. Calculate amplitude of oscillation.
View SolutionA partical excutes SHM with an angular frequency ω=4πrad/s. If it is at its extereme position initially, then find the transition when it is at a distance √2/2 times its amplitude from the mean position.
View SolutionThe time period of a particle executing SHM is T. After a time T/6 after it passes its mean position, then att=0 its :
View SolutionA particle performing SHM with amplitude 10cm. At What distance from mean position the kinetic energy of the particle is thrice of its potential energy ?
View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is πm/s. Amplitude of oscillation is
(cos45∘=1√2)
View Solution