Question

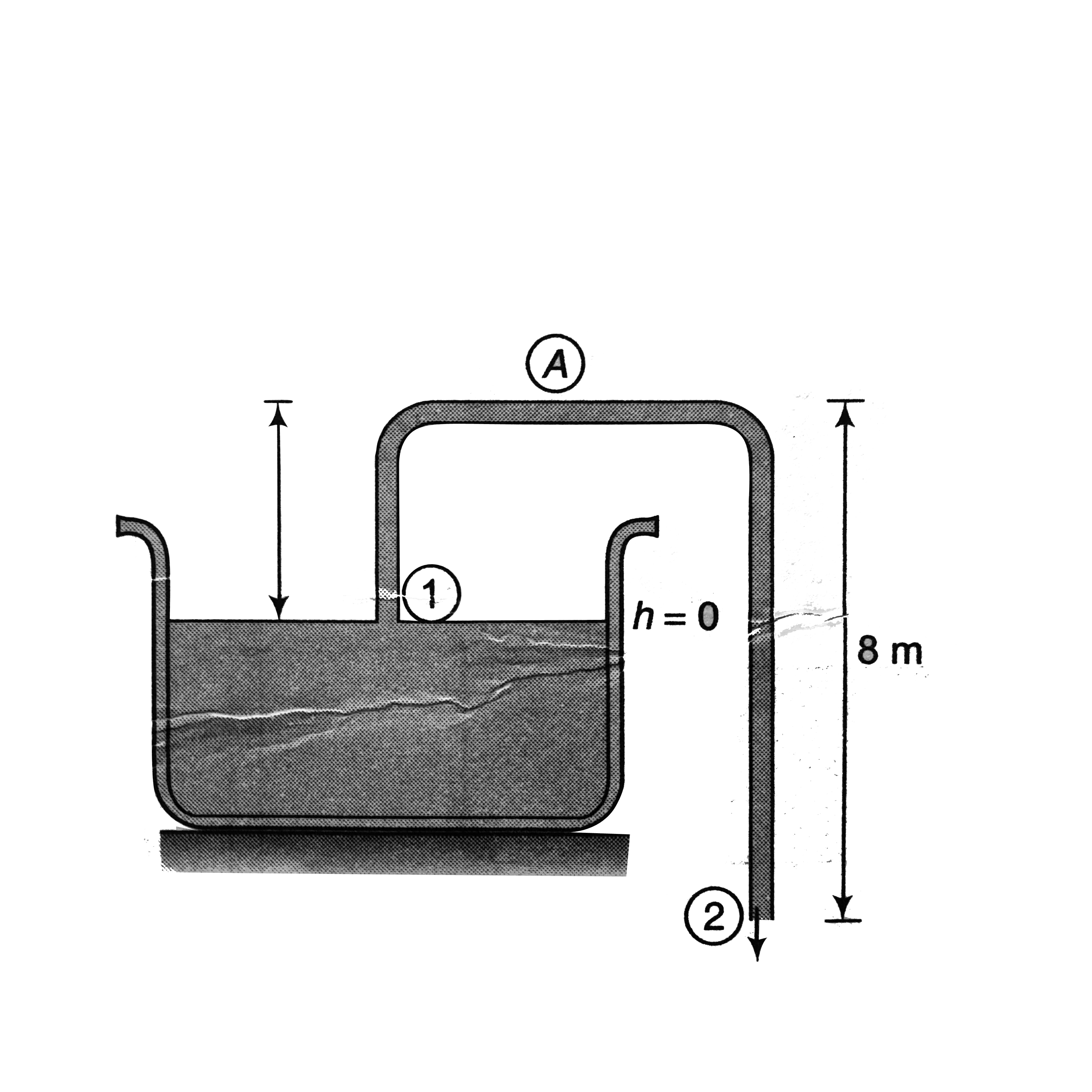
The U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure $=1.01\times {10}^{5}N/m^2,g=9.8m/s^2$ and density of water $={10}^{3}kg/m^3$
.

Answer

A::B::D

Explanation

(a) Applying Bernoulli's equation between points (1) and (2)
`P_(1)+(1)/(2) rho v_(1)^(2)+ rho gh_(1)=p_(2)+(1)/(2) rho v_(2)^(2)+ rho gh_(2)`
Since, area of reservoirgtgt area of pipe
`v_(1)~~0`.
also, `p_(1)=p_(2)=`atmospheric pressure
so, `v_(2)=sqrt(2g(h_(1)-h_(2))`
`= sqrt(2xx9.8xx7)`
`=11.7 m//s`
(b) The minimum pressure in the bend will be at A. Therefore, applying Bernoulli's equation between (1) and (A)
`p_(1)+(1)/(2) rho v_(1)^(2)+ rho gh_(1)=P_(A)+(1)/(2)rho v_(A)^(2)+rhogh_(A)`
Again, `v_(1)~~0` and from continuity equation `v_(A)-v_(2)`
or,` p_(A)=p_(1)+ rho g (h_(1)-h_(A))- (1)/(2) rho v_(2)^(2)`
Therefore, substituting the value , we have
`p_(A)=(1.01xx 10^(5))+(1000)(9.8)(-1)-(1)/(2)xx(1000)(11.7)^(2)`
`=2.27xx10^(4) N//m^(2)`.