A liquid of density ρ is filled upto a geight of 'h' in a container as shown in firgure. Base of the contaimer is a square of side L. Ignoring the atmospheric pressure find
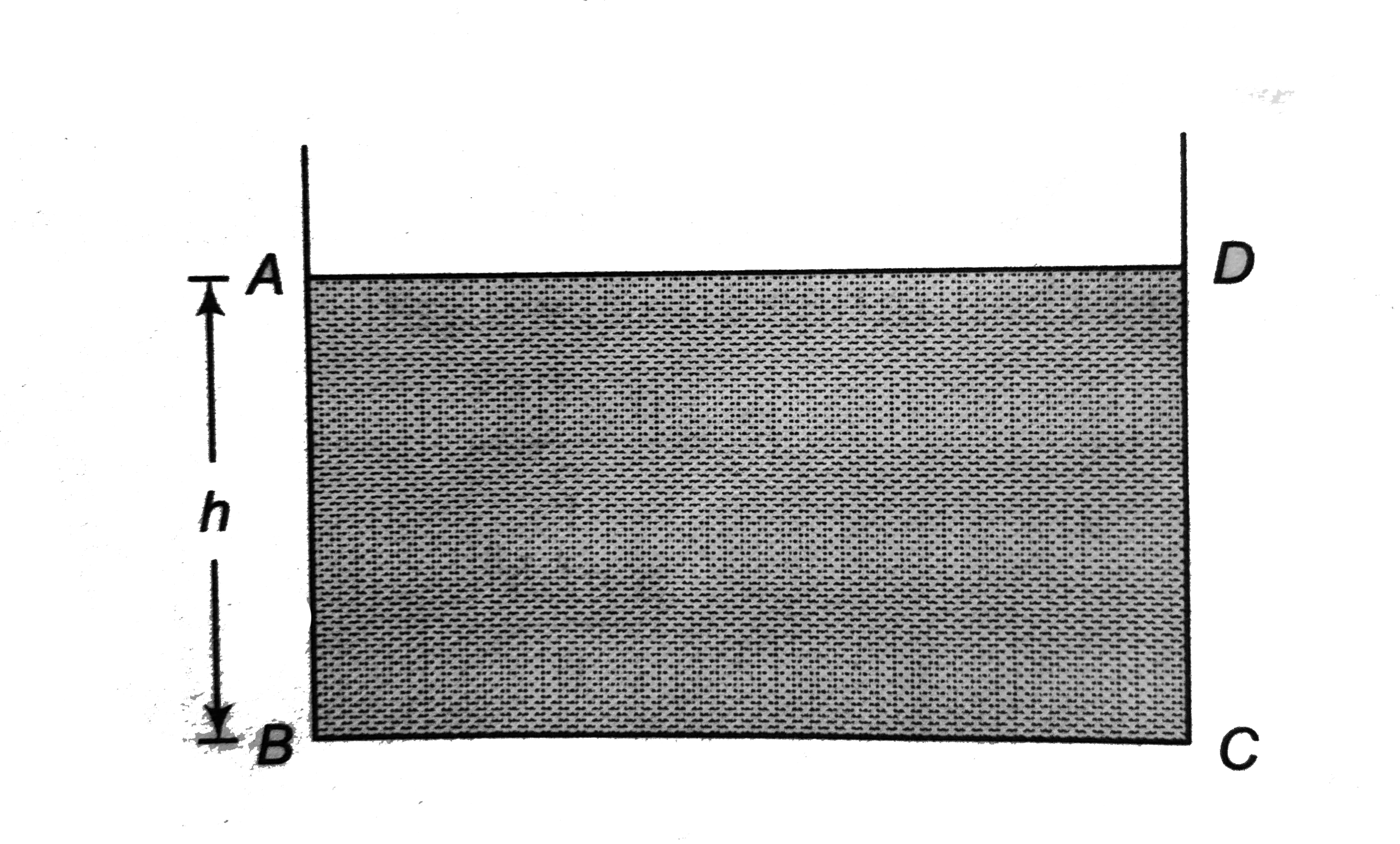
(a) pressure force F1 on its base.
(b) torque of force F1 about an axis passing through C and perpendicular to plane of paper.
(c ) Pressure force F2 on the vertical side wall DC.
(d) torque of force F2 about the same axis mentioned in part (b).
(e) point of application of force F2
The correct Answer is:A, B, C
(a) Base is horizontal. So, we can directy apply
F1=pA=(ρgh)(L)(L)
=ρghL2
(b) Point of application of F1 is at a perpendicular distance L2 from the axis mentioned in the question
∴τF1=F1×r⊥=(ρghL2)(L2)
=12ρghL3
(c) and (d) : side wall is vertical. Pressure is non-uniform. so, force and torque both will be obtained by integration.
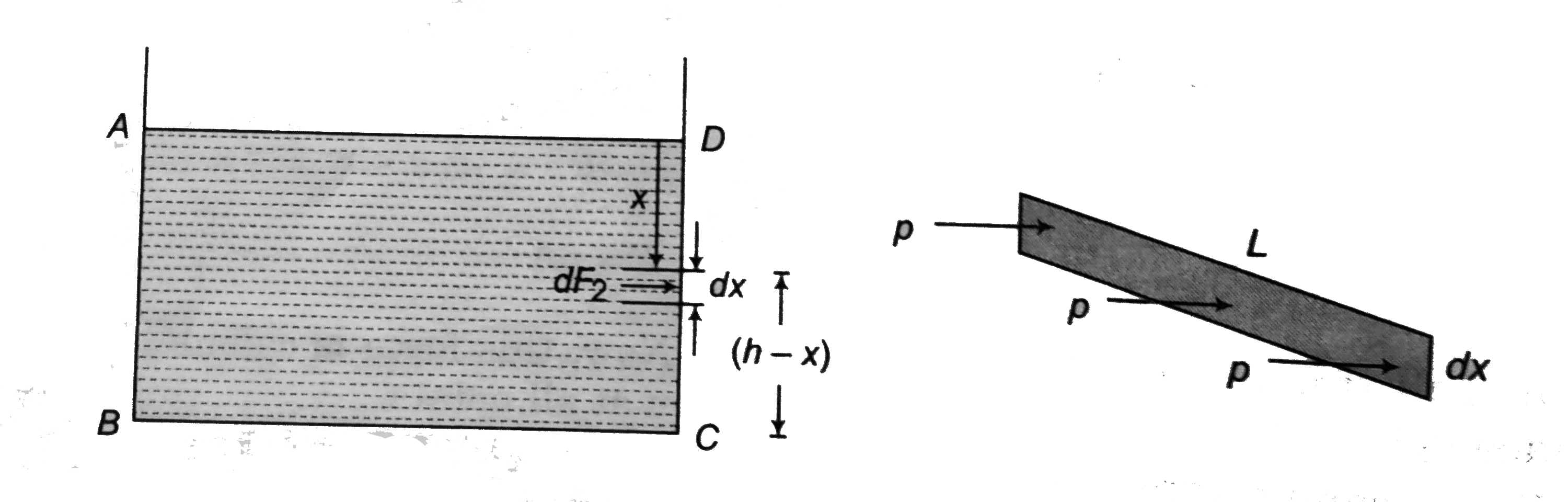
Pressure at depth x,
p=ρgx
Area of small element shown in figure is
dA=L(dx)
∴dF2=(p)(dA)=(ρgx)(Ldx)
Perpendicular distance of this small force dF2 from the axis mentioned in the question is (h−x). Therefore, small torque of force dF2 is
dτ=(dF2)(h−x)
=(ρgx)(h−x)(Ldx)
Now, F2−∫x=hx=0dF2
τF2=∫x=hx=0(dτ)
Substituting the value and then integration, we get
F2=ρgLh22
and τF2=ρgLh36
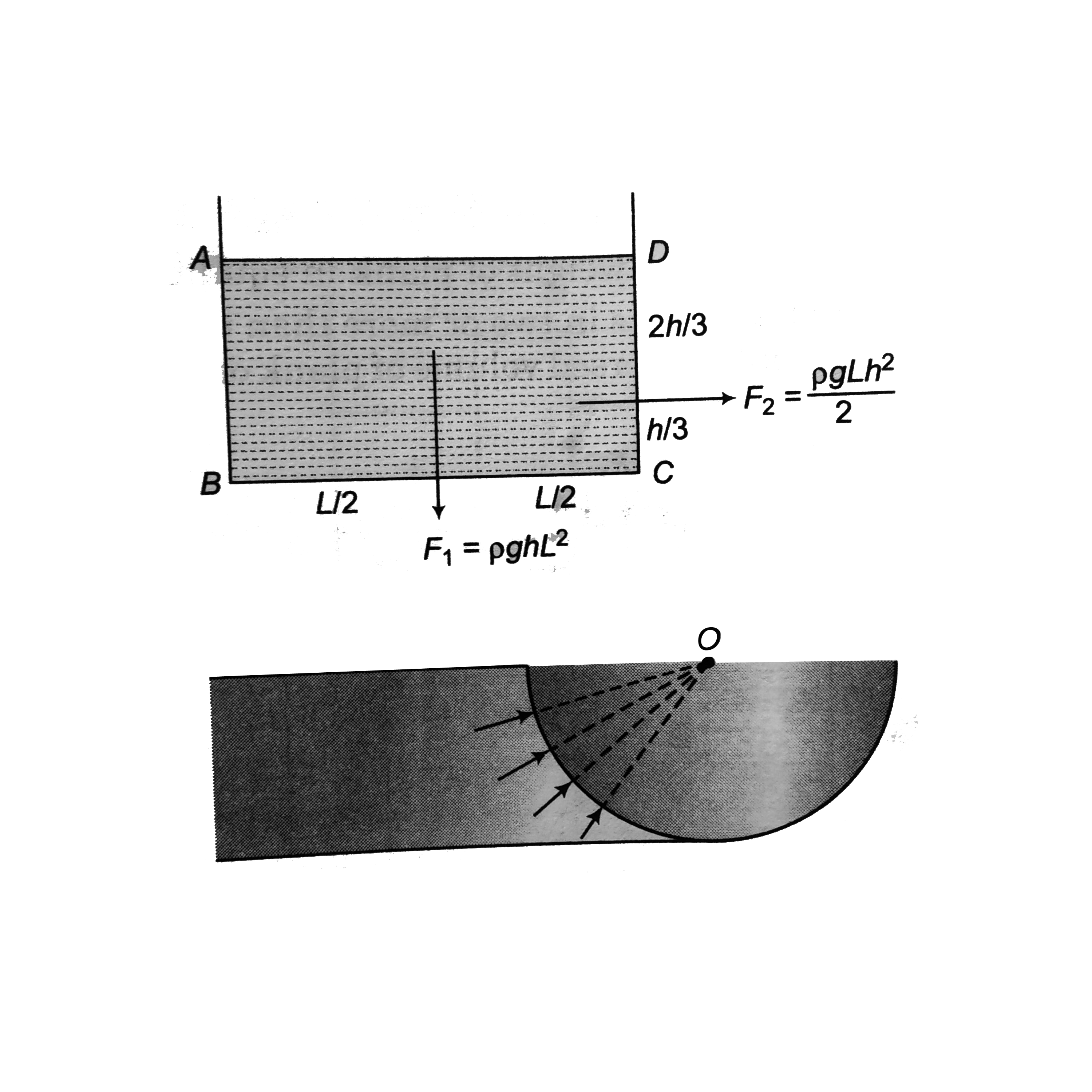
(e) For point of application of F2, we can apply
r⊥=τF2F2 (from C)
=(ρgLH3/6)(ρgH2L/2)=h3
Note that point of application of F2 is below the centre, as pressure is not uniform. It is increasing with depth.
F1 and F2 and their points of application are shown in figure below.
 .
.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Miscellaneous Examples10videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.16videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Medical entranes gallery49videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
If liquid of density ρ is filled in an open cylindrical container to height h, then pressure at bottom
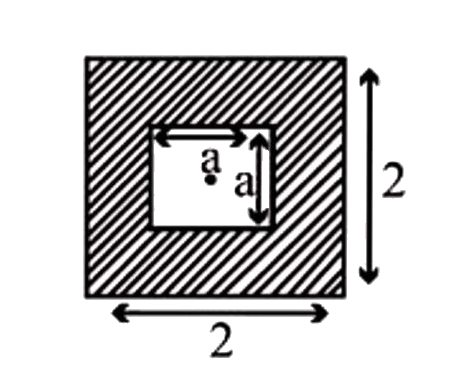
View SolutionA square of a side a is cut from a square of side 2a as shown in the figure. Mass of this square with a hole is M. Then its moment of inertia about an axis passing through its centre of mass and perpendicular to its plane will be
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depth
A beaker is filled with a liquid of density ρ upto a height h If the beaker is at rest, the mean pressure at the walls is:
View SolutionA metal sphere connected by a string is dipped in a liquid of density ρ as shown in figure. The pressure at the bottom of the vessel will be, (p0=atmospheric pressure )
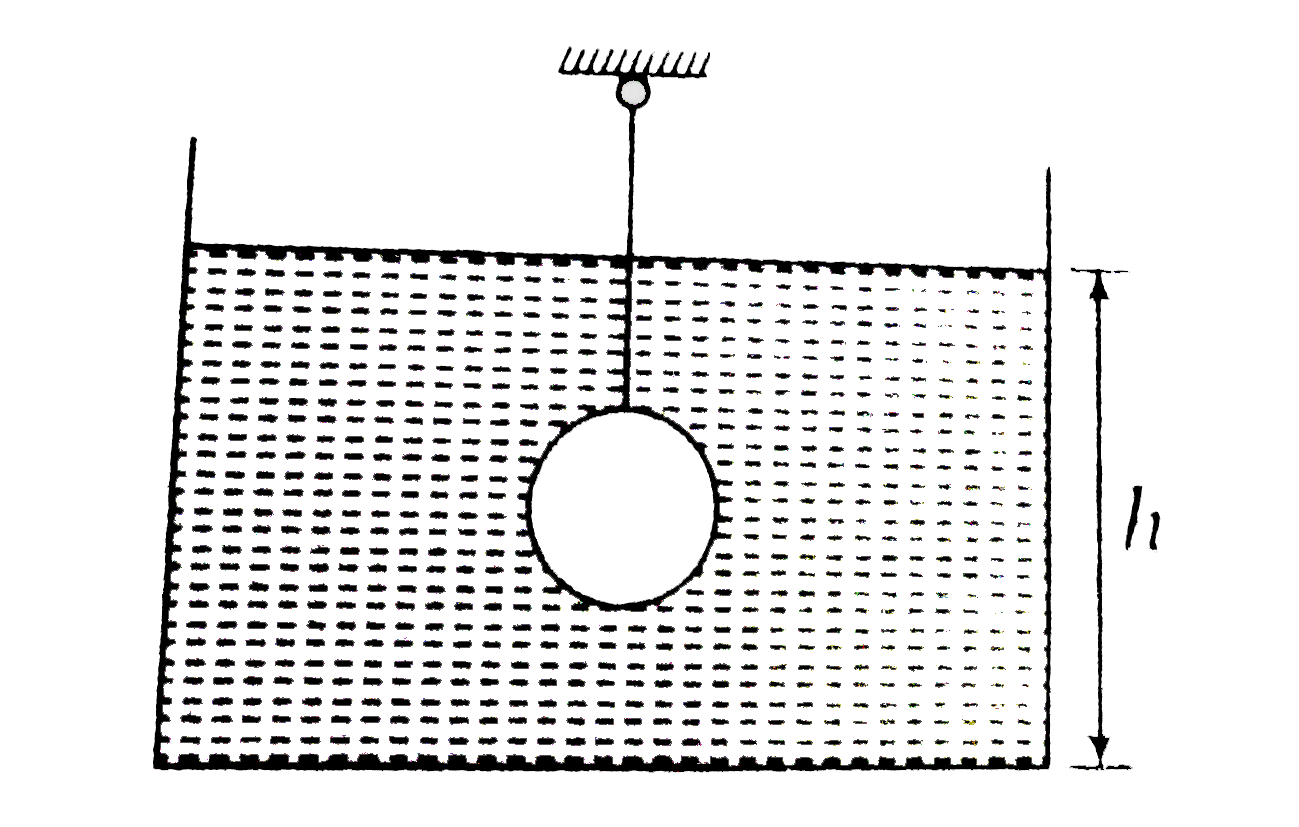 View Solution
View SolutionForces F1andF2 act on a point mass in two mutually perpendicular directions. The resultant force on the point mass will be
View SolutionForces F1andF2 act on a point mass in two mutually perpendicular directions. The resultant force on the point mass will be
View SolutionA liquid of density ρ is filled in a beaker of cross section S to a height H and then a cylinder of mass m and corss section s is made to float in it as shown in figure. It the atmospheric pressure is p0, find the pressure a at the top face A of the cylinder b at the botom face C of the cylinder and c. at the base B of the beaker. Can ever these three pressures be equal?
 View Solution
View Solution
A liquid of density ρ is rotated by an angular speed ω as shown in figure,Using the concept of pressure equation, find a relation between h1,h2,x1 and x2.View SolutionA rectangular container moves with an acceleration a along the positive direction as shown in figure. The pressure at the A in excess of the atmospheric pressure p0 is (take ρ as the density of liquid)
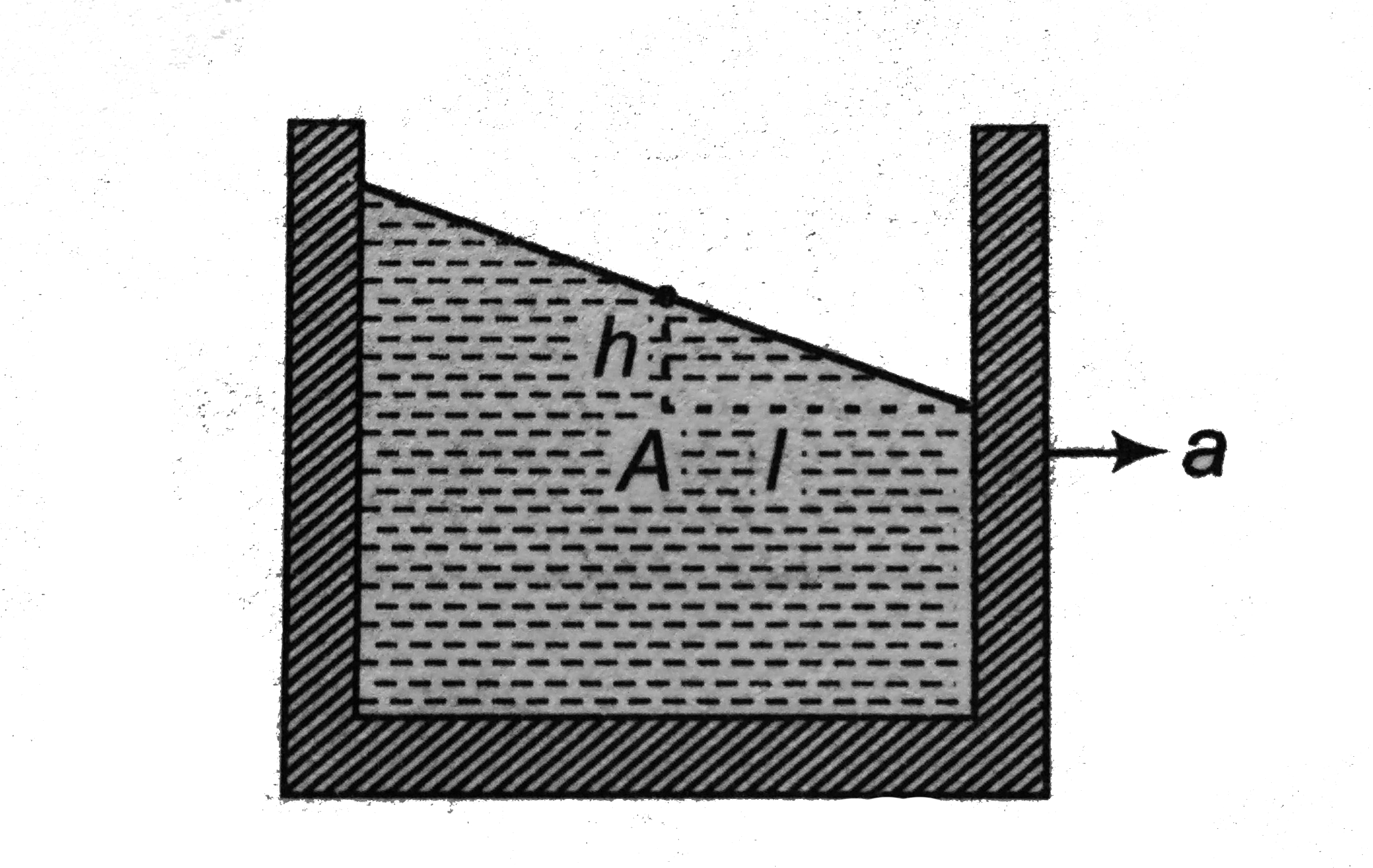 View Solution
View Solution

