What is the minimum volume of a block of wood(density if it is to hold a woman entirely above the water when she stands on it?
The correct Answer is:C
To solve the problem of determining the minimum volume of a block of wood required to hold a 50 kg woman entirely above water, we can follow these steps:
Step 1: Understand the Problem
We need to find the volume of a block of wood that can support a 50 kg woman without any part of her being submerged in water. The density of the wood is given as 850 kg/m³, and the density of water is 1000 kg/m³.
Step 2: Apply the Principle of Buoyancy
According to Archimedes' principle, the buoyant force (upthrust) acting on the block of wood must equal the total weight that the block is supporting (the weight of the woman plus the weight of the block itself).
Step 3: Calculate the Weight of the Woman
The weight of the woman can be calculated using the formula:
Weight=mass×g
Since we are working in terms of kg and kgf (kilogram-force), we can take the weight of the woman as:
Ww=50 kgf
Step 4: Calculate the Weight of the Block of Wood
The weight of the block of wood can be expressed as:
Wb=Vb×ρb
Where:
- Vb is the volume of the block of wood (which we need to find).
- ρb is the density of the wood, which is 850 kg/m³.
Step 5: Calculate the Buoyant Force
The buoyant force acting on the block of wood when it is fully submerged is given by:
Fb=Vb×ρw
Where:
- ρw is the density of water, which is 1000 kg/m³.
Step 6: Set Up the Equation
For the block to hold the woman entirely above water, the buoyant force must equal the total weight (weight of the woman plus weight of the block):
Fb=Ww+Wb
Substituting the expressions we have:
Vb×ρw=Ww+Vb×ρb
This simplifies to:
Vb×1000=50+Vb×850
Step 7: Rearranging the Equation
Rearranging the equation gives:
Vb×1000−Vb×850=50
Factoring out Vb:
Vb×(1000−850)=50
This simplifies to:
Vb×150=50
Step 8: Solve for Volume
Now, we can solve for Vb:
Vb=50150=13 m3
Thus, the minimum volume of the block of wood required is:
Vb=0.333 m3
Final Answer
The minimum volume of the block of wood required to hold a 50 kg woman entirely above water is 0.333 m³.
---
We need to find the volume of a block of wood that can support a 50 kg woman without any part of her being submerged in water. The density of the wood is given as 850 kg/m³, and the density of water is 1000 kg/m³.
According to Archimedes' principle, the buoyant force (upthrust) acting on the block of wood must equal the total weight that the block is supporting (the weight of the woman plus the weight of the block itself).
The weight of the woman can be calculated using the formula:
Weight=mass×g
Since we are working in terms of kg and kgf (kilogram-force), we can take the weight of the woman as:
Ww=50 kgf
The weight of the block of wood can be expressed as:
Wb=Vb×ρb
Where:
- Vb is the volume of the block of wood (which we need to find).
- ρb is the density of the wood, which is 850 kg/m³.
The buoyant force acting on the block of wood when it is fully submerged is given by:
Fb=Vb×ρw
Where:
- ρw is the density of water, which is 1000 kg/m³.
For the block to hold the woman entirely above water, the buoyant force must equal the total weight (weight of the woman plus weight of the block):
Fb=Ww+Wb
Substituting the expressions we have:
Vb×ρw=Ww+Vb×ρb
This simplifies to:
Vb×1000=50+Vb×850
Rearranging the equation gives:
Vb×1000−Vb×850=50
Factoring out Vb:
Vb×(1000−850)=50
This simplifies to:
Vb×150=50
Now, we can solve for Vb:
Vb=50150=13 m3
Thus, the minimum volume of the block of wood required is:
Vb=0.333 m3
The minimum volume of the block of wood required to hold a 50 kg woman entirely above water is 0.333 m³.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question2videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Single Correct36videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Single Correct49videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
Knowledge Check
- Question 1 - Select One
A vessel contains two immiscible liquids of density ρ1=1000kg/m3 and ρ2=1500kg/m3. A solid block of volume V=103m3 and density d=800kg/m3 is tied to one end of a string and the outer end is tied to the bottom of the vessel as shown in figure.
The block is immersed with two fifths of its volume in the liquid of lower density. The entire system is kept in an elevator which is moving upwards with an acceleration of a=g2. Find the tension in the string. A1. 8NB2. 6NC3. 10ND4. 12N
A1. 8NB2. 6NC3. 10ND4. 12N
A rectangular bar of soap has density 800kg/m3 floats in water density 1000kg/m3. Oil of density 300kg/m3 is slowly added, forming a layer that does not mix with water. When the top surface of thhe oil is the some level as the top surface of the soap. What is the ratio of the oil layer thickness to the soap's thickness. x/L?
 View Solution
View SolutionCalculate the molarity of water if its density is 1000kgm−3
View SolutionA hollow sphere of mass M=50kg and radius r=(340π)1/3m is immersed in a tank of water (density ρw=103kg/m3). The sphere is tied to the bottom of a tank by two wires A and B as shown. Tension in wire A is (g=10m/s2)
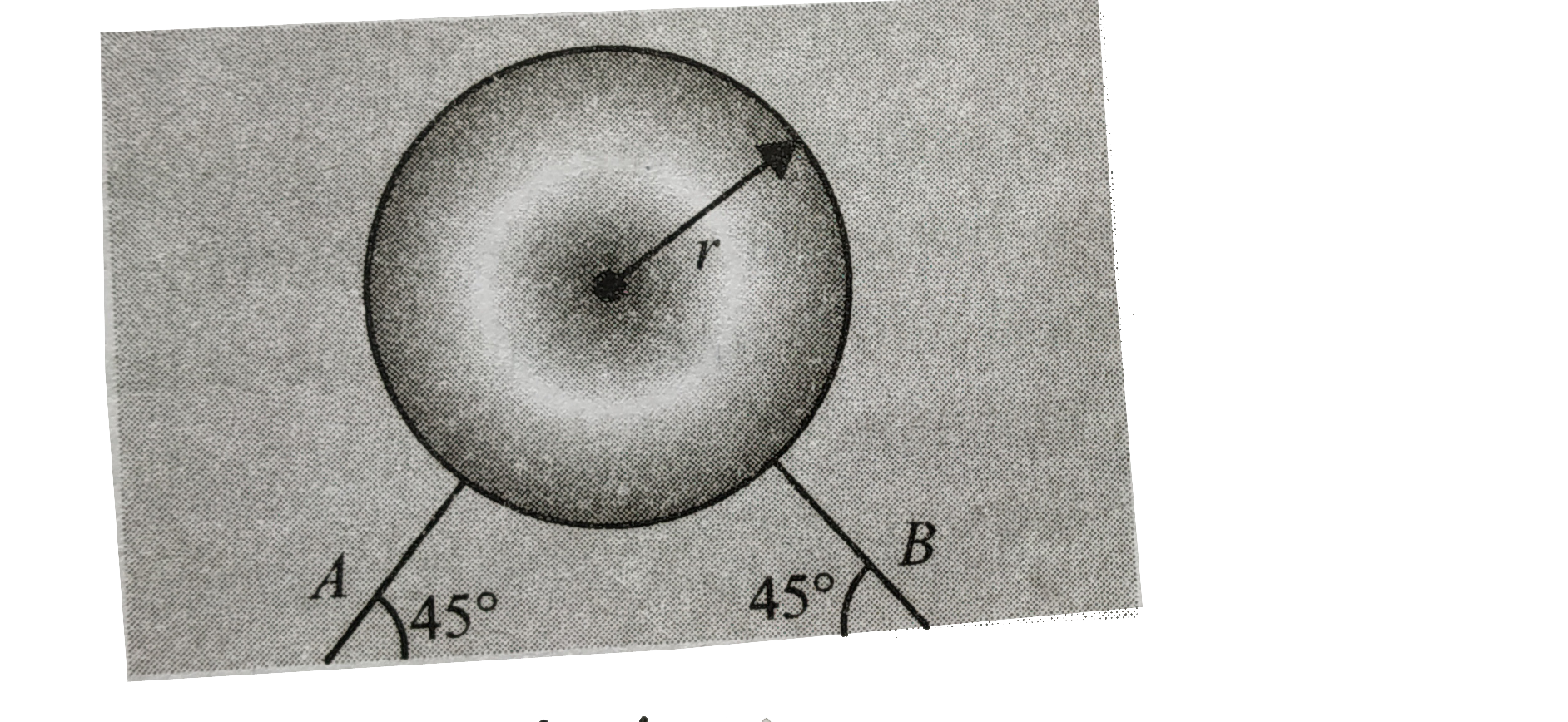 View Solution
View SolutionA block of wood floats on water with 25 th of its volume above the water surface. Calculate the density of wood.
View SolutionA small block of wood of density 0.4×103kg/m3 is submerged in water at a depth of 2.9m. Find
(a) the acceletation of the block towards the surface when the block is released and
(b) the time for the block to reach the surface, Ignore viscosity.View SolutionThe mass of wood block is 6.932 g. If density of wood is 7.7g/cm3, what is its volume ?
View SolutionThe mass of wood block is 6.932 g. If density of wood is 7.7g/cm3, what is its volume ?
View Solution

