A glass capillary sealed at the upper end is of length 0.11m and internal diameter 2×105m. The tube is immersed vertically into a liquid of surface tension 5.06×10−2N/m. To what length the capillary has to be immersed so that the liquid level inside and outside the capillary becomes the same. What will happen to water level inside the capillary if the seal is now broken?
The correct Answer is:A, B, C
To solve the problem step by step, we will break it down into two parts as stated in the question.
Part 1: Finding the length to which the capillary must be immersed
1. Understanding the Setup:
- We have a sealed glass capillary of length L=0.11m and internal diameter d=2×10−5m.
- The capillary is immersed in a liquid with surface tension T=5.06×10−2N/m.
- We need to find the length x to which the capillary must be immersed so that the liquid level inside and outside the capillary becomes the same.
2. Using Pressure Balance:
- The pressure at the bottom of the capillary (inside) must equal the atmospheric pressure (outside).
- The pressure inside the capillary can be expressed as:
Pinside=P0+2TR
- Where R is the radius of the capillary, which is half of the diameter:
R=d2=2×10−52=1×10−5m
3. Applying Boyle's Law:
- The volume of liquid inside the capillary can be expressed as Vinside=A⋅(L−x), where A is the cross-sectional area of the capillary.
- The area A can be calculated as:
A=πR2=π(1×10−5)2
- The volume outside the capillary is Voutside=A⋅L.
4. Setting Up the Equation:
- According to Boyle's Law:
P0⋅Voutside=Pinside⋅Vinside
- Substituting the expressions for pressure and volume:
P0⋅A⋅L=(P0+2TR)⋅A⋅(L−x)
- Canceling A from both sides:
P0⋅L=(P0+2TR)⋅(L−x)
5. Solving for x:
- Rearranging gives us:
P0⋅L=P0⋅L−P0⋅x+2TR⋅L−2TR⋅x
- Simplifying leads to:
P0⋅x+2TR⋅x=2TR⋅L
- Factoring out x:
x(P0+2TR)=2TR⋅L
- Finally, solving for x:
x=2TR⋅LP0+2TR
6. Substituting Values:
- Given P0=1.01×105Pa, T=5.06×10−2N/m, R=1×10−5m, and L=0.11m:
x=2×5.06×10−2×0.111×10−51.01×105+2×5.06×10−21×10−5
7. Calculating the Final Value:
- After performing the calculations, we find:
x≈0.01m or 1cm
Part 2: Effect of Breaking the Seal
1. Understanding the Effect of Breaking the Seal:
- When the seal is broken, the pressure inside the capillary becomes equal to the atmospheric pressure.
- This will allow the liquid to rise in the capillary.
2. Calculating the New Height:
- The height h to which the liquid will rise can be calculated using:
h=2TρgR
- Where ρ is the density of water (≈1000kg/m3) and g is the acceleration due to gravity (≈9.8m/s2).
3. Substituting Values:
- Substituting the known values:
h=2×5.06×10−21000×9.8×1×10−5
4. Calculating the Height:
- Performing the calculations gives:
h≈1.03m
5. Conclusion:
- Since the original length of the capillary is 0.11m and the liquid can rise 1.03m, the liquid will rise to the top of the capillary when the seal is broken.
Summary
- The capillary must be immersed to a length of 1 cm for the liquid levels to equalize.
- If the seal is broken, the liquid will rise to the top of the capillary, reaching a height of approximately 1.03 m.
- We have a sealed glass capillary of length L=0.11m and internal diameter d=2×10−5m.
- The capillary is immersed in a liquid with surface tension T=5.06×10−2N/m.
- We need to find the length x to which the capillary must be immersed so that the liquid level inside and outside the capillary becomes the same.
- The pressure at the bottom of the capillary (inside) must equal the atmospheric pressure (outside).
- The pressure inside the capillary can be expressed as:
Pinside=P0+2TR
- Where R is the radius of the capillary, which is half of the diameter:
R=d2=2×10−52=1×10−5m
- The volume of liquid inside the capillary can be expressed as Vinside=A⋅(L−x), where A is the cross-sectional area of the capillary.
- The area A can be calculated as:
A=πR2=π(1×10−5)2
- The volume outside the capillary is Voutside=A⋅L.
- According to Boyle's Law:
P0⋅Voutside=Pinside⋅Vinside
- Substituting the expressions for pressure and volume:
P0⋅A⋅L=(P0+2TR)⋅A⋅(L−x)
- Canceling A from both sides:
P0⋅L=(P0+2TR)⋅(L−x)
- Rearranging gives us:
P0⋅L=P0⋅L−P0⋅x+2TR⋅L−2TR⋅x
- Simplifying leads to:
P0⋅x+2TR⋅x=2TR⋅L
- Factoring out x:
x(P0+2TR)=2TR⋅L
- Finally, solving for x:
x=2TR⋅LP0+2TR
- Given P0=1.01×105Pa, T=5.06×10−2N/m, R=1×10−5m, and L=0.11m:
x=2×5.06×10−2×0.111×10−51.01×105+2×5.06×10−21×10−5
- After performing the calculations, we find:
x≈0.01m or 1cm
- When the seal is broken, the pressure inside the capillary becomes equal to the atmospheric pressure.
- This will allow the liquid to rise in the capillary.
- The height h to which the liquid will rise can be calculated using:
h=2TρgR
- Where ρ is the density of water (≈1000kg/m3) and g is the acceleration due to gravity (≈9.8m/s2).
- Substituting the known values:
h=2×5.06×10−21000×9.8×1×10−5
- Performing the calculations gives:
h≈1.03m
- Since the original length of the capillary is 0.11m and the liquid can rise 1.03m, the liquid will rise to the top of the capillary when the seal is broken.
- The capillary must be immersed to a length of 1 cm for the liquid levels to equalize.
- If the seal is broken, the liquid will rise to the top of the capillary, reaching a height of approximately 1.03 m.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question2videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Single Correct36videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Single Correct49videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
A glass capillary of length l and inside radius r(r<<l) is submerged vertically into water. The upper end of the capillary is scaled. The atmospheric pressure is p0. To what length h has the capillary to be submerged to make the water levels inside and outside the capillary coincide. Assume that temperature of air in the capillary remains constant. (given, surface tension of water = T, angle of contact between glass water interface =0∘)
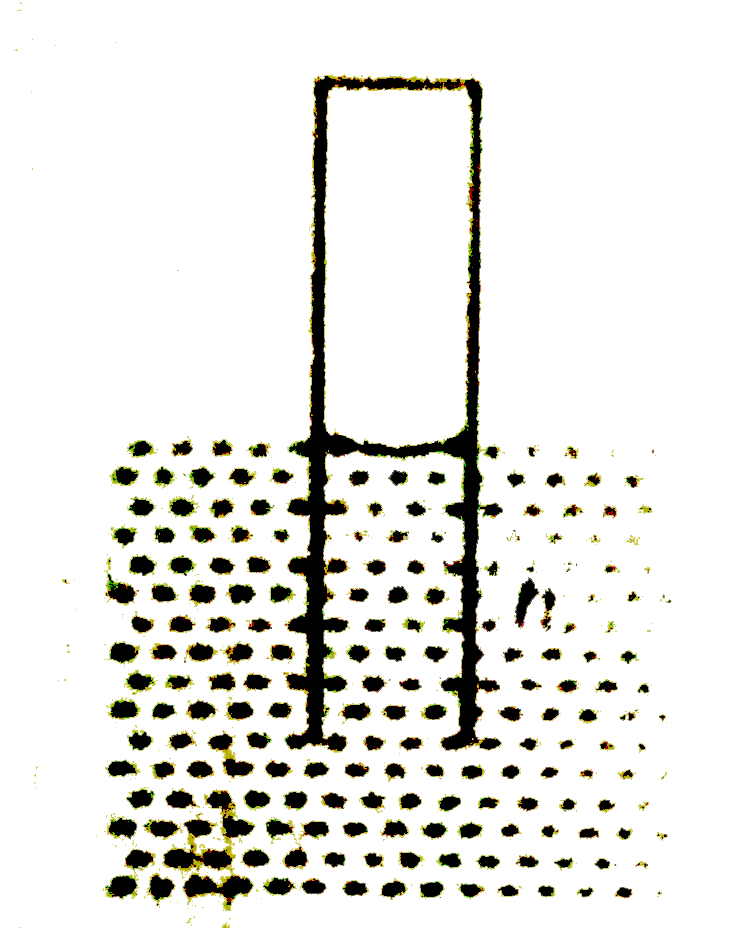 View Solution
View SolutionA capillary tube of inside diameter 1 mm is dipped vertically in a liquid of surface tension 63×10−3N/m and density 1262kgm3. Find the height of the capillary rise if the angle of contact is 10∘.
View Solution
Knowledge Check
- Question 1 - Select One
If the surface tension of water is 0.06 N/m, then the capillary rise in a tube of diameter 1mm is (θ=0∘)
A1.22 cmB2.44 cmC3.12 cmD3.86 cm
A capillary tube of length 12 cm and inside diameter 0.033m is dipped vertically into water. The top end of the capillary is sealed. If the outside pressure is 1.01×105N/m2. Find the length to which the capillary tube should be submerged in water so that the levels inside the outside coincide. σ=72×10−3N/m.
View SolutionA spherical drop of water has 1mm radius. If the surface tension of water is 70×10−3N/m, then the difference of pressure between inside and outside of the spherical drop is:
View SolutionRadius of a capillary is 2×10−3m. A liquid of weight 6.28×10−4N may remain in the capillary, then the surface tension of liquid will be:
View SolutionA spherical drop of water has 1 mm radiusHf the surface tension of water is 75×10−3 N/m, then difference of pressure between inside and outside of the drop is
View SolutionA long capillary tube of radius 0.2mm is placed vertically inside a beaker of water.
If the surface tension of water is 7.2×10−2N/m the angle of contact between glass and water is zero, then determine the height of the water column in the tube.View SolutionIf a 5cm long capillary tube with 0.1mm internal diameter, open at both ends, is slightly dipped in water having surface tension 75dyn/cm, state whether:
(i) water will rise halfway in the capillary,
(ii) water will rise up to the upper end of capillary,
(iii) water will overflow out of the upper end of capillary.
Explain your answer.View SolutionA glass capillary tube of inner diameter 0.28 mm is lowered vertically into water in a vessel. The pressure to be applied on the water in the capillary tube so that water level in the tube is same as the vessel in Nm2 is (surface tension of water =0.07Nm atmospheric pressure =105Nm2
View Solution

